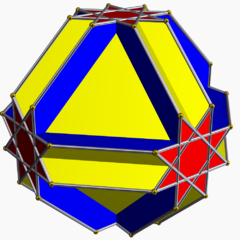
立方截角立方八面體
| ||||||||||||||||||||||||||||||||||||||||||||||||||
Read other articles:

U.S. Highway in Michigan This article is about the section of highway in Michigan. For the entire length of highway, see U.S. Route 10. For the state trunkline of the same number, see M-10 (Michigan highway). US Highway 10US 10 highlighted in red, the ferry connection in blueRoute informationMaintained by MDOTLength139.656 mi[1] (224.755 km)ExistedNovember 11, 1926[2]–presentTouristroutes Lake Michigan Circle Tour and Spur West Michigan Pike Pure Michigan...

Thomas Cup 1949 Généralités Sport Badminton Organisateur(s) IBF Édition 1re Lieu(x) Glasgow Preston Date février 1949 Nations 10 (qualifications)3 (tournoi final) Épreuves 1 Palmarès Vainqueur Fédération de Malaisie (1er titre) Finaliste Danemark Navigation Singapour 1952 modifier Article principal : Thomas Cup. La 1re édition du championnat du monde de badminton par équipes masculines, appelé également Thomas Cup, a eu lieu en février 1949 en Grande-Bretagne. Format d...

National anthem of Afghanistan from 1978 to 1992 Garam shah lā garam shahEnglish: Be Ardent, Be More Ardentگرم شه، لا گرم شهFormer national anthem of AfghanistanLyricsSulaiman LaiqMusicJalīl GhahlāndAdopted1978Relinquished1992Preceded byNational anthem of the Republic of Afghanistan”Succeeded byFortress of Islam, Heart of Asia National anthems of Afghanistan Royal Salute 1926–1943 Grand Salute 1943–1973 So long as there is Earth and Heaven 1973

赫尔曼·施陶丁格出生(1881-03-23)1881年3月23日德意志帝国黑森-达姆施塔特沃尔姆斯逝世1965年9月8日(1965歲—09—08)(84歲)德国弗赖堡国籍德国母校哈勒大学知名于聚合物化学奖项诺贝尔化学奖科学生涯研究领域有机化学、聚合物化学机构斯特拉斯堡大学卡尔斯鲁厄理工学院苏黎世联邦理工学院弗赖堡大学博士導師丹尼尔·福尔兰德尔博士生拉沃斯拉夫·鲁日奇卡 赫尔曼·施陶丁...

Палац і парк Багатель в Булонському лісі Парк Багатель (фр. Parc de Bagatelle) знаходиться на території Булонського лісу в Парижі. Парк Багатель є частиною паризького Ботанічного саду, разом з Отейським оранжерейним садом в тому ж таки Булонському лісі та двома іншими парками у Ве

Ensemble Stars!Promosi untuk Permainanあんさんぶるスターズ!(Ansanburu Sutāzu!)GenreSchool life[1] Musik PermainanPengembangCacalia StudioPenerbitHappy Elements K.K.Genre Life simulation Rhythm game PlatformiOSAndroidRilisJP: 28 April 2015 (Android)1 Mei 2015 (iOS) MangaPengarangSayo IchiPenerbitKodanshaMajalahAriaDemografiShōjoTerbit28 Agustus 2015 – 28 Desember 2016Volume4 Seri animeSutradaraMasakazu Hishida (菱田正和)ProduserYuka Aoto (青戸友香) (Bandai Namco...

Czechs in SerbiaЧеси у СрбијиČesi v Srbsku Coat of arms of the National Council of the Czech National Minority in SerbiaTotal population1,824 (2011)LanguagesSerbian and CzechReligionRoman Catholicism, ProtestantismRelated ethnic groupsSlovaks, other West Slavs According to the 2011 census, Czechs (Serbian: Чеси / Česi) in Serbia number 1,824 of population.[1] National Council of the Czech National Minority in Serbia have seat in Bela Crkva in Vojvodina. Demographics P...

American actress and director Margaret WebsterScene from Margaret Webster's Broadway production of Othello (1943) with Uta Hagen as Desdemona, Webster as Emilia, and Jack Manning as RoderigoBorn(1905-03-15)March 15, 1905New York City, USDiedNovember 13, 1972(1972-11-13) (aged 67)Sydenham, London, EnglandOccupations Actress director producer ParentsBen Webster (father)Dame May Whitty (mother)RelativesBenjamin Nottingham Webster (great-grandfather) Margaret Webster (March 15, 1905 – ...

Esbjerg Power StationEsbjerg Power Station with chimneyLocation of Esbjerg Power Station in DenmarkCountryDenmarkLocationEsbjergCoordinates55°27′16.5″N 8°27′19.1″E / 55.454583°N 8.455306°E / 55.454583; 8.455306StatusOperationalCommission date1992Owner(s)ØrstedOperator(s)ØrstedThermal power stationPrimary fuelCoalPower generationNameplate capacity378 MWAnnual net output2,405 GWh (electricity 2006)2,559 ...

Quetzaltenango Gemeente in Guatemala Situering Departement Quetzaltenango Coördinaten 14° 50′ NB, 91° 31′ WL Algemeen Oppervlakte 127 km² Inwoners (2017) 164.684[1] (1296 inw./km²) Hoogte 2333 m Foto's Quetzaltenango Portaal Noord-Amerika Quetzaltenango is een stad en gemeente in Guatemala en is de hoofdstad van het gelijknamige departement. De stad is gelegen in een dal op een hoogte van 2333 meter boven zeeniveau en wordt omgeven door vulkanen; de gemee...

Turkish football manager This biography of a living person needs additional citations for verification. Please help by adding reliable sources. Contentious material about living persons that is unsourced or poorly sourced must be removed immediately from the article and its talk page, especially if potentially libelous.Find sources: Hikmet Karaman – news · newspapers · books · scholar · JSTOR (January 2018) (Learn how and when to remove this template m...

Japanese-American wrestler and restaurateur (1938–2008) Rocky Aoki青木 廣彰Aoki, c. 1981BornHiroaki Aoki(1938-10-09)October 9, 1938Tokyo, JapanDiedJuly 10, 2008(2008-07-10) (aged 69)New York City, New York, U.S.Resting placeKuhombutsu Joshin-ji, Setagaya ward, Tokyo, JapanAlma materKeio University (no degree)New York City Community College (1963, A.A. in Management)OccupationsRestauranterPowerboat RacerKnown forFounding Benihana, Double Eagle VSpouses Chizuru Kobayashi Ao...

Egyptian musician and composer This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Mohamed El Qasabgi – news · newspapers · books · scholar · JSTOR (August 2015) (Learn how and when to remove this template message) Mohamed El Qasabgi Mohamed el-Qasabgi (Arabic: محمد القصبجي; pronounced in local Egypti...

「アイルランド国防軍」とは異なります。 この記事は検証可能な参考文献や出典が全く示されていないか、不十分です。出典を追加して記事の信頼性向上にご協力ください。(このテンプレートの使い方)出典検索?: アイルランド共和軍 – ニュース · 書籍 · スカラー · CiNii · J-STAGE · NDL · dlib.jp · ジャパンサーチ · TWL(2017�...

大竹 耕太郎阪神タイガース #49 2023年4月8日 阪神甲子園球場基本情報国籍 日本出身地 熊本県熊本市南区生年月日 (1995-06-29) 1995年6月29日(28歳)身長体重 184 cm87 kg選手情報投球・打席 左投左打ポジション 投手プロ入り 2017年 育成選手ドラフト4位初出場 2018年8月1日年俸 6700万円(2024年)[1]経歴(括弧内はプロチーム在籍年度) 熊本県立済々黌高等学校 早稲田大�...

Lady Gaga: Queen of Pop Sampul buku, edisi InggrisPengarangEmily HerbertNegaraInggrisBahasaInggrisSubjekLady GagaGenreBiografiPenerbitJohn Blake Publishing Ltd.Tanggal terbit2010Halaman288ISBNISBN 1844549631OCLC515459835Didahului olehStephen Gately and Boyzone – Blood Brothers 1976–2009 Diikuti olehKatie v. Peter: The Inside Story of Their Divorce Lady Gaga: Queen of Pop adalah biografi artis rekaman Amerika Serikat Lady Gaga, ditulis oleh Emily Herbert (nama pen...

شبكة توزيع الخطوط الجوية تقنية السفر (بالإنجليزية: Travel technology) (وتسمى أيضًا تقنية السياحة وأتمتة الضيافة) هي تطبيق لتقنية المعلومات (IT) أو تقنية المعلومات والاتصالات (ICT) في صناعة السفر والسياحة والضيافة. بعض أشكال تقنية السفر هي تتبع الرحلات، والتخطيط المسبق للسفر من خلال �...

American politician (1829–1897) John Mercer LangstonMember of the U.S. House of Representativesfrom Virginia's 4th districtIn officeSeptember 23, 1890 – March 3, 1891Preceded byEdward Carrington VenableSucceeded byJames F. EpesUnited States Minister Resident to the Dominican RepublicActingIn officeMarch 26, 1884 – June 23, 1885PresidentChester A. ArthurGrover ClevelandPreceded byPosition establishedSucceeded byJohn ThompsonUnited States Minister Resident to...
Paghimo ni bot Lsjbot. Alang sa ubang mga dapit sa mao gihapon nga ngalan, tan-awa ang Proctor Drain. 43°32′05″N 83°03′01″W / 43.53474°N 83.05022°W / 43.53474; -83.05022 Proctor Drain Kanal Nasod Tinipong Bansa Estado Michigan Kondado Sanilac County Gitas-on 232 m (761 ft) Tiganos 43°32′05″N 83°03′01″W / 43.53474°N 83.05022°W / 43.53474; -83.05022 Timezone EST (UTC-5) - summer (DST) EDT (UTC-4) Ge...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Juni 2012. Peristiwa Olo adalah peristiwa perlawanan fisik melawan penjajah yang dilakukan oleh pribumi dalam mempertahankan kemerdekaan Indonesia yang terjadi di kota Padang sekitar tahun 1947. Perlawanan ini dimulai dengan dibakarnya sebuah rumah yang dihuni oleh ...