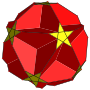
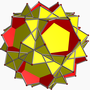
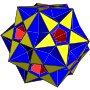
小星形截角十二面體
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Read other articles:
Literasi Digital Inklusif (disingkat LIDI) adalah bagian dari program Hackathon Merdeka yang spesifik mengajarkan literasi digital kepada anak-anak sekolah setara SMP di daerah-daerah di seluruh Indonesia. Karena targetnya adalah anak dan remaja, materi yang diajarkan adalah game development. Program ini pertama kali diinisiasi di Sleman, Yogyakarta, dan ditularkan ke daerah-daerah lain sebagai percontohan.[1][2][3] Catatan kaki ^ Sleman Menjadi Proyek Percontohan Lite...

American organization for broad-based interfaith fellowship This article relies excessively on references to primary sources. Please improve this article by adding secondary or tertiary sources. Find sources: Christian Churches Together – news · newspapers · books · scholar · JSTOR (April 2010) (Learn how and when to remove this template message) Christian Churches Together in the USA (CCT) is an organization formed in 2006 to broaden and expand fellow...

American film director (born 1978) Matt Bettinelli-OlpinBettinelli-Olpin in 2013Born (1978-02-19) February 19, 1978 (age 45)California, U.S.OccupationsFilm directorscreenwriteractormusicianYears active1993–presentMusical careerGenresPunk rockInstrument(s)Guitar LabelsAsian Man Musical artistWebsiteRadio Silence Matt Bettinelli-Olpin (born February 19, 1978) is an American director, writer, actor, and musician. He is a founding member of the punk band Link 80 and co-creator of the ...

Не плутати з Кароліна-Бугаз. У Вікіпедії є статті про інші значення цього терміна: Затока (значення). село Кароліно-Бугаз Герб Прапор Вигляд на Кароліно-БугазВигляд на Кароліно-Бугаз Країна Україна Область Одеська область Район Білгород-Дністровський район Громада

German newspaper The 19 June 1848 edition of Neue Rheinische Zeitung. The Neue Rheinische Zeitung: Organ der Demokratie (New Rhenish Newspaper: Organ of Democracy) was a German daily newspaper, published by Karl Marx in Cologne between 1 June 1848 and 19 May 1849. It is recognised by historians as one of the most important dailies of the Revolutions of 1848 in Germany. The paper was regarded by its editors and readers as the successor of an earlier Cologne newspaper, the Rheinische Zeitung (R...

US-based professional road bicycle racing team U.S. Postal Service Pro Cycling TeamDiscovery Channel Pro Cycling TeamTeam informationUCI codeDSCRegisteredUnited StatesFounded1988 (1988)Disbanded2007Discipline(s)RoadStatusUCI ProTeam (2005–2007)Key personnelGeneral managerBill StapletonTeam manager(s)Johan BruyneelTeam name history1988–19891992–199419951996–200320042005–2007Sunkyong (Amateur)Subaru–MontgomeryMontgomery–BellU.S. Postal ServiceU.S. Postal Service

River in Orange County, California, United States For other uses, see San Juan River (disambiguation). San Juan CreekSan Juan River[1]Hikers ford San Juan Creek below the hot springs, February 2008.Map of the San Juan Creek watershedEtymologyNamed for Mission San Juan Capistrano by Spanish conquistadors in the 1700s.LocationCountryUnited StatesStateCaliforniaRegionOrange County, Riverside CountyPhysical characteristicsSourcehead of San Juan Canyon, at the confluence of Morrell Ca...

Мы те, кто мы естьангл. We Are Who We Are Жанр подростковая драма Режиссёр Лука Гуаданьино В ролях Джек Дилан ГрейзерДжордан Кристин СимонХлоя СевиньиАлисе БрагаСкотт МескудиВера АлабиСпенс Мур IIФранческа СкорсезеБен ТейлорКори НайтТом Мерсье Композитор Дев Хайнс Страны &#...

本表是動態列表,可能永遠無法完結。歡迎您參考可靠來源來查漏補缺。 安徽省各地级市地区生产总值列表收录各主要年份安徽省地级行政区的地区生产总值(GDP)总量和人均GDP指标,当年数据参考次年底出版的《中国统计年鉴》和《安徽统计年鉴》。GDP指标有修订,地级区划单位GDP每隔五年的经济普查有修订。由于已知的技术原因,图表暂时不可用。带来不便,我们深表�...

Scottish actor McCredie at CamGlen Radio Colin McCredie (born 8 June 1972 in Dumbarton, Scotland) is a Scottish actor, best known for his role as DC Stuart Fraser in the STV drama Taggart, Nick Morrison in the BBC Scotland Soap Opera River City and in the films Shallow Grave, Night is Day and The Missing Postman. Biography Colin grew up in Perth and began acting as a child at Perth Theatre before attending the Scottish Youth Theatre. He now appears in CBeebies' Woolly and Tig with his real da...

Fonograf Fonograf (bahasa Yunani: φονε, suara; γραφο, tulisan) adalah mesin yang berguna untuk merekam suara. Suara disimpan setelah ditangkap oleh silinder. Fonograf, detail. Thomas Edison dengan fonografnya. Fonograf adalah mesin pertama yang dapat memainkan dan menyimpan suara. Pada model tertua dipasangkan pita suara di lapisan film metal yang tipis. Versi berikutnya menggunakan pita suara dari bahan lilin dan seluloid. Gelombang suara dipancarkan melalui membran ke baut logam (j...

Genus of birds Grey goose redirects here. For the vodka, see Grey Goose (vodka). For other uses, see Grey Goose (disambiguation). AnserTemporal range: Miocene-Holocene Pair of greylag geese, Anser anser Scientific classification Domain: Eukaryota Kingdom: Animalia Phylum: Chordata Class: Aves Order: Anseriformes Family: Anatidae Tribe: AnseriniVigors 1825 Genus: AnserBrisson, 1760 Type species Anas anser[1]Linnaeus, 1758 Species Anser albifrons Anser anser A. a. anser A. a. rubrirostr...

Season of television series ArcherSeason 2DVD coverStarring H. Jon Benjamin Judy Greer Amber Nash Chris Parnell Aisha Tyler Jessica Walter Country of originUnited StatesNo. of episodes13ReleaseOriginal networkFXOriginal releaseJanuary 27 (2011-01-27) –April 21, 2011 (2011-04-21)Season chronology← PreviousSeason 1Next →Season 3List of episodes The second season of the animated television series, Archer originally aired in the United States on the cable network FX...

River in New ZealandParinga RiverLocationCountryNew ZealandPhysical characteristicsMouth • locationTasman SeaLength37 kilometres (23 mi) The Paringa River is a river of the West Coast Region of New Zealand's South Island. It flows generally northwest from its origins in the Southern Alps west of Mount McCullaugh, reaching the Tasman Sea 10 km (6.2 mi) southwest of Bruce Bay.[1] Geologist Jeremy Kilner wrote his 2005 bachelor's with honours the...

ButterfieldTypePublicTraded asBSX: NTB.BHFounded1858HeadquartersHamilton, BermudaArea servedBahamas, Bermuda, Cayman Islands, Guernsey, Switzerland, United KingdomProductsbanking Butterfield, officially The Bank of N. T. Butterfield & Son Limited, is a bank founded and based in Bermuda. It provides services to clients from Bermuda, the Cayman Islands, Guernsey and Jersey, where its principal banking operations are located, and The Bahamas, Switzerland, Singapore and the United Kingdo...

British rock band Pale WavesPale Waves performing in June 2019Background informationOriginManchester, EnglandGenres Indie pop indie rock synth-pop pop-punk pop rock dream pop Years active2014–presentLabels Dirty Hit Interscope Members Heather Baron-Gracie Ciara Doran Hugo Silvani Charlie Wood Past members Ben Bateman Ryan Marsden Websitewww.palewaves.co.uk Pale Waves are an English rock band from Manchester, formed in 2014. It was founded originally as Creek when lead singer and guitarist H...

ヴィニー・ジョーンズ 2007年7月のジョーンズ名前本名 ヴィンセント・ピーター・ジョーンズVincent Peter Jonesラテン文字 Vinnie Jones基本情報国籍 ウェールズ生年月日 (1965-01-05) 1965年1月5日(58歳)出身地 ワトフォード身長 185cm選手情報ポジション MF利き足 右足 クラブ1年 クラブ 出場 (得点)1984-1986 ウィールドストーン 38 (2)1986 → ホルムスンド(loan) 22 (1)1986-1989 ウィンブルド�...

精神分析学のシリーズ記事 精神分析学 概念 エリクソンの心理社会的発達理論 心理性的発達理論 意識 前意識 無意識 精神装置(英語版) イド・エゴ・スーパーエゴ リビドー 動因理論(英語版) 転移 逆転移 防衛機制 抵抗(英語版) 投影 否認 重要人物 アルフレッド・アドラー マイケル・バリント ウィルフレッド・ビオン ヨーゼフ・ブロイアー ナンシー・チョド�...

يشكل القلب والرئتين الموقع الرئيس لتبادل الغازات[1] في الثديات، يؤدي مجال كهربائي عابر (مؤقت ثم يعود وهكذا دواليك) في القلب حيث يؤدي المجال الكهربائي إلى فصل الغازات تبعاً لشحناتها، تختلف شدة المجال الكهربائي في القلب من موقع ووقت لآخر وهو ما يؤدي لنمط نبضات القلب المخ...

Bu atamaning boshqa maʼnolari ham mavjud. Qarang: Kamon (maʼnolari). Kamon (musiqada) — 1) bir uchidan ikkinchisiga qil tutami tortilgan tayoqcha; gʻijjak, skripka kabi torli cholgʻu asboblarini chalish uchun ishlatiladi. Kamonning ishqalanishidan tor tebranib tovush chiqaradi. Tuzilishi jihatdan kamonga nisbatan takomillashgan smichok hozir kamon oʻrnida qoʻllanadi va u ham kamon deb yuritiladi; 2) Kavkaz, Oʻrta va Yaqin Sharq mamlakatlarida gʻijjakning nomi, kamoncha deb ham atala...