Anneau atomique
|
Read other articles:

Reports into UK disasters This article's lead section may be too short to adequately summarize the key points. Please consider expanding the lead to provide an accessible overview of all important aspects of the article. (November 2021) The Cullen Report can refer to one of three reports of public inquiries into UK disasters that were overseen by William Cullen, Baron Cullen of Whitekirk. Piper Alpha The first Cullen Report was prompted by Occidental Petroleum's Piper Alpha disaster on 6 July...
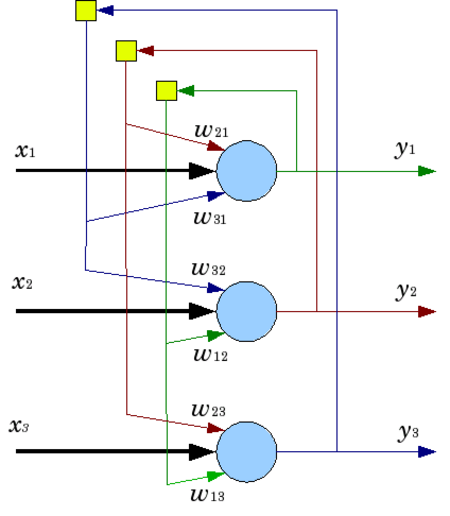
Нейро́нная сеть Хо́пфилда (англ. Hopfield network) — полносвязная нейронная сеть с симметричной матрицей связей. В процессе работы динамика таких сетей сходится (конвергирует) к одному из положений равновесия. Эти положения равновесия определяются заранее в процессе обуче

يفتقر محتوى هذه المقالة إلى الاستشهاد بمصادر. فضلاً، ساهم في تطوير هذه المقالة من خلال إضافة مصادر موثوق بها. أي معلومات غير موثقة يمكن التشكيك بها وإزالتها. (فبراير 2016) الجبل الأسود في الألعاب الأولمبية علم الجبل الأسود رمز ل.أ.د. MNE ل.أ.و. اللجنة الأولمبية للجبل ال

2012 film directed by Anna Muylaert Chamada a CobrarTheatrical release posterDirected byAnna MuylaertWritten byAnna MuylaertStarringCida AlmeidaMaria ManoelaLourenço MutarelliPierre SantosTatiana ThoméCinematographyMarcelo TrottaEdited byAndré FinottiMusic byRica AmabisTejo DamascenoProductioncompanyAfrica FilmesDistributed byGullane EntretenimentoRelease dates 8 October 2012 (2012-10-08) (Festival do Rio)[1] 2 August 2013 (2013-08-02) (Brazil)...

خوليان بيستييرو، غليسيان إنتيليكتوالمعلومات عامةالتقسيم الإداري ليغانيس البلد إسبانيا شبكة المواصلات مترو مدريد المالك Consorcio Regional de Transportes de Madrid (en) الإدارة Metro de Madrid S.A. (en) الخطوط Madrid Metro Line 12 (en) المحطات المجاورة إل كاراسكالعلى الخط: Madrid Metro Line 12 (en) باتجاه: بويرتا ديل سور �...

Копиці снопів пшениці. Обжинко́ві пісні — народно-поетичні обрядові твори, які виконують під час жнив і обжинків (свято завершення збирання врожаю). Найповніша збірка обжинкових пісень — «Жниварські пісні», 1971. Див. також Русальні пісні Веснянки Купальські пісні З�...

Giampaolo Pazzini Informasi pribadiNama lengkap Giampaolo Pazzini[1]Tanggal lahir 2 Agustus 1984 (umur 39)Tempat lahir Pescia, ItalyTinggi 180 m (590 ft 7 in)[2]Posisi bermain StrikerKarier junior1999–2003 AtalantaKarier senior*Tahun Tim Tampil (Gol)2003–2005 Atalanta 51 (12)2005–2009 Fiorentina 108 (25)2009–2011 Sampdoria 75 (36)2011–2012 Inter Milan 50 (16)2012–2015 AC Milan 74 (21)2015–2020 Verona 128 (49)2018 → Levante (loan) 9 (1)Total ...

Ця стаття про кримінальну банду. Про телесеріал див. Гострі картузи. «Дитина» Гаррі Фаулз, член банди «Заго́стрені козирки́» — це злочинне угруповання, що діяло в Бірмінгемі, Англія, в кінці XIX століття і менш активно на початку XX століття. Як говорять у народі, члени ба�...

1994 United States Senate election in Texas ← 1993 (special) November 8, 1994 2000 → Nominee Kay Bailey Hutchison Richard W. Fisher Party Republican Democratic Popular vote 2,604,218[1] 1,639,615[1] Percentage 60.85% 37.31% County resultsHutchison: 40-50% 50–60% 60–70% 70–80% 80–90% ...

I Found YouSingle by Benny Blanco and Calvin Harrisfrom the album Friends Keep Secrets ReleasedNovember 2, 2018 (2018-11-02)RecordedOctober 2018Genre Dance-pop house nu-disco Length3:11LabelFriends Keep SecretsInterscopeSongwriter(s)Benny BlancoAdam WilesProducer(s)Benny BlancoCalvin HarrisBenny Blanco singles chronology Eastside (2018) I Found You (2018) Better to Lie (2018) Calvin Harris singles chronology Promises(2018) I Found You(2018) Checklist[1][2 ...

Throughout World War II, the Empire of Japan created a number of puppet states that played a noticeable role in the war by collaborating with Imperial Japan. With promises of Asia for the Asiatics cooperating in a Greater East Asia Co-Prosperity Sphere, Japan also sponsored or collaborated with parts of nationalist movements in several Asian countries colonised by European empires or the United States.[1] The Japanese recruited volunteers from several occupied regions and also from am...

Artikel ini tidak memiliki referensi atau sumber tepercaya sehingga isinya tidak bisa dipastikan. Tolong bantu perbaiki artikel ini dengan menambahkan referensi yang layak. Tulisan tanpa sumber dapat dipertanyakan dan dihapus sewaktu-waktu.Cari sumber: NetBIOS – berita · surat kabar · buku · cendekiawan · JSTOR NetBIOS (singkatan dari istilah dalam bahasa Inggris: Network Basic Input/Output System) adalah sebuah spesifikasi yang dibuat oleh Internation...

Protected area in Estonia Anija Nature ReserveLocationEstoniaCoordinates59°22′21″N 25°16′34″E / 59.37250°N 25.27611°E / 59.37250; 25.27611Area72 haEstablished1990 (1999) Anija Nature Reserve is a nature reserve which is located in Harju County, Estonia.[1] The area of the nature reserve is 72 ha.[2] The protected area was founded in 1990 on the basis of Anija botanical-landscape conservation area (Estonian: Anija botaanilis-maastikuline...

This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: British Polling Council – news · newspapers · books · scholar · JSTOR (April 2017) (Learn how and when to r...

Indian horror television series TypewriterPromotional posterGenreHorror dramaCreated bySujoy GhoshWritten bySujoy GhoshSuresh NairDirected bySujoy GhoshStarringPurab KohliPalomi GhoshJisshu SenguptaSameer KochharAarna SharmaBijou ThaangjamMikhail GandhiPalash KambleAaryansh MalviyaTheme music composerRaja Narayan DebComposerRaja Narayan DebCountry of originIndiaOriginal languageHindiNo. of seasons1No. of episodes5 (list of episodes)ProductionExecutive producerSujoy GhoshProduction locationsBa...

Farmer Phil's FestivalGenreRock, ska, reggae, cover bandsDatesAugustLocation(s)Near Gatten Farm, Ratlinghope, Shropshire, EnglandYears active1998–presentWebsitehttp://www.farmerphilsfestival.com Farmer Phil's Festival is a music festival that takes place in early August at Near Gatten Farm, near Ratlinghope, Shropshire. The festival was founded by, and is managed by the eponymous Philip Harding and was first held in 1998. History Main Stage in 2017 - Ferocious Dog on stage Early festivals w...

Movie theatre in Istanbul Atlas CinemaAtlas SinemasıAtlas CinemaLocation within IstanbulAddressİstiklal Avenue 133IstanbulTurkeyLocationBeyoğluCoordinates41°02′02″N 28°58′47″E / 41.0339°N 28.9796°E / 41.0339; 28.9796OwnerMinistry of Treasury and FinanceTypeCinemaCapacity500, 130, 85ConstructionBuilt1877; 146 years ago (1877)OpenedFebruary 19, 1948; 75 years ago (1948-02-19)Renovated2019–2021BuilderAgop KöçeyanWebsi...

Merah Manggis Belahan manggisCommon connotationsKulit dalam manggis Koordinat warnaTriplet hex#800000sRGBB (r, g, b)(128, 0, 0)HSV (h, s, v)(0°, 100%, 50%)SumberDaftar Istilah Warna[1]X11[2]B: Dinormalkan ke [0–255] (bita) Merah manggis merupakan salah satu corak warna merah tua. Warna ini juga dikenal dengan sebutan merah marun, merah pulasan, dan merun. Warna ini menyerupai warna kulit dalam manggis yang berwarna ke...

Индоарийские языки Классификация индоевропейские языки индоиранские языки индоарийские языки ISO 639-5 inc LINGUIST List inda ABS ASCL 52 IETF inc Glottolog indo1321 Распространение современных индоарийских и дардских языков Центральная и восточно-центральная зоны ...

Mountain range along the eastern coast of India Eastern GhatsGodavari River at Papi Hills of the Eastern Ghats in Andhra PradeshHighest pointPeakSitamma KondaElevation1,680 m (5,510 ft)[1]Prominence1,680 m (5,510 ft)GeographyCountryIndiaStatesOdisha, Andhra Pradesh, Tamil Nadu, Karnataka and TelanganaRegionsEastern India and South IndiaBiomeTropical forests and WetlandsGeologyType of rockIgneous, Iron and Limestone The Eastern Ghats are a discontinuous range o...

![{\displaystyle \mathbb {Z} +X\mathbb {Q} [X]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/6c9036dd9806a6fe9130fdc852af176b288b1728)
