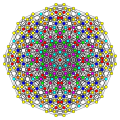
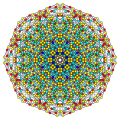
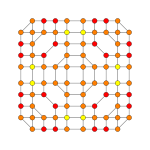
Hexicated 7-cubes

Orthogonal projections in B4 Coxeter plane

7-cube
Hexicated 7-cube
Hexitruncated 7-cube
Hexicantellated 7-cube

Hexiruncinated 7-cube
Hexicantitruncated 7-cube
Hexiruncitruncated 7-cube
Hexiruncicantellated 7-cube

Hexisteritruncated 7-cube
Hexistericantellated 7-cube
Hexipentitruncated 7-cube
Hexiruncicantitruncated 7-cube

Hexistericantitruncated 7-cube
Hexisteriruncitruncated 7-cube
Hexisteriruncicantellated 7-cube
Hexipenticantitruncated 7-cube

Hexipentiruncitruncated 7-cube
Hexisteriruncicantitruncated 7-cube
Hexipentiruncicantitruncated 7-cube
Hexipentistericantitruncated 7-cube

Hexipentisteriruncicantitruncated 7-cube
(Omnitruncated 7-cube)

In seven-dimensional geometry, a hexicated 7-cube is a convex uniform 7-polytope, including 6th-order truncations (hexication) from the regular 7-cube.

There are 32 hexications for the 7-cube, including all permutations of truncations, cantellations, runcinations, sterications, and pentellations. 20 are represented here, while 12 are more easily constructed from the 7-orthoplex.

The simple hexicated 7-cube is also called an expanded 7-cube, with only the first and last nodes ringed, is constructed by an expansion operation applied to the regular 7-cube. The highest form, the hexipentisteriruncicantitruncated 7-cube is more simply called a omnitruncated 7-cube with all of the nodes ringed.

These polytope are among a family of 127 uniform 7-polytopes with B7 symmetry.

Hexicated 7-cube

Hexicated 7-cube
Type uniform 7-polytope
Schläfli symbol t0,6{4,35}
Coxeter-Dynkin diagrams
6-faces
5-faces
4-faces
Cells
Faces
Edges
Vertices
Vertex figure
Coxeter groups B7, [4,35]
Properties convex

In seven-dimensional geometry, a hexicated 7-cube is a convex uniform 7-polytope, a hexication (6th order truncation) of the regular 7-cube, or alternately can be seen as an expansion operation.

Alternate names

  • Small petated hepteract (acronym: ) (Jonathan Bowers)

Images

orthographic projections
Coxeter plane B7 / A6 B6 / D7 B5 / D6 / A4
Graph
Dihedral symmetry [14] [12] [10]
Coxeter plane B4 / D5 B3 / D4 / A2 B2 / D3
Graph
Dihedral symmetry [8] [6] [4]
Coxeter plane A5 A3
Graph
Dihedral symmetry [6] [4]

Hexitruncated 7-cube

hexitruncated 7-cube
Type uniform 7-polytope
Schläfli symbol t0,1,6{4,35}
Coxeter-Dynkin diagrams
6-faces
5-faces
4-faces
Cells
Faces
Edges
Vertices
Vertex figure
Coxeter groups B7, [4,35]
Properties convex

Alternate names

  • Petitruncated hepteract (acronym: ) (Jonathan Bowers)

Images

orthographic projections
Coxeter plane B7 / A6 B6 / D7 B5 / D6 / A4
Graph
Dihedral symmetry [14] [12] [10]
Coxeter plane B4 / D5 B3 / D4 / A2 B2 / D3
Graph
Dihedral symmetry [8] [6] [4]
Coxeter plane A5 A3
Graph
Dihedral symmetry [6] [4]

Hexicantellated 7-cube

Hexicantellated 7-cube
Type uniform 7-polytope
Schläfli symbol t0,2,6{4,35}
Coxeter-Dynkin diagrams
6-faces
5-faces
4-faces
Cells
Faces
Edges
Vertices
Vertex figure
Coxeter groups B7, [4,35]
Properties convex

Alternate names

  • Petirhombated hepteract (acronym: ) (Jonathan Bowers)

Images

orthographic projections
Coxeter plane B7 / A6 B6 / D7 B5 / D6 / A4
Graph
Dihedral symmetry [14] [12] [10]
Coxeter plane B4 / D5 B3 / D4 / A2 B2 / D3
Graph
Dihedral symmetry [8] [6] [4]
Coxeter plane A5 A3
Graph
Dihedral symmetry [6] [4]

Hexiruncinated 7-cube

Hexiruncinated 7-cube
Type uniform 7-polytope
Schläfli symbol t0,3,6{4,35}
Coxeter-Dynkin diagrams
6-faces
5-faces
4-faces
Cells
Faces
Edges
Vertices
Vertex figure
Coxeter groups B7, [4,35]
Properties convex

Alternate names

  • Petiprismated hepteract (acronym: ) (Jonathan Bowers)

Images

orthographic projections
Coxeter plane B7 / A6 B6 / D7 B5 / D6 / A4
Graph too complex
Dihedral symmetry [14] [12] [10]
Coxeter plane B4 / D5 B3 / D4 / A2 B2 / D3
Graph
Dihedral symmetry [8] [6] [4]
Coxeter plane A5 A3
Graph
Dihedral symmetry [6] [4]

Hexicantitruncated 7-cube

Hexicantitruncated 7-cube
Type uniform 7-polytope
Schläfli symbol t0,1,2,6{4,35}
Coxeter-Dynkin diagrams
6-faces
5-faces
4-faces
Cells
Faces
Edges
Vertices
Vertex figure
Coxeter groups B7, [4,35]
Properties convex

Alternate names

  • Petigreatorhombated hepteract (acronym: ) (Jonathan Bowers)

Images

orthographic projections
Coxeter plane B7 / A6 B6 / D7 B5 / D6 / A4
Graph
Dihedral symmetry [14] [12] [10]
Coxeter plane B4 / D5 B3 / D4 / A2 B2 / D3
Graph
Dihedral symmetry [8] [6] [4]
Coxeter plane A5 A3
Graph
Dihedral symmetry [6] [4]

Hexiruncitruncated 7-cube

Hexiruncitruncated 7-cube
Type uniform 7-polytope
Schläfli symbol t0,1,3,6{4,35}
Coxeter-Dynkin diagrams
6-faces
5-faces
4-faces
Cells
Faces
Edges
Vertices
Vertex figure
Coxeter groups B7, [4,35]
Properties convex

Alternate names

  • Petiprismatotruncated hepteract (acronym: ) (Jonathan Bowers)

Images

orthographic projections
Coxeter plane B7 / A6 B6 / D7 B5 / D6 / A4
Graph
Dihedral symmetry [14] [12] [10]
Coxeter plane B4 / D5 B3 / D4 / A2 B2 / D3
Graph
Dihedral symmetry [8] [6] [4]
Coxeter plane A5 A3
Graph
Dihedral symmetry [6] [4]

Hexiruncicantellated 7-cube

Hexiruncicantellated 7-cube
Type uniform 7-polytope
Schläfli symbol t0,2,3,6{4,35}
Coxeter-Dynkin diagrams
6-faces
5-faces
4-faces
Cells
Faces
Edges
Vertices
Vertex figure
Coxeter groups B7, [4,35]
Properties convex

In seven-dimensional geometry, a hexiruncicantellated 7-cube is a uniform 7-polytope.

Alternate names

  • Petiprismatorhombated hepteract (acronym: ) (Jonathan Bowers)

Images

orthographic projections
Coxeter plane B7 / A6 B6 / D7 B5 / D6 / A4
Graph
Dihedral symmetry [14] [12] [10]
Coxeter plane B4 / D5 B3 / D4 / A2 B2 / D3
Graph
Dihedral symmetry [8] [6] [4]
Coxeter plane A5 A3
Graph
Dihedral symmetry [6] [4]

Hexisteritruncated 7-cube

hexisteritruncated 7-cube
Type uniform 7-polytope
Schläfli symbol t0,1,4,6{4,35}
Coxeter-Dynkin diagrams
6-faces
5-faces
4-faces
Cells
Faces
Edges
Vertices
Vertex figure
Coxeter groups B7, [4,35]
Properties convex

Alternate names

  • Peticellitruncated hepteract (acronym: ) (Jonathan Bowers)

Images

orthographic projections
Coxeter plane B7 / A6 B6 / D7 B5 / D6 / A4
Graph
Dihedral symmetry [14] [12] [10]
Coxeter plane B4 / D5 B3 / D4 / A2 B2 / D3
Graph
Dihedral symmetry [8] [6] [4]
Coxeter plane A5 A3
Graph
Dihedral symmetry [6] [4]

Hexistericantellated 7-cube

hexistericantellated 7-cube
Type uniform 7-polytope
Schläfli symbol t0,2,4,6{4,35}
Coxeter-Dynkin diagrams
6-faces
5-faces
4-faces
Cells
Faces
Edges
Vertices
Vertex figure
Coxeter groups B7, [4,35]
Properties convex

Alternate names

  • Peticellirhombihepteract (acronym: ) (Jonathan Bowers)

Images

orthographic projections
Coxeter plane B7 / A6 B6 / D7 B5 / D6 / A4
Graph
Dihedral symmetry [14] [12] [10]
Coxeter plane B4 / D5 B3 / D4 / A2 B2 / D3
Graph
Dihedral symmetry [8] [6] [4]
Coxeter plane A5 A3
Graph
Dihedral symmetry [6] [4]

Hexipentitruncated 7-cube

Hexipentitruncated 7-cube
Type uniform 7-polytope
Schläfli symbol t0,1,5,6{4,35}
Coxeter-Dynkin diagrams
6-faces
5-faces
4-faces
Cells
Faces
Edges
Vertices
Vertex figure
Coxeter groups B7, [4,35]
Properties convex

Alternate names

  • Petiteritruncated hepteract (acronym: ) (Jonathan Bowers)

Images

orthographic projections
Coxeter plane B7 / A6 B6 / D7 B5 / D6 / A4
Graph
Dihedral symmetry [14] [12] [10]
Coxeter plane B4 / D5 B3 / D4 / A2 B2 / D3
Graph
Dihedral symmetry [8] [6] [4]
Coxeter plane A5 A3
Graph
Dihedral symmetry [6] [4]

Hexiruncicantitruncated 7-cube

Hexiruncicantitruncated 7-cube
Type uniform 7-polytope
Schläfli symbol t0,1,2,3,6{4,35}
Coxeter-Dynkin diagrams
6-faces
5-faces
4-faces
Cells
Faces
Edges
Vertices
Vertex figure
Coxeter groups B7, [4,35]
Properties convex

Alternate names

  • Petigreatoprismated hepteract (acronym: ) (Jonathan Bowers)

Images

orthographic projections
Coxeter plane B7 / A6 B6 / D7 B5 / D6 / A4
Graph
Dihedral symmetry [14] [12] [10]
Coxeter plane B4 / D5 B3 / D4 / A2 B2 / D3
Graph
Dihedral symmetry [8] [6] [4]
Coxeter plane A5 A3
Graph too complex too complex
Dihedral symmetry [6] [4]

Hexistericantitruncated 7-cube

Hexistericantitruncated 7-cube
Type uniform 7-polytope
Schläfli symbol t0,1,2,4,6{4,35}
Coxeter-Dynkin diagrams
6-faces
5-faces
4-faces
Cells
Faces
Edges
Vertices
Vertex figure
Coxeter groups B7, [4,35]
Properties convex

Alternate names

  • Peticelligreatorhombated hepteract (acronym: ) (Jonathan Bowers)

Images

orthographic projections
Coxeter plane B7 / A6 B6 / D7 B5 / D6 / A4
Graph too complex
Dihedral symmetry [14] [12] [10]
Coxeter plane B4 / D5 B3 / D4 / A2 B2 / D3
Graph
Dihedral symmetry [8] [6] [4]
Coxeter plane A5 A3
Graph
Dihedral symmetry [6] [4]

Hexisteriruncitruncated 7-cube

Hexisteriruncitruncated 7-cube
Type uniform 7-polytope
Schläfli symbol t0,1,3,4,6{4,35}
Coxeter-Dynkin diagrams
6-faces
5-faces
4-faces
Cells
Faces
Edges
Vertices
Vertex figure
Coxeter groups B7, [4,35]
Properties convex

Alternate names

  • Peticelliprismatotruncated hepteract (acronym: ) (Jonathan Bowers)

Images

orthographic projections
Coxeter plane B7 / A6 B6 / D7 B5 / D6 / A4
Graph too complex
Dihedral symmetry [14] [12] [10]
Coxeter plane B4 / D5 B3 / D4 / A2 B2 / D3
Graph
Dihedral symmetry [8] [6] [4]
Coxeter plane A5 A3
Graph
Dihedral symmetry [6] [4]

Hexisteriruncicantellated 7-cube

Hexisteriruncitruncated 7-cube
Type uniform 7-polytope
Schläfli symbol t0,2,3,4,6{4,35}
Coxeter-Dynkin diagrams
6-faces
5-faces
4-faces
Cells
Faces
Edges
Vertices
Vertex figure
Coxeter groups B7, [4,35]
Properties convex

Alternate names

  • Peticelliprismatorhombihepteract (acronym: ) (Jonathan Bowers)

Images

orthographic projections
Coxeter plane B7 / A6 B6 / D7 B5 / D6 / A4
Graph too complex
Dihedral symmetry [14] [12] [10]
Coxeter plane B4 / D5 B3 / D4 / A2 B2 / D3
Graph
Dihedral symmetry [8] [6] [4]
Coxeter plane A5 A3
Graph
Dihedral symmetry [6] [4]

Hexipenticantitruncated 7-cube

hexipenticantitruncated 7-cube
Type uniform 7-polytope
Schläfli symbol t0,1,2,5,6{4,35}
Coxeter-Dynkin diagrams
6-faces
5-faces
4-faces
Cells
Faces
Edges
Vertices
Vertex figure
Coxeter groups B7, [4,35]
Properties convex

Alternate names

  • Petiterigreatorhombated hepteract (acronym: ) (Jonathan Bowers)

Images

orthographic projections
Coxeter plane B7 / A6 B6 / D7 B5 / D6 / A4
Graph
Dihedral symmetry [14] [12] [10]
Coxeter plane B4 / D5 B3 / D4 / A2 B2 / D3
Graph
Dihedral symmetry [8] [6] [4]
Coxeter plane A5 A3
Graph
Dihedral symmetry [6] [4]

Hexipentiruncitruncated 7-cube

Hexisteriruncicantitruncated 7-cube
Type uniform 7-polytope
Schläfli symbol t0,1,2,3,4,6{4,35}
Coxeter-Dynkin diagrams
6-faces
5-faces
4-faces
Cells
Faces
Edges
Vertices
Vertex figure
Coxeter groups B7, [4,35]
Properties convex

Alternate names

  • Great petacellated hepteract (acronym: ) (Jonathan Bowers)

Images

orthographic projections
Coxeter plane B7 / A6 B6 / D7 B5 / D6 / A4
Graph too complex
Dihedral symmetry [14] [12] [10]
Coxeter plane B4 / D5 B3 / D4 / A2 B2 / D3
Graph
Dihedral symmetry [8] [6] [4]
Coxeter plane A5 A3
Graph
Dihedral symmetry [6] [4]

Hexisteriruncicantitruncated 7-cube

Hexisteriruncicantitruncated 7-cube
Type uniform 7-polytope
Schläfli symbol t0,1,2,3,4,6{4,35}
Coxeter-Dynkin diagrams
6-faces
5-faces
4-faces
Cells
Faces
Edges
Vertices
Vertex figure
Coxeter groups B7, [4,35]
Properties convex

Alternate names

  • Great petacellated hepteract (acronym: ) (Jonathan Bowers)

Images

orthographic projections
Coxeter plane B7 / A6 B6 / D7 B5 / D6 / A4
Graph too complex
Dihedral symmetry [14] [12] [10]
Coxeter plane B4 / D5 B3 / D4 / A2 B2 / D3
Graph
Dihedral symmetry [8] [6] [4]
Coxeter plane A5 A3
Graph
Dihedral symmetry [6] [4]

Hexipentiruncicantitruncated 7-cube

Hexipentiruncicantitruncated 7-cube
Type uniform 7-polytope
Schläfli symbol t0,1,2,3,5,6{4,35}
Coxeter-Dynkin diagrams
6-faces
5-faces
4-faces
Cells
Faces
Edges
Vertices
Vertex figure
Coxeter groups B7, [4,35]
Properties convex

Alternate names

  • Petiterigreatoprismated hepteract (acronym: ) (Jonathan Bowers)

Images

orthographic projections
Coxeter plane B7 / A6 B6 / D7 B5 / D6 / A4
Graph too complex
Dihedral symmetry [14] [12] [10]
Coxeter plane B4 / D5 B3 / D4 / A2 B2 / D3
Graph
Dihedral symmetry [8] [6] [4]
Coxeter plane A5 A3
Graph
Dihedral symmetry [6] [4]

Hexipentistericantitruncated 7-cube

Hexipentistericantitruncated 7-cube
Type uniform 7-polytope
Schläfli symbol t0,1,2,4,5,6{4,35}
Coxeter-Dynkin diagrams
6-faces
5-faces
4-faces
Cells
Faces
Edges
Vertices
Vertex figure
Coxeter groups B7, [4,35]
Properties convex

Alternate names

  • Petitericelligreatorhombihepteract (acronym: putcagroh) (Jonathan Bowers)

Images

orthographic projections
Coxeter plane B7 / A6 B6 / D7 B5 / D6 / A4
Graph too complex
Dihedral symmetry [14] [12] [10]
Coxeter plane B4 / D5 B3 / D4 / A2 B2 / D3
Graph
Dihedral symmetry [8] [6] [4]
Coxeter plane A5 A3
Graph
Dihedral symmetry [6] [4]

Omnitruncated 7-cube

Omnitruncated 7-cube
Type uniform 7-polytope
Schläfli symbol t0,1,2,3,4,5,6{36}
Coxeter-Dynkin diagrams
6-faces
5-faces
4-faces
Cells
Faces
Edges
Vertices
Vertex figure
Coxeter groups B7, [4,35]
Properties convex

The omnitruncated 7-cube is the largest uniform 7-polytope in the B7 symmetry of the regular 7-cube. It can also be called the hexipentisteriruncicantitruncated 7-cube which is the long name for the omnitruncation for 7 dimensions, with all reflective mirrors active.

Alternate names

  • Great petated hepteract (Acronym: ) (Jonathan Bowers)

Images

orthographic projections
Coxeter plane B7 / A6 B6 / D7 B5 / D6 / A4
Graph too complex
Dihedral symmetry [14] [12] [10]
Coxeter plane B4 / D5 B3 / D4 / A2 B2 / D3
Graph
Dihedral symmetry [8] [6] [4]
Coxeter plane A5 A3
Graph
Dihedral symmetry [6] [4]

Notes

References

  • H.S.M. Coxeter:
    • H.S.M. Coxeter, Regular Polytopes, 3rd Edition, Dover New York, 1973
    • Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6 [1]
      • (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380-407, MR 2,10]
      • (Paper 23) H.S.M. Coxeter, Regular and Semi-Regular Polytopes II, [Math. Zeit. 188 (1985) 559-591]
      • (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III, [Math. Zeit. 200 (1988) 3-45]
  • Norman Johnson Uniform Polytopes, Manuscript (1991)
    • N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, PhD (1966)
  • Klitzing, Richard. "7D uniform polytopes (polyexa)". x3o3o3o3o3o4x - , x3x3o3o3o3o3x- , x3o3o3x3o3o4x - , x3x3x3o3o3o4x - , x3x3o3x3o3o4x - , x3o3x3x3o3o4x - , x3o3x3o3o3x4x - , x3o3x3o3x3o4x - , x3x3o3o3o3x4x - , x3x3x3x3o3o4x - , x3x3x3o3x3o4x - , x3x3o3x3x3o4x - , x3o3x3x3x3o4x - , x3x3x3oxo3x4x - , x3x3x3x3x3o4x - , x3x3x3o3x3x4x - , x3x3o3x3x3x4x - , x3x3x3x3x3x4x -
Fundamental convex regular and uniform polytopes in dimensions 2–10
Family An Bn I2(p) / Dn E6 / E7 / E8 / F4 / G2 Hn
Regular polygon Triangle Square p-gon Hexagon Pentagon
Uniform polyhedron Tetrahedron Octahedron • Cube Demicube Dodecahedron • Icosahedron
Uniform polychoron Pentachoron 16-cell • Tesseract Demitesseract 24-cell 120-cell • 600-cell
Uniform 5-polytope 5-simplex 5-orthoplex • 5-cube 5-demicube
Uniform 6-polytope 6-simplex 6-orthoplex • 6-cube 6-demicube 122 • 221
Uniform 7-polytope 7-simplex 7-orthoplex • 7-cube 7-demicube 132 • 231 • 321
Uniform 8-polytope 8-simplex 8-orthoplex • 8-cube 8-demicube 142 • 241 • 421
Uniform 9-polytope 9-simplex 9-orthoplex • 9-cube 9-demicube
Uniform 10-polytope 10-simplex 10-orthoplex • 10-cube 10-demicube
Uniform n-polytope n-simplex n-orthoplex • n-cube n-demicube 1k2 • 2k1 • k21 n-pentagonal polytope
Topics: Polytope families • Regular polytope • List of regular polytopes and compounds

Read other articles:

Pulau Tomia

Pulau TomiaPeta lokasi Pulau TomiaNegaraIndonesiaGugus kepulauanTukang besiProvinsiSulawesi TenggaraKabupatenKabupaten WakatobiLuas115 km²[1]Populasi15.789 Pulau Tomia adalah salah satu pulau di gugusan kepulauan tukang besi wilayah Kabupaten Wakatobi, provinsi Sulawesi Tenggara, Indonesia. Pulau ini terletak di selatan Pulau Kaledupa dan di Utara pulau Binongko. Pulau-pulau sekitar Di sekitar pulau Tomia terdapat beberapa pulau kecil yang terdiri dari: Pulau Kaledupa Pulau Bino...

 

Chata pri Zelenom plese

Chata pri Zelenom plese Die Chata pri Zelenom plese mit dem fast 600 Höhenmeter überragenden Gipfel der Jastrabia veža Die Chata pri Zelenom plese mit dem fast 600 Höhenmeter überragenden Gipfel der Jastrabia veža Lage Dolina Zeleného plesa; Slowakei; Talort: Tatranská Lomnica, Vysoké Tatry Gebirgsgruppe Hohe Tatra Geographische Lage: 49° 12′ 36,5″ N, 20° 13′ 16,8″ O49.21014120.221321551Koordinaten: 49° 12′ 36,5″ N...

 

Keyakinan

Keyakinan adalah sebuah sikap subyektif bahwa sesuatu atau proposisi itu benar.[1] Dalam epistemologi, para filsuf menggunakan istilah kepercayaan untuk merujuk pada sikap tentang dunia yang bisa benar atau salah.[2] Memercayai sesuatu berarti menganggapnya benar; misalnya, percaya bahwa salju itu putih sebanding dengan menerima kebenaran proposisi salju berwarna putih. Namun, memegang keyakinan tidak membutuhkan introspeksi aktif. Misalnya, hanya sedikit yang mempertimbangkan...

Therapeutic touch

Pseudoscientific energy therapy Therapeutic touchAlternative therapyMeSHD019124 Part of a series onParanormal Main articles Astral projection Astrology Aura Bilocation Breatharianism Clairvoyance Close encounter Cold spot Crystal gazing Conjuration Cryptozoology Demonic possession Demonology Ectoplasm Electronic voice phenomenon Exorcism Extrasensory perception Forteana Fortune-telling Ghost hunting Indigo children Magic Mediumship Miracle Occult Orb Ouija Paranormal fiction Paranormal televi...

 

CPR-1000

Kerncentrale Daya Bay Een CPR-1000-kernreactor is een Chinese kernreactor van het type drukwaterreactor die 1080 megawatt elektrisch vermogen levert. De CPR-1000 wordt gebouwd en bedreven door China Guangdong Nuclear Power Company (CGNPC). De CPR-1000 lijkt in opbouw op de kerncentrale Grevelingen. Hij is immers gebaseerd op een Franse kernreactor van 900 MW in 1990 door Areva ingevoerd in China. Op 15 juli 2010 ging de eerste CPR-1000 in bedrijf[1] voor Ling Ao Nuclear Power Plant ...

 

2014 Al-Safira offensive

Military operation launched by Syrian rebels 2014 Al-Safira offensivePart of the Syrian Civil WarDate8–12 October 2014(4 days)LocationAl-Safira, Aleppo Governorate, SyriaResult Syrian Army victoryTerritorialchanges The Army recaptures all villages by 12 October[1][2] Rebels initially capture seven villages near the Defense Factories[3][4][5]Belligerents Islamic Front Syrian Arab Republic Syrian Armed Forces National Defense ForceCommanders and le...

1971 Philadelphia mayoral election

1971 mayoral election in Philadelphia, Pennsylvania 1971 Philadelphia mayoral election ← 1967 November 2, 1971 1975 → Turnout77%[1] 4 pp   Nominee Frank Rizzo Thacher Longstreth Party Democratic Republican Popular vote 394,067 345,912 Percentage 52.87% 46.41% Mayor before election James Tate Democratic Elected Mayor Frank Rizzo Democratic Elections in Pennsylvania Federal government U.S. President 1789 1792 1796 1800 1804 1808 1812 1816 1820 1824 1828 1...

 

2016 IIHF Women's World Championship

2016 edition of the IIHF Women's World Championship See also: 2016 Women's Ice Hockey World Championships 2016 IIHF Women's World ChampionshipTournament detailsHost country CanadaDates28 March – 4 April 2016Opened byDavid JohnstonTeams8Venue(s)2 (in 1 host city)Final positionsChampions  United States (7th title)Runner-up  CanadaThird place  RussiaFourth place FinlandTournament statisticsGames played21Goals scored108 (5.14 per game)...

 

Goblin (film)

2010 Canadian film This article is missing information about the film's production and release. Please expand the article to include this information. Further details may exist on the talk page. (April 2015) GoblinWritten byRaul InglisDirected byJeffery Scott LandoStarringGil BellowsTracy SpiridakosCamille SullivanDonnelly RhodesReilly DolmanAndrew WheelerColin CunninghamErin BoyesJordan MooreBrett DierTheme music composerChristopher NickelCountry of originCanadaOriginal languageEnglishProduc...

The Moray Eels Eat The Holy Modal Rounders

1968 studio album by The Holy Modal RoundersThe Moray Eels Eat The Holy Modal RoundersStudio album by The Holy Modal RoundersReleased1968GenrePsychedelic folk[1]Length26:24LabelElektraProducerFrazier MohawkThe Holy Modal Rounders chronology Indian War Whoop(1967) The Moray Eels Eat The Holy Modal Rounders(1968) Good Taste Is Timeless(1971) The Moray Eels Eat the Holy Modal Rounders is the fourth studio album by the New York psychedelic folk band the Holy Modal Rounders, releas...

 

1867 in Canada

Canada-related events during the year of 1867 This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: 1867 in Canada – news · newspapers · books · scholar · JSTOR (September 2023) (Learn how and when to remove this template message) ← 1866 1865 1864 1867 in Canada → 1868 1869 1870 Decades: 1840s 1850s 18...

 

Jesper Parnevik

Swedish professional golfer Jesper ParnevikParnevik in April 2007Personal informationFull nameJesper Bo ParnevikNicknameSpacemanBorn (1965-03-07) 7 March 1965 (age 58)Botkyrka, SwedenHeight1.83 m (6 ft 0 in)Sporting nationality SwedenResidenceJupiter, Florida, U.S.SpouseMia ParnevikChildren4, including PegCareerCollegePalm Beach Junior CollegeTurned professional1986Current tour(s)PGA Tour ChampionsFormer tour(s)PGA TourEuropean TourProfessional wins15Highest ranking7 ...

Kantai Collection (TV series)

Japanese anime television series This article is about the anime television series. For the original game, see Kantai Collection. Kantai CollectionCover of the first Blu-ray volume featuring (from left to right) Yūdachi, Fubuki and Mutsuki.艦隊これくしょん -艦これ-(Kantai Korekushon: Kankore)GenreAction, comedy, militaryCreated byDMM.comKadokawa GamesC2 Praparat (season 2) Anime television seriesKantai Collection: KanColle (season 1)KanColle: Let's Meet at Sea (season 2)Direc...

 

The Lottery (play)

This article is about the play by Henry Fielding. For the short story by Shirley Jackson, see The Lottery. Titlepage to The Lottery: a Farce The Lottery is a play by Henry Fielding and was a companion piece to Joseph Addison's Cato. As a ballad opera, it contained 19 songs and was a collaboration with Mr Seedo, a musician. It first ran on 1 January 1732 at the Theatre Royal, Drury Lane. The play tells the story of a man in love with a girl. She claims she has won a lottery, however, making an...

 

Luna Park, Pittsburgh

Amusement park For other amusement parks known by the same name, see Luna Park. Luna ParkThe entrance to the parkLocationPittsburgh, PennsylvaniaCoordinates40°27′18″N 79°57′10″W / 40.4551°N 79.9528°W / 40.4551; -79.9528StatusDefunctOpened1905Closed1909 (1909)OwnerFrederick IngersollArea16 acres (6.5 ha) Luna Park was an amusement park in the North Oakland neighborhood of the city of Pittsburgh, Pennsylvania, USA, from 1905 to 1909.[1] Cons...

Gustavo Meza

Gustavo Meza Gustavo Meza en 2008Información personalNombre de nacimiento Gustavo Adolfo Meza Wevar Nacimiento 1936 Osorno (Chile) Nacionalidad ChilenaLengua materna Español FamiliaPadre Pelegrín Meza Loyola Cónyuge Delfina Guzmán (1959-1969)Yael Unger (1969-1991)Elsa Poblete (desde 1992) EducaciónEducado en Universidad de Chile (Psicología y Teatro) Alumno de Pedro Orthous Información profesionalOcupación Actor, autor teatral y director de teatro Empleador Univer...

 

The Kingslayer

1949 short story collection by L. Ron Hubbard The Kingslayer Dust-jacket from the first editionAuthorL. Ron HubbardCover artistWilliam BenulisCountryUnited StatesLanguageEnglishGenreScience fictionPublisherFantasy Publishing Company, Inc.Publication date1949Media typePrint (hardback)Pages208OCLC52003995 The title novella was reprinted in Two Complete Science-Adventure Books in 1950 The Kingslayer is a collection of science fiction short stories by American writer L. Ron Hubbard. It ...

 

Kygo Life AS

Lifestyle brand This article is an orphan, as no other articles link to it. Please introduce links to this page from related articles; try the Find link tool for suggestions. (March 2018) Kygo Life ASTypeAksjeselskapIndustryAudioFounded2016; 7 years ago (2016)FoundersKyrre Gørvell-DahllJon Inge GullikstadHeadquartersOslo, NorwayProductsHeadphonesEarphonesLoudspeakersOwnerKyrre Gørvell-DahllJon Inge GullikstadWebsitekygolife.com Kygo Life AS is a lifestyle brand founded by ...

Flash (Barry Allen)

Barry AllenBarry Allen, Flash kedua, pada sampul The Flash #30 (Juni 2014). Seni karya Brett Booth.Informasi publikasiPenerbitDC ComicsPenampilan pertamaShowcase #4 (Oktober 1956)Dibuat olehRobert Kanigher Carmine InfantinoInformasi dalam ceritaAlter egoBartholomew Henry Barry AllenSpesiesMetahumanTempat asalCentral CityAfiliasi timJustice LeagueKemitraanFlash (Jay Garrick)Kid Flash (beberapa)Wally WestGreen Lantern (Hal Jordan)Kemampuan Kecepatan super, kelincahan, dan stamina manusia super ...

 

Kenneth Ma

Untuk atlet balap Hong Kong, lihat Kenneth Ma (atlet balap). Ini adalah nama Tionghoa; marganya adalah Ma (馬). Kenneth MaLahir13 Februari 1974 (umur 50)Hong KongTempat tinggalTaikoo CityKebangsaanTemplat:CanAlmamaterUniversity of British ColumbiaPekerjaanAktorTahun aktif1999–sekarangPasanganNancy Wu, Jacqueline WongKarier musikNama lainMa Ming (馬明) Kenneth Ma Hanzi tradisional: 馬國明 Hanzi sederhana: 马国明 Alih aksara Mandarin - Hanyu Pinyin: Mǎ Guómíng Yue (Kanto...

 

Strategi Solo vs Squad di Free Fire: Cara Menang Mudah!