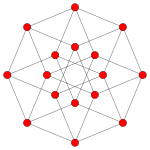
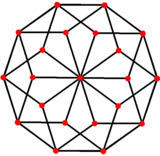
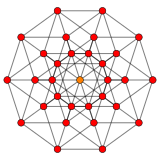
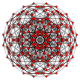
5-cube
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Read other articles:

Australian rules footballer and cricketer This article is about the Australian rules football player. For other people, see Charles Baker (disambiguation). Australian rules footballer Charlie Baker Personal informationFull name Charles Michael BakerDate of birth (1880-06-18)18 June 1880Place of birth Ballarat East, VictoriaDate of death 4 May 1962(1962-05-04) (aged 81)Place of death Ballarat, VictoriaOriginal team(s) St Pats, BallaratHeight 175 cm (5 ft 9 in)Playing c...

Werner-von-Siemens-Gymnasium Weißenburg Haupteingang (von Norden her gesehen) Schulform Gymnasium mit naturwissenschaftlich-technologischem, sprachlichem und wirtschaftswissenschaftlichem Zweig Gründung 1337 / 1932 Adresse An der Hagenau 24 Ort Weißenburg in Bayern Land Bayern Staat Deutschland Koordinaten 49° 1′ 31″ N, 10° 58′ 37″ O49.0254110.97687Koordinaten: 49° 1′ 31″ N, 10° 58′ 37″ O Träger Landkreis Wei�...

En informática, la optimización por nube de partículas u optimización por enjambre de partículas (conocida por sus siglas en inglés: PSO, de «particle swarm optimization») hace referencia a una metaheurística que evoca el comportamiento de las partículas en la naturaleza. Los métodos PSO se atribuyen originalmente a los investigadores Kennedy, Eberhart[1] y Shi.[2] En un principio fueron concebidos para elaborar modelos de conductas sociales,[3] como el movimie...

St. Michael in der Altstadt von Palma Eingangsportal Die Basilika St. Michael (spanisch Basílica de San Miguel) ist eine Kirche in der Altstadt von Palma auf Mallorca in Spanien. Die Kirche im Bistum Mallorca ist dem Erzengel Michael geweiht und trägt den Titel einer Basilica minor.[1] Inhaltsverzeichnis 1 Geschichte 2 Bauwerk 3 Weblinks 4 Einzelnachweise Geschichte San Miquel wurde nach der Reconquista ab 1229 errichtet. Die Kirche soll an der Stelle der großen Moschee von Madina ...

People of British descent in Africa Anglo-African redirects here. For British people of black African heritage, see Black British. For the abolitionist newspaper, see The Anglo-African. For the Lagos newspaper, see The Anglo-African (Lagos). For the term Anglo-African, see Anglo § Africa. British diaspora in AfricaTotal population2–2.5 millionRegions with significant populations South Africa1,600,000[1] Zambia40,000[2] Kenya32,000[3] Zimbabw...

Tudung saji plastik di sebuah rumah di Indonesia Tudung saji merupakan alat dapur yang berfungsi sebagai penutup hidangan atau makanan di atas meja. Kerajinan daerah Tudung saji dapat dibuat dari beragam bahan misalnya bambu, plastik, dedaunan yang dikeringkan atau alumunium. Di berbagai daerah, tudung saji merupakan hasil kerajinan tangan masyarakat setempat. Di Kepulauan Bangka-Belitung, tudung saji bersama dulang adalah lambang dari gotong royong dan tradisi nganggung.[1] Pada masy...

Filipino politician This biography of a living person needs additional citations for verification. Please help by adding reliable sources. Contentious material about living persons that is unsourced or poorly sourced must be removed immediately from the article and its talk page, especially if potentially libelous.Find sources: Antonio Ferrer – news · newspapers · books · scholar · JSTOR (May 2013) (Learn how and when to remove this template message) I...

You can help expand this article with text translated from the corresponding article in Czech. (April 2020) Click [show] for important translation instructions. View a machine-translated version of the Czech article. Machine translation, like DeepL or Google Translate, is a useful starting point for translations, but translators must revise errors as necessary and confirm that the translation is accurate, rather than simply copy-pasting machine-translated text into the English Wikipedia....

2012 American filmHibakushaDirected byChoz BelenSteve NguyenWritten bySteve NguyenIvan TsangProduced bySteve NguyenDean MatsudaBrian L. TanStarringKarin Anna CheungConnie LimDaisuke SuzukiWilliam Frederick KnightJane LuiProductioncompanyStudio APARelease date September 28, 2012 (2012-09-28) Running time45 minutesCountryUnited StatesLanguageEnglish Hibakusha is a 2012 American animated short film directed by Steve Nguyen and Choz Belen, and produced by Iconic Films, the Document...

Sport stadium in Oregon, USAThis article is about Hillsboro Stadium. For the Hillsborough Stadium in Sheffield, England, see Hillsborough Stadium. Hillsboro StadiumMain grandstand in 2009HillsboroLocation in the United StatesShow map of the United StatesHillsboroLocation in OregonShow map of OregonAddress4450 NE Century BoulevardLocationHillsboro, Oregon, U.S.Coordinates45°33′14″N 122°54′25″W / 45.554°N 122.907°W / 45.554; -122.907OwnerCity of HillsboroOper...

Yang Dipertuan Besar (juga dieja sebagai Yang di-Pertuan Besar) adalah gelar bangsawan Melayu. Indonesia Kesultanan Riau-Lingga Di Kesultanan Lingga, Yang Dipertuan Besar adalah gelar lain dari sultan. Kesultanan Asahan Sultan Asahan terakhir (sebelum Republik Indonesia berdiri) memiliki nama lengkap Sri Paduka Tuanku Sultan Sha'ibun 'Abdu'l Jalil Rahmad Shah III ibnu al-Marhum Sultan Muhammad Husain, Sultan dan Yang di-Pertuan Besar Asahan[1] Kesultanan Siak Yang Dipertuan Besar Siak...

Lukas Graham Lukas Graham 2016年基本情報出身地 デンマーク コペンハーゲンジャンル Popsoul活動期間 2011年 -レーベル Copenhagen RecordsWarnerThen We Take the World公式サイト lukasgraham.comメンバー Lukas ForchhammerMark FalgrenMagnus Larsson旧メンバー Anders KirkKasper DaugaardMorten Ristorp ルーカス・グラハム(Lukas Graham)は、デンマークコペンハーゲン出身のソウル・ポップ・バンド。ルーカス・フォ�...

1987 studio album by Regine VelasquezRegineStudio album by Regine VelasquezReleased1987Recorded1986-1987GenrePop, OPMLength30:54LanguageEnglish, TagalogLabelViva RecordsProducerVic del Rosario, Jr. (executive)Ronnie HenaresRegine Velasquez chronology Regine(1987) Nineteen '90(1989) Singles from Regine Isang LahiReleased: 1986 Kung Maibabalik Ko LangReleased: 1987 Maybe Now, Maybe ThenReleased: 1987 Urong SulongReleased: 1988 Regine is the first studio album by Filipino singer-actress ...

Podcast The Majority Report redirects here. For the British Parliamentary report published after the 1905 Royal Commission of the Poor Laws, see Majority report (Poor Law). PodcastThe Majority Report with Sam Seder (2010–present)The Sam Seder Show (2006–2007)The Majority Report with Janeane Garofalo (2004–2006)PresentationHosted bySam Seder (2006–2007, 2010–present)Janeane Garofalo (2004–2006) Co-hosted byEmma Vigeland (2020–present)Matt Lech (2015–present)Bradley Alsop (2021�...

Lexus CT (ZWA10) Общие данные Производитель Lexus (Toyota Motor Corporation) Годы производства 2011—2022 Сборка Миявака, Япония Класс Компактный Дизайн и конструкция Тип кузова 5‑дв. хетчбэк (5‑мест.) Платформа Toyota MC Компоновка переднемоторная, переднеприводная Колёсная формула 4 × 2 Д...

Afghan journalist Shafiqa HabibiShafiqa Habibi talks to Hasht-e Subh Daily, 8 March 2019Born1941 (age 81–82)Kabul, Kingdom of AfghanistanNationalityAfghanOccupation(s)journalist, television anchor, activist and politicianYears active1961 - Shafiqa Habibi is a journalist, television anchor, activist and politician from Afghanistan. She is known for her work to support women journalists, and for her 2004 candidacy for Vice President of Afghanistan as the running mate of Abdul Ra...

Book by Edgar Rice Burroughs The Oakdale Affair and The Rider First book edition of The Oakdale Affair and The RiderAuthorEdgar Rice BurroughsIllustratorJohn Coleman BurroughsCover artistJohn Coleman BurroughsCountryUnited StatesLanguageEnglishGenreNovelPublisherEdgar Rice Burroughs, Inc.Publication date1937Media typePrintPages172 and 144 p. The Oakdale Affair and The Rider is a collection of two short novels by American writer Edgar Rice Burroughs. The Oakdale Affair, a contemporar...

For the EP, see Like Wow (EP). 2001 single by Leslie CarterLike Wow!Single by Leslie Carterfrom the album Shrek: Music from the Original Motion Picture B-sideTrueReleasedJanuary 13, 2001 (2001-01-13) (US)June 12, 2001 (2001-06-12) (Australia)Recorded2000GenrePopLabelDreamWorksSongwriter(s)Jimmy Harry and Sandra St. VictorInternational cover Like Wow! is the debut single by American recording artist Leslie Carter. Her debut album of the same name was shelved, maki...

Bài này viết về nhạc sĩ Huỳnh Anh. Đối với loài hoa, xem Huỳnh anh. Huỳnh AnhThông tin cá nhânSinh(1932-01-02)2 tháng 1, 1932Cần Thơ, Nam Kỳ, Đông Dương thuộc PhápMất13 tháng 12, 2013(2013-12-13) (81 tuổi)San Francisco, California, Hoa KỳGiới tínhnamDân tộcKinhNghề nghiệpNhạc sĩ, Nhạc côngSự nghiệp âm nhạcNhạc cụTrốngCa khúcMưa rừngKiếp cầm caRừng lá thay chưa xts Huỳnh Anh (2 tháng 1 năm 1932 - 13 t...

Private university in Manado, Indonesia This article relies excessively on references to primary sources. Please improve this article by adding secondary or tertiary sources. Find sources: De La Salle Catholic University Manado – news · newspapers · books · scholar · JSTOR (January 2011) (Learn how and when to remove this template message) Universitas Katolik De La Salle ManadoCatholic University of De La Salle ManadoMottoReligio, Mores, Cultura (Latin...