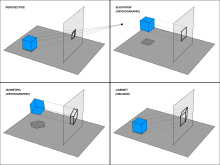
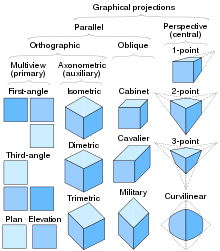
Orthographic projection
|
Read other articles:

Чо́колівкаКиїв площа Космонавтів Загальна інформація 50°25′30″ пн. ш. 30°27′14″ сх. д. / 50.42500° пн. ш. 30.45389° сх. д. / 50.42500; 30.45389Координати: 50°25′30″ пн. ш. 30°27′14″ сх. д. / 50.42500° пн. ш. 30.45389° сх. д. / 50.42500; 30.45389Країна ...

Everett Shinn (L-R), Everett Shinn, Henri Robert y John SloanInformación personalNacimiento 6 de noviembre de 1876 Woodstown, Nueva JerseyFallecimiento 1 de mayo de 1953 (76 años) Nueva YorkNacionalidad EstadounidenseFamiliaCónyuge Florence Scovel Shinn EducaciónEducado en Spring Garden College Alumno de Thomas Pollock Anshutz Información profesionalÁrea PinturaMovimientos Realismo Americano, Arte modernoMiembro de Academia Estadounidense de las Artes y las Letras [editar dato...

Om Основна інформаціяМісце народження Сан-Франциско, СШАРоки активності 2003–до сьогодніЖанр Stoner rock[1] doom metal[2] drone metal[3] Лейбли Drag City, Southern Lord, Holy Mountain, Sub Popomvibratory.com Файли у Вікісховищі Om (іноді пишеться як OM) — американський хеві-метал гурт з Сан-Франциско, К�...

2022 Australian federal election Pre-election pendulum Polling National Electorate Candidates Political parties National results House of Representatives Senate Post-election pendulum State and territory results New South Wales Victoria Queensland Western Australia South Australia Tasmania Australian Capital Territory Northern Territory vte See also: Pre-election pendulum for the 2022 Australian federal election The Australian Labor Party won the 2022 federal election, winning 77 of 151 seats...

Este artículo o sección necesita referencias que aparezcan en una publicación acreditada.Este aviso fue puesto el 14 de marzo de 2020. Muestra preparada para la determinación de la materia seca de la paja de maíz para producir energía. La materia seca o extracto seco es la parte que resta de un material tras extraer toda el agua posible a través de un calentamiento hecho en condiciones de laboratorio. Es una noción usada principalmente en biología y agricultura. El procedimiento cons...

Der Titel dieses Artikels ist mehrdeutig. Siehe auch: Balstad, Ballstedt bzw. Ballenstedt. Ballstädt Landgemeinde Nessetal Wappen von Ballstädt Koordinaten: 51° 2′ N, 10° 43′ O51.03361111111110.716944444444270Koordinaten: 51° 2′ 1″ N, 10° 43′ 1″ O Höhe: 270 m Fläche: 11,86 km² Einwohner: 644 (31. Dez. 2018) Bevölkerungsdichte: 54 Einwohner/km² Eingemeindung: 1. Januar 2019 Postleitzahl: 99869 Vor...

Artikel ini tidak memiliki referensi atau sumber tepercaya sehingga isinya tidak bisa dipastikan. Tolong bantu perbaiki artikel ini dengan menambahkan referensi yang layak. Tulisan tanpa sumber dapat dipertanyakan dan dihapus sewaktu-waktu.Cari sumber: Kasma Booty – berita · surat kabar · buku · cendekiawan · JSTOR Kasma Booty AMN LahirKasmah binti Abdullah1932Kisaran, Keresidenan Sumatera Timur, Hindia Timur Belanda (kini Sumatera Utara, Indonesia)Men...

Michael Kunze (2009) Michael Kunze (* 9. November 1943 in Prag; auch unter dem Pseudonym Stephan Prager bekannt[1]) ist ein deutscher Liedtexter, Schriftsteller, Librettist, Rechtshistoriker, Jurist, und Musikproduzent. Kunze verfasste die Texte von zahlreichen deutschen und internationalen Popmusik-Hits und wurde dafür mit den Grammy und ECHO Lifetime Awards, dem GEMA-Musikautorenpreis sowie 79 Goldenen und Platin-Schallplatten ausgezeichnet. Er ist der Verfasser mehrerer Bücher un...

State park in the U.S. state of Oregon Dabney State Recreation AreaThe Sandy River at Dabney SRA.Show map of OregonShow map of the United StatesTypePublic, stateLocationMultnomah County, OregonNearest cityTroutdaleCoordinates45°31′02″N 122°21′12″W / 45.5173423°N 122.353424°W / 45.5173423; -122.353424[1]Operated byOregon Parks and Recreation Department Dabney State Recreation Area is a park on the Sandy River in the U.S. state of Oregon. Lo...

Louis E. Atkinson Louis Evans Atkinson (* 16. April 1841 in Delaware Township, Juniata County, Pennsylvania; † 5. Februar 1910 in Mifflintown, Pennsylvania) war ein US-amerikanischer Politiker. Zwischen 1883 und 1893 vertrat er den Bundesstaat Pennsylvania im US-Repräsentantenhaus. Werdegang Louis Atkinson besuchte die öffentlichen Schulen seiner Heimat. Nach einem anschließenden Medizinstudium am College of the City of New York und seiner 1861 erfolgten Zulassung als Arzt begann er...

ГородЛибчице-над-ВлтавоуLibčice nad Vltavou Флаг Герб 50°11′57″ с. ш. 14°21′46″ в. д.HGЯO Страна Чехия Край Среднечешский Район Прага-запад Староста Петра Пелешкова История и география Первое упоминание 993[1] Площадь 7,101057 км²[2] Высота центра 207 м Население На�...

Orson Welles in Citizen Kane The sources for Citizen Kane, the 1941 American motion picture that marked the feature film debut of Orson Welles, have been the subject of speculation and controversy since the project's inception. With a story spanning 60 years, the quasi-biographical film examines the life and legacy of Charles Foster Kane, played by Welles, a fictional character based in part upon the American newspaper magnate William Randolph Hearst and Chicago tycoons Samuel Insull and Haro...

1996 studio album by Erik FriedlanderThe WatchmanStudio album by Erik FriedlanderReleasedAugust 20, 1996RecordedJanuary 30, 1996 Seltzer Sound, New York, NYGenreAvant-garde, Jazz, Contemporary classical musicLength56:05LabelTzadik TZ 7107ProducerErik FriedlanderErik Friedlander chronology Chimera(1995) The Watchman(1996) Topaz(1999) The Watchman is a 1996 album by cellist Erik Friedlander which was released on the Tzadik label.[1][2] Reception Professional ratingsRevie...

American new-age music record label This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Narada Productions – news · newspapers · books · scholar · JSTOR (November 2018) (Learn how and when to remove this template message) Narada ProductionsParent companyThe Blue Note Label GroupFounded1983 (1983)FounderJohn...

Abdul Rahim NayyarBornDecember 1883Kapurthala, Punjab, British IndiaDied17 September 1948 (aged 64)Gujranwala, Punjab, PakistanOccupation(s)Muslim Missionary, Religious ScholarKnown forestablishing Ahmadiyya Islam in West Africa Al-Hajj Abdul Rahim Nayyar (December 1883 – September 17, 1948) was a companion of Mirza Ghulam Ahmad and a missionary of the Ahmadiyya Islamic movement in West Africa. He pledged allegiance to Ghulam Ahmad, formally joining the Ahmadiyya movement, in 1901.[...

Jean d'ArrasBiographieDécès MonsActivité ÉcrivainPériode d'activité XIVe siècleAutres informationsA travaillé pour Universités de Douaimodifier - modifier le code - modifier Wikidata Jean d’Arras (actif à la fin du XIVe siècle) est un écrivain français de la fin du XIVe siècle, auteur de la première version écrite, un roman en prose, de la légende de la fée Mélusine, fondatrice de la famille des Lusignan. Cet auteur reste mal connu : il est de l’entourage...

Renang pada Olimpiade Musim Panas 1956LokasiMelbourne Sports and Entertainment CentreMelbourneTanggal29 November – 7 Desember 1956Jumlah disiplin13Peserta235 dari 33 negara← 19521960 → Renang pada Olimpiade Musim Panas 1956 adalah pelaksanaan cabang olahraga renang pada penyelenggaraan Olimpiade Musim Panas 1956. Kompetisi pada cabang olahraga ini berlangsung di Melbourne Sports and Entertainment Centre, Melbourne. Edisi ini menandingkan 13 nomor. 235 at...

For other people named John Meagher, see John Meagher (disambiguation). John William MeagherBorn(1917-12-05)December 5, 1917Jersey City, New Jersey, USDiedApril 14, 1996(1996-04-14) (aged 78)Place of burialArlington National CemeteryAllegianceUnited States of AmericaService/branchUnited States ArmyRankTechnical SergeantUnit2nd Battalion, 305th Infantry Regiment, 77th Infantry DivisionBattles/warsWorld War II Battle of Okinawa AwardsMedal of Honor John William Meagher (December 5, 1917 &#...

جزء من سلسلة مقالات حولحقوق الشباب أنشطة Bailey v. Drexel Furniture Co. Child Labor Deterrence Act قانون حماية خصوصية الأطفال على الإنترنت اتفاقية حقوق الطفل قانون معايير العمل العادل لعام 1938 Hammer v. Dagenhart History of youth rights in the United States Morse v. Frederick Newsboys' strike of 1899 Quebec Charter of Human Rights and Freedoms Wild in the Streets نظريات/مفا�...

2009 studio album by Neon IndianPsychic ChasmsStudio album by Neon IndianReleasedOctober 13, 2009 (2009-10-13)Recorded2009Genre Chillwave[1] pop[2] indie pop[3] electropop[4] Length30:46LabelLefseProducerAlan PalomoNeon Indian chronology Psychic Chasms(2009) The Flaming Lips with Neon Indian(2011) Professional ratingsAggregate scoresSourceRatingMetacritic81/100[5]Review scoresSourceRatingAllMusic[3]The A.V. ClubA[6]...