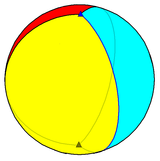
–Ю—Б–Њ–µ–і—А
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Read other articles:

Title in Spanish peerage Dukedom of Palma de MallorcaArms of the Infanta Cristina, Duchess of Palma de MallorcaCreation date26 September 1997CreationFirstCreated byJuan Carlos IPeeragePeerage of SpainFirst holderInfanta CristinaLast holderInfanta CristinaHeir apparentReverted to the Crown.StatusExtinctExtinction date11 June 2015 Duchess of Palma de Mallorca was substantive title in the Peerage of Spain. The title takes its name from the city of Palma de Mallorca, in the Balearic Islands. The ...

LaLa DX Pa√≠s Jap√≥n Idioma Japon√©sCategor√≠a Sh≈Нjo mangaFundaci√≥n 1983 Primera edici√≥n 9 de julio de 1983 √Ъltima edici√≥n A√Їn en publicaci√≥nDesarrolloPublicador HakusenshaCirculaci√≥nFrecuencia bimestral[LaLa Web Oficial P√°gina web oficial][editar datos en Wikidata] LaLa DX es una revista japonesa de manga Sh≈Нjo publicada por Hakusensha. Se public√≥ por primera vez en 1985, y es la hermana de la revista LaLa, revista Sh≈Нjo de la editorial. La revista fue publicada origina...

ЎІўДЎєўДЎІўВЎІЎ™ ЎІўДўЕЎђЎ±ўКЎ© ЎІўДўДЎІЎ™ўБўКЎ© ЎІўДўЕЎђЎ± ўДЎІЎ™ўБўКЎІ ЎІўДўЕЎђЎ± ўДЎІЎ™ўБўКЎІ Ў™ЎєЎѓўКўД ўЕЎµЎѓЎ±ўК - Ў™ЎєЎѓўКўД ЎІўДЎєўДЎІўВЎІЎ™ ЎІўДўЕЎђЎ±ўКЎ© ЎІўДўДЎІЎ™ўБўКЎ© ўЗўК ЎІўДЎєўДЎІўВЎІЎ™ ЎІўДЎЂўЖЎІЎ¶ўКЎ© ЎІўДЎ™ўК Ў™ЎђўЕЎє Ў®ўКўЖ ЎІўДўЕЎђЎ± ўИўДЎІЎ™ўБўКЎІ.[1][2][3][4][5] ўЕўВЎІЎ±ўЖЎ© Ў®ўКўЖ ЎІўДЎ®ўДЎѓўКўЖ ўЗЎ∞ўЗ ўЕўВЎІЎ±ўЖЎ© ЎєЎІўЕЎ© ўИўЕЎ±ЎђЎєўКЎ© ўДўДЎѓўИўДЎ™ўКўЖ: ўИЎђўЗ ЎІўДўЕўВЎІЎ±ўЖЎ© ЎІўДўЕЎђЎ± ўДЎІЎ™ў...

6th Prime Minister of the State of Kuwait For Sheikh Nasser Bin Sabah Al-Ahmad Al-Sabah, see Nasser Sabah Al-Ahmad Al-Sabah. His Highness SheikhNasser Al-Mohammed Ahmad Al-Jaber Al-SabahЎІўДЎіўКЎЃ ўЖЎІЎµЎ± ЎІўДўЕЎ≠ўЕЎѓ ЎІўДЎ£Ў≠ўЕЎѓ ЎІўДЎђЎІЎ®Ў± ЎІўДЎµЎ®ЎІЎ≠6th Prime Minister of KuwaitIn office7 February 2006 вАУ 28 November 2011MonarchSabah Al-Ahmad Al-Jaber Al-SabahDeputyJaber Al-Mubarak Al-Hamad Al-SabahPreceded bySabah Al-Ahmad Al-Jaber Al-SabahSucceeded byJaber Al-Mubarak Al-...

1994 greatest hits album by Kenny GThe Very Best of Kenny GGreatest hits album by Kenny GReleasedMay 9, 1994GenreJazzLength64:43LabelAristaKenny G chronology Miracles: The Holiday Album(1994) The Very Best of Kenny G(1994) The Moment(1996) The Very Best of Kenny G is the third greatest hits album by saxophonist Kenny G. It was released by Arista Records in 1994. Track listing Forever in Love - 4:57 Waiting for You - 4:59 By the Time This Night Is Over - 4:46 Jasmine Flower - 4:36 Them...

Libel trial in England in 1792 Thomas Paine, the defendant The trial of Thomas Paine for seditious libel was held on 18 December 1792 in response to his publication of the second part of the Rights of Man. The government of William Pitt, worried by the possibility that the French Revolution might spread to England, had begun suppressing works that espoused radical philosophies. Paine's work, which advocated the right of the people to overthrow their government, was duly targeted, with a writ ...

1976 single by Bee Gees You Should Be DancingSingle by Bee Geesfrom the album Children of the World B-sideSubwayReleasedJune 1976 (1976-06)[1]Recorded19 JanuaryвАУ1, 8 February 19766 May 1976StudioCriteria (Miami)Le Studio (Morin Heights, Quebec)GenreDisco[1][2]Length4:16 (single)4:47 (12 version)LabelRSOSongwriter(s)Barry GibbRobin GibbMaurice GibbProducer(s)Bee Gees, Albhy Galuten, Karl RichardsonBee Gees singles chronology Fanny (Be Tender with My Love) (1...

Municipality in Gelderland, Netherlands This article is about the municipality in the Netherlands. For a community in Suriname, see Wageningen, Suriname. Municipality in Gelderland, NetherlandsWageningenMunicipalityWageningen market square FlagCoat of armsMotto: City of Life SciencesLocation in GelderlandWageningenLocation within the NetherlandsShow map of NetherlandsWageningenLocation within EuropeShow map of EuropeCoordinates: 51¬∞58вА≤N 5¬∞40вА≤E / 51.967¬∞N 5.667¬∞E&#...

ж≠§жҐЭзЫЃйЬАи¶Би°•еЕЕжЫіе§ЪжЭ•жЇРгАВ (2023еєі1жЬИ12жЧ•)иѓЈеНПеК©и£ЬеЕЕе§ЪжЦєйЭҐеПѓйЭ†жЭ•жЇРдї•жФєеЦДињЩзѓЗжЭ°зЫЃпЉМжЧ†ж≥ХжЯ•иѓБзЪДеЖЕеЃєеПѓиГљжЬГеЫ†зВЇеЉВиЃЃжПРеЗЇиАМ襀粿йЩ§гАВиЗідљњзФ®иАЕпЉЪиѓЈжРЬ糥дЄАдЄЛжЭ°зЫЃзЪДж†ЗйҐШпЉИжЭ•жЇРжРЬ糥пЉЪиТЩеП§еЬЛдљЫжХЩ — зљСй°µгАБжЦ∞йЧїгАБдє¶з±НгАБе≠¶жЬѓгАБеЫЊеГПпЉЙпЉМдї•ж£АжЯ•зљСзїЬдЄКжШѓеР¶е≠ШеЬ®иѓ•дЄїйҐШзЪДжЫіе§ЪеПѓйЭ†жЭ•жЇРпЉИеИ§еЃЪжМЗеЉХпЉЙгАВ ж≠§жҐЭзЫЃзњїи≠ѓеУБи≥™з®НжЬЙдЄНиґ≥гАВиЂЛеНФеК©зњїи≠ѓжЬђжҐЭзЫЃжИЦйЗНжЦ∞зЈ®еѓЂпЉМеєґж≥®жДПйБњеЕНзњїиѓСиЕФ...

ўДўЕЎєЎІўЖўН Ў£ЎЃЎ±ўЙЎМ ЎЈЎІўДЎє ЎІўДўЖЎІЎ≠ўКЎ© ЎІўДўЕЎ±ўГЎ≤ўКЎ© (Ў™ўИЎґўКЎ≠). ЎІўДўЖЎІЎ≠ўКЎ© ЎІўДўЕЎ±ўГЎ≤ўКЎ© ЎІўДЎ•Ў≠ЎѓЎІЎЂўКЎІЎ™ 36¬∞10вА≤40вА≥N 49¬∞08вА≤30вА≥E / 36.177777777778¬∞N 49.141666666667¬∞E / 36.177777777778; 49.141666666667 Ў™ўВЎ≥ўКўЕ Ў•ЎѓЎІЎ±ўК ЎІўДЎ®ўДЎѓ Ў•ўКЎ±ЎІўЖ ЎІўДЎ™ўВЎ≥ўКўЕ ЎІўДЎ£ЎєўДўЙ ўЕўВЎІЎЈЎєЎ© Ў£Ў®ўЗЎ±[1] ЎєЎѓЎѓ ЎІўДЎ≥ўГЎІўЖ ЎєЎѓЎѓ ЎІўДЎ≥ўГЎІўЖ 151528 (2016)[1] ЎєЎѓЎѓ ЎІў...

Film school of Concordia University in Montreal, Canada This article relies excessively on references to primary sources. Please improve this article by adding secondary or tertiary sources. Find sources: Mel Hoppenheim School of Cinema вАУ news ¬Ј newspapers ¬Ј books ¬Ј scholar ¬Ј JSTOR (January 2010) (Learn how and when to remove this template message) Mel Hoppenheim School of CinemaThe Faubourg Building, which houses the MHSoC at Concordia UniversityAddre...

Defunct outlaw motorcycle club from Quebec, Canada This article uses bare URLs, which are uninformative and vulnerable to link rot. Please consider converting them to full citations to ensure the article remains verifiable and maintains a consistent citation style. Several templates and tools are available to assist in formatting, such as reFill (documentation) and Citation bot (documentation). (September 2022) (Learn how and when to remove this template message) Popeye MCPatch logo of the Po...

Airport in Vernon, British ColumbiaVernon Regional AirportIATA: YVEICAO: CYVKSummaryAirport typePublicOwnerCity of VernonOperatorVernon Regional Airport CorporationLocationOkanagan Landing, Vernon, British ColumbiaTime zonePST (UTCвИТ08:00) вАҐ Summer (DST)PDT (UTCвИТ07:00)Elevation AMSL1,141 ft / 348 mCoordinates50¬∞14вА≤46вА≥N 119¬∞19вА≤51вА≥W / 50.24611¬∞N 119.33083¬∞W / 50.24611; -119.33083WebsiteOfficial websiteMapCYVKLocation in British C...

Asamblea General de las Naciones UnidasЎІўДЎђўЕЎєўКЎ© ЎІўДЎєЎІўЕЎ© ўДўДЎ£ўЕўЕ ЎІўДўЕЎ™Ў≠ЎѓЎ©иБФеРИеЫље§ІдЉЪAssembl√©e g√©n√©rale des Nations uniesUnited Nations General Assembly–У–µ–љ–µ—А–∞–ї—М–љ–∞—П –∞—Б—Б–∞–Љ–±–ї–µ—П –Ю–Ю–Э 76¬∞ per√≠odo Sal√≥n de sesionesInformaci√≥n general√Бmbito Organizaci√≥n de las Naciones UnidasCreaci√≥n 1946Atribuciones Art√≠culo IV de la Carta de las Naciones UnidasTipo UnicameralLiderazgoPresidente Csaba K≈Сr√ґsi Composici√≥nMiembros 193 Estados miembros2 Estados ...

2008 Hong Kong filmLa LingerieTheatrical posterDirected byChan Hing-KaiJanet ChunWritten byChan Hing-KaiJanet ChunProduced byChan Hing-KaiAmy ChinStarringStephy TangJanice ManJJ JiaKathy YuenRonald ChengCinematographyWong Wing-HungEdited byCheung Ka-KitMusic byChiu Tsang-HeiProductioncompaniesIcon PicturesOne Hundred Years of Film Co. Ltd.Distributed byChina Star Entertainment GroupRelease date 8 August 2008 (2008-08-08) Running time118 minutesCountryHong KongLanguageCantoneseB...

Zé Caipora, de Angelo Agostini. La historieta o cómic brasileño tiene su origen en el siglo XIX, con la irrupción en la prensa de las tiras de estilo satírico. Recién a comienzos del siglo XX se publicarán las primeras revistas dedicadas específicamente a las historietas. A pesar de contar con reconocidos artistas (Maurício de Sousa, Mike Deodato, Flávio Colin, Glauco, Laerte, Ziraldo), y que algunos de ellos alcanzaran trascendencia internacional trabajando para el exteri...

Species of gastropod This taxon may be invalid (unaccepted). Redirect to the correct taxon or request deletion by adding a {{subst:prod|concern=TEXT}} tag. See this article's talk page for more information. (Jan 2019) Calliostoma punctulatum Scientific classification Kingdom: Animalia Phylum: Mollusca Class: Gastropoda (unranked): clade Vetigastropoda Family: Calliostomatidae Genus: Calliostoma Species: C. punctulatum Binomial name Calliostoma punctulatum(Martyn, 1784) Synonyms[1...
Paghimo ni bot Lsjbot. 38¬∞58вА≤21вА≥N 38¬∞30вА≤22вА≥E / 38.9725¬∞N 38.50611¬∞E / 38.9725; 38.50611 √Цvledik Ge√Іidi Gintang Nasod Turkeya Lalawigan Malatya Gitas-on 1,405 m (4,610 ft) Tiganos 38¬∞58вА≤21вА≥N 38¬∞30вА≤22вА≥E / 38.9725¬∞N 38.50611¬∞E / 38.9725; 38.50611 Timezone EET (UTC+2) - summer (DST) EEST (UTC+3) GeoNames 446450 Gintang ang √Цvledik Ge√Іidi sa Turkeya.[1] Nahimutang ni sa lalawigan sa Malatya...

No ApologiesAlbum studio karya Leony VHDirilis1 Februari 2004GenrePop, R&B, rock, soul, teen popLabelSongCity MusicKronologi Leony VH Lie Lie An Taw Taw (2000)String Module Error: Match not foundString Module Error: Match not found No Apologies (2004) -String Module Error: Match not foundString Module Error: Match not found No Apologies merupakan sebuah album musik milik penyanyi sekaligus mantan personel Trio Kwek-Kwek, Leony VH yang dirilis pada tahun 2004. Lagu yang dijagokan adala...

American prelate His Excellency, The Most ReverendGregory Lawrence ParkesJCLBishop of St. PetersburgChurchCatholic ChurchArchdioceseMiamiDioceseSt. PetersburgAppointedNovember 28, 2016InstalledJanuary 4, 2017PredecessorRobert Nugent LynchOrdersOrdinationJune 26, 1999by Norbert Dorsey, C.P.ConsecrationJune 5, 2012by Thomas Gerard Wenski, John Gerard Noonan, and Felipe de Jes√Їs Est√©vezPersonal detailsBorn (1964-04-02) April 2, 1964 (age 60)Mineola, New York, USPrevious post(s)B...