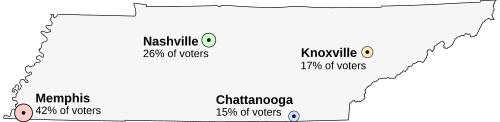
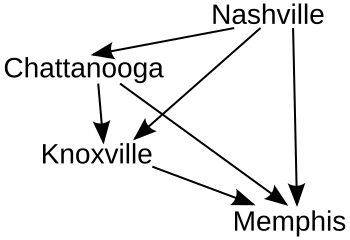
Ranked pairs
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Read other articles:

SanggrahanDesaKantor Desa SanggrahanNegara IndonesiaProvinsiJawa TengahKabupatenSukoharjoKecamatanGrogolKode pos57552Kode Kemendagri33.11.09.2011 Luas184,3535km²Jumlah penduduk8807 jiwaKepadatan29jiwa/km² Sanggrahan adalah desa di kecamatan Grogol, Sukoharjo, Jawa Tengah, Indonesia. Pembagian wilayah Desa Sanggrahan terdiri dari beberapa dukuh, antara lain: Dukuh Badongan Dukuh Badan Dukuh Dukuh Dukuh Sanggrahan Dukuh Mantung Dukuh Sidorejo Dukuh Ciptonegaran Dukuh Karang Dukuh Kaliwin...

Knight's Cross recipientsAllgradesGrand CrossGolden Oak Leaves, Swordsand DiamondsOak Leaves, Swords and DiamondsOak Leaves and SwordsOakLeaves 1940–41 1942 1943 1944 1945 Foreign Knight'sCross A Ba–Bm Bn–Bz C D E F G Ha–Hm Hn–Hz I J Ka–Km Kn–Kz L M N O P Q R Sa–Schr Schu–Sz T U V W X–Z Foreign Knight's Cross The Knight's Cross of the Iron Cross (German: Ritterkreuz des Eisernen Kreuzes) and its variants were the highest awards in the military and paramilitary forces...

معركة حصن بورتوفيق إستسلام القائد الإسرائيلي لنقطة بورتوفيق الحصينة بعد الموقعة 1973 معلومات عامة التاريخ 6 أكتوبر، 1973 الموقع سيناء، مصر النتيجة انتصار مصري وأستيلائها علي النقطة - أستسلام القوة الإسرائيلية المتحاربون مصر إسرائيل القادة صلاح عبد الحليمعلي المزاحي جا

Der Titel dieses Artikels ist mehrdeutig. Weitere Bedeutungen sind unter Xenophon (Begriffsklärung) aufgeführt. Xenophon Sokrates (rechts) und möglicherweise Xenophon (links). Detail aus der Schule von Athen von Raffael, 1510–1511 Xenophon (altgriechisch Ξενοφῶν Xenophṓn; * zwischen 430 und 425 v. Chr. in Athen; † ca. 354 v. Chr. in Korinth[1]) war ein antiker griechischer Politiker, Feldherr und Schriftsteller in den Bereichen Geschichte, Ökonomie und Philosophie. E...

Parts of this article (those related to 20-plus years old population data) need to be updated. Please help update this article to reflect recent events or newly available information. (April 2021) This article is part of a series on thePolitics of Germany Constitution (Basic Law) Federal Constitutional Court Human rights Head of State President of Germany Frank-Walter Steinmeier (SPD) Executive Chancellor of Germany (list) Olaf Scholz (SPD) Vice Chancellor of Germany Robert Habeck (Grüne) Ca...

2000 first-person shooter video game This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Counter-Strike video game – news · newspapers · books · scholar ·...

Municipality of Montenegro Municipality in MontenegroNikšić Municipality Opština NikšićОпштина НикшићMunicipality Coat of armsNikšić Municipality in MontenegroCountryMontenegroSeatNikšićArea • Total2,065 km2 (797 sq mi)Population • Total72,443 • Density35/km2 (91/sq mi)Postal code81400Area code+382 40Car platesNKISO 3166-2 codeME-12Websitewww.niksic.me Nikšić Municipality (Montenegrin and Serbian: Opšti...

2017 sports competition 2017 Central American GamesHost cityManaguaCountryNicaraguaTeams7OpeningDecember 3, 2017 (2017-12-03)ClosingDecember 17, 2017 (2017-12-17)Opened byDaniel OrtegaMain venueDennis Martínez National StadiumWebsiteOfficial website← 2013 San Jose2022 Santa Tecla → The 2017 Central American Games, the XI edition of the Central American Games, were hosted in Managua, Nicaragua during 3–17 December 2017.[1] Sports &...

Single woman who owns many pet cats Crazy cat lady redirects here. For the Simpsons character, see List of recurring The Simpsons characters § Crazy Cat Lady. For the syndrome, see Crazy cat lady syndrome. For the video game, see The Cat Lady. Not to be confused with Catgirl or Catwoman. A woman feeding cats in Rome A cat lady is a cultural archetype or stock character, most often depicted as a white woman, a middle-aged or elderly spinster or widow, who has many cats. The term may be p...

هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (مارس 2022) أرام هاكوبيان معلومات شخصية الميلاد 15 أغسطس 1979 (العمر 44 سنة)يريفان الطول 1.75 م (5 قدم 9 بوصة) مركز اللعب مهاجم الجنسية أرمينيا معلومات النادي الناد...

Japanese voice actress (1937–2021) Masako Sugaya菅谷政子Born(1937-08-21)August 21, 1937Tokyo, JapanDiedFebruary 25, 2021(2021-02-25) (aged 83)OccupationVoice actressAgentArts Vision Masako Sugaya (菅谷政子, Sugaya Masako) (August 21, 1937 – February 25, 2021) was a Japanese voice actress who has worked primarily in anime. She died on February 25, 2021.[1][2] Filmography Anime Television Perman (1967), Ganko[3] Under Sea Boy Marine (1969), Kurikuri Umeb...

Elections in Oregon Federal government Presidential elections 1860 1864 1868 1872 1876 1880 1884 1888 1892 1896 1900 1904 1908 1912 1916 1920 1924 1928 1932 1936 1940 1944 1948 1952 1956 1960 1964 1968 1972 1976 1980 1984 1988 1992 1996 2000 2004 2008 2012 2016 2020 2024 Presidential primaries Democratic 2000 2004 2008 2016 2020 2024 Republican 2008 2012 2016 2020 2024 U.S. Senate elections 1859 1860 sp 1862 sp 1864 1870 1872 1882 1885 1885 sp 1888 1890 1895 1898 1898 sp 1901 1903 1907 1907 s...

2022 psychological thriller film Nocebo (The Curse)Theatrical release posterDirected byLorcan FinneganWritten byGarret ShanleyProduced by Bianca Balbuena Brunella Cocchiglia Maxime Cottray Cloé Garbay David Gilbery Patti Lapus Emily Leo Bradley Liew Mary McCarthy Bastien Sirodot Marlon Vogelgesang Starring Eva Green Mark Strong Chai Fonacier Billie Gadsdon Cathy Belton Anthony Falcon Cinematography Jakub Kijowski Radek Ladczuk Edited byTony CranstounMusic byJose BuencaminoProductioncompanies...

Kampung Adat Praijing Kampung Adat Praijing adalah sebuah kampung yang terletak di Desa Tebara, Waikabubak, Kabupaten Sumba Barat, Provinsi Nusa Tenggara Timur.[1][2][3] Kampung ini terletak sekitar 3 km dari pusat Kota Waikabubak.[2] Kampung ini dikenal sebagai kampung wisata yang memiliki 38 rumah tradisional Sumba. Sebelumnya, di kampung ini terdapat 42 rumah tradisional, tetapi kini hanya 38 yang tersisa akibat terjadinya kebakaran pada tahun 2000.[...

2015 Chinese documentary film Taste of China味道中国Directed byHuang YinghaoZhang WeiWang BingJin YingProductioncompaniesDocuChina.Co.LtdSMG PicturesRelease date January 23, 2015 (2015-01-23) Running time86 minutesCountryChinaLanguageMandarinBox officeCN¥150,000 Taste of China (Chinese: 味道中国) is a 2015 Chinese documentary film directed by Huang Yinghao, Zhang Wei, Wang Bing and Jin Ying. It was released on January 23, 2015.[1] Cast Yang Zhenhua Chen Han...

American civil servant T. Coleman AndrewsCommissioner of Internal RevenueIn officeFebruary 4, 1953 – October 31, 1955PresidentDwight D. EisenhowerPreceded byJustin F. Winkle (acting)Succeeded byO. Gordon Delk (acting) Personal detailsBornThomas Coleman Andrews(1899-02-19)February 19, 1899Richmond, Virginia, USDiedOctober 15, 1983(1983-10-15) (aged 84)Richmond, Virginia, USPolitical partyRepublicanState's Rights Party Thomas Coleman Andrews (February 19, 1899 – October 15, 19...

Sri Lankan politician Hon.Champika PremadasaMPMember of Parliamentfor Kegalle DistrictIncumbentAssumed office 2000 Personal detailsBorn (1948-11-04) November 4, 1948 (age 75)NationalitySri LankanPolitical partyUnited National PartyResidence(s)55/6 Water Tank Road, Rambukkana Abathenna Devayalage Champika Premadasa (born November 4, 1948) is a Sri Lankan politician and a member of the Parliament of Sri Lanka. References A.D. CHAMPIKA PREMADASA. Directory of Members. Parliament of Sri ...

Untuk pengertian lain, lihat Scarsdale. Scarsdale adalah sebuah kota di Westchester County, New York, Amerika Serikat. Scarsdale berpenduduk 17.823 jiwa menurut sensus tahun 2000. Geografi Menurut United States Census Bureau (Biro Sensus Amerika Serikat), kota ini mencakup wiayah seluas 17,2 km². Lingkungan Edgewood Fox Meadow Greenacres Heathcote Quaker Ridge Lingkungan yang di luar desa Scarsdale namun masih memiliki kode ZIP 10583 (milik Scarsdale): Beech Hill (Kota Yonkers) Edgemont (Des...

Former Director of Business Development at Cambridge Analytica Brittany KaiserBrittany Kaiser in November 2019 during Web Summit 2019 in Lisbon, Portugal.BornBrittany Nicole Kaiser (1987-11-06) 6 November 1987 (age 36)[1][2]Houston, Texas, U.S. Brittany Nicole Kaiser (born November 6, 1987)[1][2] is the former business development director for Cambridge Analytica, which collapsed after details of its misuse of Facebook data became public. Cambridge Analyti...

1943 Italian filmA Little WifeDirected byGiorgio BianchiWritten byZoltán Nagyiványi (novel) Giorgio Bianchi Sandro De Feo Vincenzo TalaricoStarringFosco Giachetti Assia Noris Clara CalamaiCinematographyAldo TontiEdited byLeo CatozzoMusic bySalvatore AllegraProductioncompanyGrandi FilmDistributed byGrandi FilmRelease date22 December 1943Running time76 minutesCountryItalyLanguageItalian A Little Wife (Italian: Una piccola moglie) is a 1943 Italian white-telephones drama film directed by Giorg...