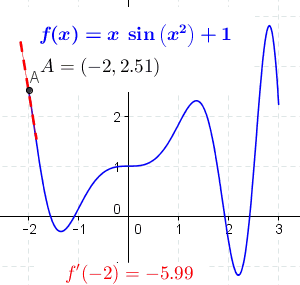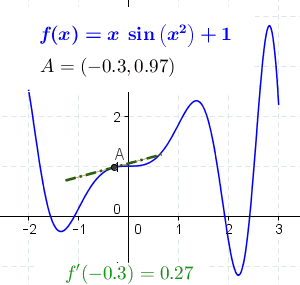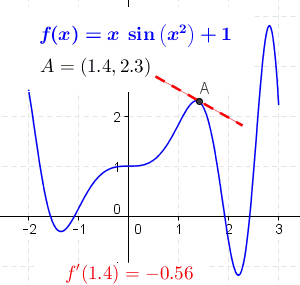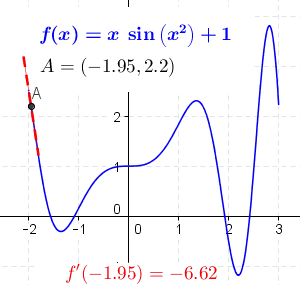
Slope
|
Read other articles:

Đừng nhầm lẫn với Dominica. Cộng hòa Dominica Tên bằng ngôn ngữ chính thức República Dominicana (tiếng Tây Ban Nha) Quốc kỳ Huy hiệu Bản đồ Vị trí của Cộng hoà Dominica Tiêu ngữDios, Patria, Libertad(tiếng Tây Ban Nha: Thiên Chúa, Quê hương, Tự do)Quốc ca¡Quisqueyanos Valientes!'¡Valiant Quisqueyans!Hành chínhChính phủDân chủTổng thốngLuis AbinaderThủ đôSanto Domingo18°30′N 69°59′W18°30′B 69°59′T ...

Het mondstuk van een trompetHet mondstuk is het deel van een (blaas)muziekinstrument waar de toon gevormd wordt door het laten trillen van lippen of het (enkel/dubbel) riet. De rest van het muziekinstrument zorgt voornamelijk voor versterking van het geluid en de verandering in toonhoogte. Soorten mondstukken Er zijn verschillende soorten mondstukken. Komvormig metalen mondstuk Op veel koperblaasinstrumenten (trompet, trombone, tuba) staat een komvormig metalen mondstuk, waartegen de lippen m...
Маскування — термін, який має кілька значень. Ця сторінка значень містить посилання на статті про кожне з них.Якщо ви потрапили сюди за внутрішнім посиланням, будь ласка, поверніться та виправте його так, щоб воно вказувало безпосередньо на потрібну статтю.@ пошук посила�...

Der Titel dieses Artikels ist mehrdeutig. Zum auch Ahmadiyya genannten Sufi-Orden siehe Badawiyya. Mirza Ghulam Ahmad, Begründer der Ahmadiyya Die Ahmadiyya (Urdu احمدیہ ‚Ahmad-tum‘) ist eine islamische Gemeinschaft, die von Mirza Ghulam Ahmad in den 1880er Jahren in Britisch-Indien gegründet wurde. Ab 1889 leisteten ihm die Anhänger den Treueid. Sie ließen sich 1901 unter dem Namen Ahmadiyya Musalmans in die offiziellen Zensuslisten der britisch-indischen Verwaltung ein...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Januari 2023. Bandar Udara BajuraIATA: BJUICAO: VNBR Bandar Udara BajuraLokasi bandar udara di NepalInformasiJenisPublikMelayaniBajura, NepalKetinggian dpl1,311 mdplKoordinat29°30′N 81°40′E / 29.500°N 81.667°E / 29.500; 81.6...

Дозвіл на використання цієї роботи зберігається в архівах системи VRT. Його ідентифікаційний номер 2014020810007752.Якщо вам потрібне підтвердження, зв'яжіться з кимось із користувачів, що мають доступ до системи.

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada November 2022. CaptiveTheatrical release posterSutradara Jerry Jameson Produser Lucas Akoskin Terry Botwick Alex Garcia David Oyelowo Ken Wales Katrina Wolfe Ditulis oleh Brian Bird SkenarioBrian BirdPemeran David Oyelowo Kate Mara Leonor Varela Jessica Oyelowo Mimi...

Grafik fungsi dengan asimtot horizontal (y = 0), vertikal (x = 0), dan miring (garis ungu, diberikan oleh y = 2x) Kurva yang memotong suatu asimtot berkali-kali sebanyak takhingga Dalam geometri analitis, asimtot dari sebuah kurva adalah sebuah garis yang sedemikian rupa sehingga jarak antara kurva dan garis tersebut mendekati nol seiring x atau y (salah satu atau keduanya) mendekati takhingga. Beberapa sumber menyertakan persyaratan bahwa kurva mungkin tidak mel...

Portuguese footballer (born 1987) In this Portuguese name, the first or maternal family name is Vilela and the second or paternal family name is Gama. Bruno Gama Gama in action for Dnipro in 2015Personal informationFull name Bruno Alexandre Vilela Gama[1]Date of birth (1987-11-15) 15 November 1987 (age 36)[1]Place of birth Vila Verde, Portugal[1]Height 1.75 m (5 ft 9 in)[1]Position(s) WingerTeam informationCurrent team AEK LarnacaNumber ...

Si ce bandeau n'est plus pertinent, retirez-le. Cliquez ici pour en savoir plus. Cet article est orphelin. Moins de trois articles lui sont liés (février 2022). Vous pouvez aider en ajoutant des liens vers [[Journée de la résistance à l'occupation de la république autonome de Crimée et de la ville de Sébastopol]] dans les articles relatifs au sujet. Logo de la fête La journée de la résistance à l'occupation de la république autonome de Crimée et de la ville de Sébastopol (e...

1709 Ukraina Upptäckt[1]UpptäckareGrigorij SjajnUpptäcktsplatsSimeiz-observatorietUpptäcktsdatum16 augusti 1925BeteckningarMPC-beteckning(1709) UkrainaAlternativnamn1925 QA, 1936 QH[1]Uppkallad efterUkraina (land)[2]SmåplanetskategoriAsteroidbältetOmloppsbana[3]Epok: 21 januari 2022Aphelium2,887 AUPerihelium1,870 AUHalv storaxel2,378 AUExcentricitet0,2136949Siderisk omloppstid3,67 årMedelomloppshastighet19,31 km/sMedelanomali92,73°Inklination7,556°Longitud för uppstigande nod30...

Compact executive car manufactured by Opel Chevrolet Vectra redirects here. For the version of the car sold in Brazil from 2009 to 2011, see Opel Astra. Motor vehicle Opel VectraOpel Vectra C (facelift)OverviewManufacturerOpel (General Motors)Also calledVauxhall Cavalier (1988–1995)Vauxhall Vectra (1995–2008)Holden VectraChevrolet VectraProductionOctober 1988 – June 2010Body and chassisClassLarge family car (D-segment)LayoutFront-engine, front-wheel-drive/four-wheel-driveChronology...

Dave BautistaLahir18 Januari 1969 (umur 54)Washington D.C., Amerika Serikat[1]Tempat tinggalLos Angeles, California, Amerika Serikat[1]Situs webdemon-wrestling.comKarier gulat profesionalNama ringBatista[2]Dave Batista[3]Deacon Batista[1]Leviathan[1]Kahn / KhanThe Animal[1]Tinggi198 cm (6 ft 6 in) [2]Berat290 pon (130 kg)[2]Asal dariWashington D.C.[2]Dilatih olehAfa Anoaʻi[4]...

Medical conditionApparent mineralocorticoid excess syndromeOther namesAME, 11-beta-hydroxysteroid dehydrogenase deficiency type 2, Ulick syndrome.Apparent mineralocorticoid excess syndrome has an autosomal recessive pattern of inheritanceSpecialtyMedical genetics, endocrinology SymptomsHypertension, hypokalemia, metabolic alkalosis, and low plasma renin activity.[1] Apparent mineralocorticoid excess is an autosomal recessive[2] disorder causing hypertension (high blood pr...

Papiro 35Manoscritto del Nuovo TestamentoSimbolo p {\displaystyle {\mathfrak {p}}} 35 TestoVangelo secondo Matteo 7; 15 † DatazioneIV secolo Scritturagreco ConservazioneBiblioteca Medicea Laurenziana Dimensione26 x 15 cm Tipo testualealessandrino CategoriaI Il Papiro 35 ( p {\displaystyle {\mathfrak {p}}} 35) è uno dei più antichi manoscritti esistenti del Nuovo Testamento, datato paleograficamente agli inizi del IV secolo.[1] È scritto in greco. Indice 1 Contenuto del papiro 2 N...

Street in Kowloon, Hong Kong For Boundary Street in Washington, D.C., see Florida Avenue. 22°19′35.71″N 114°10′4.40″E / 22.3265861°N 114.1678889°E / 22.3265861; 114.1678889 This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Boundary Street – news · newspapers · books · scholar ...

Virgen de los Reyes Católicos, de maestro anónimo, 1490. Hispano flamenco es un término con el que la historiografía[1] designa la estrecha relación entre la cultura y el arte del espacio conocido impropiamente como Flandes (en realidad el Estado borgoñón) y la Monarquía Hispánica de la época de los Reyes Católicos (1469-1516), aunque realmente la relación se produjo especialmente con la Corona de Castilla y se extendió durante todo el Antiguo Régimen.[2] La relaci...

Berikut adalah daftar negara dan teritori yang sebelumnya dikuasai oleh Britania Raya atau merupakan bagian dari Imperium Britania disertai hari kemerdekaannya: Negara Tanggal Tahun Catatan Afghanistan 08-19 19 Agustus 1919 Antigua dan Barbuda 11-01 1 November 1981 Australia 01-26 26 Januari 1788 Bahama 07-10 10 Juli 1973 Bahrain 12-16 16 Desember 1971 15 Agustus 1971 Barbados 11-30 30 November 1966 Undang-Undang Kemerdekaan Barbados 1966 Belize 09-21...

Territorial authority district in Northland, New ZealandWhangarei DistrictTerritorial authority districtMt Lion at the Whangarei Heads as viewed from Mt ManaiaCountryNew ZealandRegionNorthlandDistrictWhangarei District CouncilWardsMāoriBream BayHikurangi-CoastalMangakahia-MaungatapereWhāngarei HeadsWhangārei UrbanLocal hapūNgāti HineNgāti WaiPatuharakekeTe ParawhauSeatWhangāreiGovernment • MayorVince Cocurullo[1] • Deputy MayorPhil Halse [2] ...

Railway station in the Cynon Valley, Wales AbercynonArriva Trains Wales Class 150 Sprinter at the station in May 2016General informationLocationAbercynon, Rhondda Cynon TafWalesCoordinates51°38′42″N 3°19′37″W / 51.645°N 3.327°W / 51.645; -3.327Grid referenceST082948Managed byTransport for WalesPlatforms2Other informationStation codeACYClassificationDfT category F1Key dates9 October 1840Station opens as Navigation House6 August 1846Renamed Aberdare Junction1...