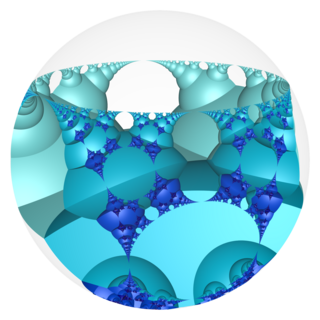
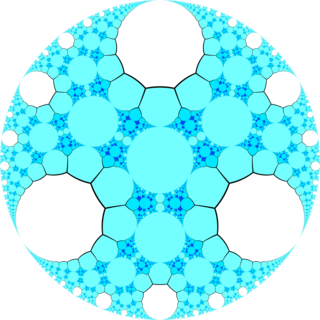
Order-infinite-3 triangular honeycomb
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Read other articles:

–õ—Ć–≤—Ė–≤—Ā—Ć–ļ–į –ĺ–Ī–Ľ–į—Ā—ā—Ć –ď–Ķ—Ä–Ī –õ—Ć–≤—Ė–≤—Ā—Ć–ļ–ĺ—ó –ĺ–Ī–Ľ–į—Ā—ā—Ė –ü—Ä–į–Ņ–ĺ—Ä –õ—Ć–≤—Ė–≤—Ā—Ć–ļ–ĺ—ó –ĺ–Ī–Ľ–į—Ā—ā—Ė –ě—Ā–Ĺ–ĺ–≤–Ĺ—Ė –ī–į–Ĺ—Ė –ü—Ä—Ė–∑–≤–ł—Ā—Ć–ļ–ĺ: –ď–į–Ľ–ł—á–ł–Ĺ–į, –õ—Ć–≤—Ė–≤—Č–ł–Ĺ–į –ö—Ä–į—ó–Ĺ–į: –£–ļ—Ä–į—ó–Ĺ–į –£—ā–≤–ĺ—Ä–Ķ–Ĺ–į: 4 –≥—Ä—É–ī–Ĺ—Ź 1939 —Ä–ĺ–ļ—É –ö–ĺ–ī –ö–ź–Ę–ě–Ę–Ę–ď: UA46000000000026241 –Ě–į—Ā–Ķ–Ľ–Ķ–Ĺ–Ĺ—Ź: 2 478 133 –ü–Ľ–ĺ—Č–į: 21 831.97 –ļ–ľ¬≤ –ď—É—Ā—ā–ĺ—ā–į –Ĺ–į—Ā–Ķ–Ľ–Ķ–Ĺ–Ĺ—Ź: 113,42 –ĺ—Ā—Ė–Ī/–ļ–ľ¬≤ –ü–ĺ—ą—ā–ĺ–≤—Ė —Ė–Ĺ–ī–Ķ–ļ—Ā–ł 79000...

ŔÖōßŔÜō≤ōßŔÜŔäŔĄŔą (ō®ōßŔĄō•ō≥ō®ōßŔÜŔäō©: Manzanillo)‚ÄŹ ŔÖōßŔÜō≤ōßŔÜŔäŔĄŔą (ōßŔĄŔÖŔÉō≥ŔäŔÉ) ō™ōßōĪŔäōģ ōßŔĄō™ō£ō≥Ŕäō≥ 24 ŔäŔąŔĄŔäŔą 1527[1] ō™Ŕāō≥ŔäŔÖ ō•ōĮōßōĪŔä ōßŔĄō®ŔĄōĮ ōßŔĄŔÖŔÉō≥ŔäŔÉ [2] ōĻōßōĶŔÖō© ŔĄŔÄ Manzanillo Municipality, Colima [ōßŔĄō•ŔÜō¨ŔĄŔäō≤Ŕäō©] ōģōĶōßō¶ōĶ ō¨ōļōĪōßŔĀŔäō© ō•ō≠ōĮōßōęŔäōßō™ 19¬į03‚Ä≤12‚Ä≥N 104¬į18‚Ä≤59‚Ä≥W / 19.053205833333¬įN 104.31638055556¬įW / 19.053205833333; -104.316380555...

West-east street in Manhattan, New York 79th StreetThe Harry F. Sinclair House at 2 East 79th StreetMaintained byNYCDOTLength2.4 mi (3.9 km)[1]Width100 feet (30.48 m)LocationManhattanPostal code10024 (west), 10075 (east)Coordinates40¬į46‚Ä≤36‚Ä≥N 73¬į57‚Ä≤48‚Ä≥W / 40.7768¬įN 73.9632¬įW / 40.7768; -73.9632West end NY 9A / Henry Hudson Parkway in Riverside ParkEast end FDR Drive in YorkvilleNorth80th StreetSouth78th StreetConstr...

For the Martina McBride song, see There You Are (Martina McBride song). 1990 single by Goo Goo DollsThere You AreSingle by Goo Goo Dollsfrom the album Hold Me Up Released1990Recorded1990GenreAlternative Rock[1]Length3:40LabelMetal Blade RecordsSongwriter(s)John Rzeznik, Robby Takac, George TutuskaGoo Goo Dolls singles chronology There You Are (1990) I'm Awake Now (1991) Music videoThere You Are on YouTube There You Are is the debut single by the Goo Goo Dolls. It was the trio's first ...

ŔáōįŔá Ŕąōßō≠ōĮō© ŔÖŔÜ ō≥ŔĄō≥ŔĄō© ŔÖŔāōßŔĄōßō™ ō≠ŔąŔĄōßŔĄō£ō≥ōßō∑ŔäōĪ ō≠ō≥ō® ōßŔĄō≠ō∂ōßōĪō© ōĻōĪō®Ŕäō© ō£ōĪŔÖŔÜŔäō© ōßŔĄōĘō≤ō™ŔäŔÉ ŔāŔĄō∑Ŕäō© ŔąŔäŔĄō≤Ŕäō© Ŕáō®ōĪōĮŔäō≥Ŕäō© ŔÖō≥Ŕäō≠Ŕäō© ōĶŔäŔÜŔäō© ŔÖōĶōĪŔäō© ō•ōļōĪŔäŔāŔäō© ōļŔąōßōĪōßŔÜŔäō© ŔáŔÜōĮŔąō≥Ŕäō© ō•ō≥ŔĄōßŔÖŔäō© Ŕäōßō®ōßŔÜŔäō© ŔäŔáŔąōĮŔäō© ŔÉŔąōĪŔäō© ōßŔĄŔÖōßŔäōß ō®ŔĄōßōĮ ōßŔĄōĪōßŔĀōĮŔäŔÜ ŔÖŔäŔÉōĪŔąŔÜŔäō≥Ŕäō© ō•ō≥ŔÉŔÜōĮŔÜōßŔĀŔäō© ŔĀōßōĪō≥Ŕäō© ō®ŔąŔĄŔąŔÜŔäō≤Ŕäō© ōĪŔąŔÖōßŔÜŔäō© ŔáŔąō®Ŕäō© ŔĀŔĄŔÉŔĄŔąōĪ ōĪŔąŔÖōßŔÜŔä ō≥ŔĄōßŔĀŔäō© ō™ōĪŔÉŔäō© ō£

British linguist (1926‚Äď2001) This article relies largely or entirely on a single source. Relevant discussion may be found on the talk page. Please help improve this article by introducing citations to additional sources.Find sources: David Neil MacKenzie ‚Äď news ¬∑ newspapers ¬∑ books ¬∑ scholar ¬∑ JSTOR (April 2013) David Neil MacKenzieBorn(1926-04-08)8 April 1926London, England, UKDied13 October 2001(2001-10-13) (aged 75)Bangor, Wales, UKOccupation...

Frederick Corbin BlesseNickname(s)BootsBorn(1921-08-22)August 22, 1921Col√≥n, Panama Canal ZoneDiedOctober 31, 2012(2012-10-31) (aged 91)Melbourne, FloridaBuriedArlington National CemeteryAllegianceUnited StatesService/branchUnited States Air ForceYears of service1945‚Äď1975RankMajor GeneralUnit413th Fighter Group18th Fighter-Bomber Group4th Fighter-Interceptor Wing366th Tactical Fighter WingCommands held32nd Fighter Interceptor Squadron474th Tactical Fighter Wing831st Air DivisionB...

Species of fish Cyprinodon nevadensis California Conservation status Vulnerable (IUCN 3.1)[1] Imperiled (NatureServe)[2] Scientific classification Domain: Eukaryota Kingdom: Animalia Phylum: Chordata Class: Actinopterygii Order: Cyprinodontiformes Family: Cyprinodontidae Genus: Cyprinodon Species: C. nevadensis Binomial name Cyprinodon nevadensis(C. H. Eigenmann & R. S. Eigenmann, 1889) Subspecies C. n. nevadensis C. n. amargosae C. n. mionectes C. n. pector...

2011 Chinese filmThe Dragon KnightPosterśīõŚÖčÁéčŚõĹšĻčŚú£ťĺôť™ĎŚ£ęDirected byShengjun YuProductioncompaniesShenzhen Tencent Computer SystemsUYONG MediaToonmax MediaBeijing Ironhide Frog Creativity Media[1]Release date September 30, 2011 (2011-09-30) Running time90 minutesCountryChinaLanguageMandarinBox officeCN¬•27.3 million The Dragon Knight (Chinese: śīõŚÖčÁéčŚõĹšĻčŚú£ťĺôť™ĎŚ£ę) is a 2011 Chinese animated film directed by Shengjun Yu. The film was released on Sep...

Television channel TV3 SPORTBroadcast areaDenmarkOwnershipOwnerViaplay GroupSister channelsTV3, TV3+, TV3 Puls, TV3 MAX, SeeHistoryLaunched7 January 2013; 10 years ago (2013-01-07)ReplacedSportskanalenFormer namesTV3 Sport 1LinksWebsitetv3sport.dkAvailabilityTerrestrialBoxer- TV3 Sport is a Danish sports television channel, owned by Viaplay Group and operated by TV3 SPORT. The channel originally broadcast as TV 2 SPORT and was a joint-venture between TV 2 and Modern Times Gr...

Do 28 Skyservant Dornier Do-28D Skyservant Role STOL light utility aircraftType of aircraft Manufacturer Dornier Flugzeugbau GmbH First flight 29 April 1959 (Do 28 A/B)23 February 1966 (Do 28D) Status In civilian and military use Primary user German Air Force Number built *Do 28 - 1 Do 28A-1 - 60[1] Do 28B-1 - 60[1] Do 28D-1 - 54 Do 28D-2 - 172 Dornier 128-6 - 6 Developed from Dornier Do 27 Developed into Dornier 228 The type designation Dornier Do 28 comprises two differ...

Overview of the European maritime exploration of Australia This article is part of a series on theHistory of AustraliaReplica ship of the Duyfken, the first European ship to reach Australia Timeline and periods Prehistory European exploration (sea) European exploration (land) 1788‚Äď1850 1851‚Äď1900 1901‚Äď1945 1945‚Äďpresent Topics Abortion Agriculture Antisemitism Banking Capital punishment Civil rights Cinema Constitution Diplomacy Economics Federation Immigration Labour LGBT Military Mona...

–ě–Ņ–ł—Ā –¶—é —Ą–ĺ—ā–ĺ–≥—Ä–į—Ą—Ė—é –Ī—É–Ľ–ĺ –∑—Ä–ĺ–Ī–Ľ–Ķ–Ĺ–ĺ –≤ —Ä–į–ľ–ļ–į—Ö –í—Ė–ļ—Ė–Ķ–ļ—Ā–Ņ–Ķ–ī–ł—Ü—Ė—ó –ö–ł—ó–≤ ‚ÄĒ –ě–Ī—É—Ö—Ė–≤ ‚ÄĒ –ď—Ä–Ķ–Ī—Ė–Ĺ–ļ–ł. –ö–ĺ–Ņ–į—á—Ė–≤—Ā—Ć–ļ–į —Ā—Ė–Ľ—Ć—Ā—Ć–ļ–į —Ä–į–ī–į, –ö–ĺ–Ņ–į—á—Ė–≤, –ě–Ī—É—Ö—Ė–≤—Ā—Ć–ļ–ł–Ļ —Ä–į–Ļ–ĺ–Ĺ –Ē–∂–Ķ—Ä–Ķ–Ľ–ĺ –≤–Ľ–į—Ā–Ĺ–į —Ä–ĺ–Ī–ĺ—ā–į –ß–į—Ā —Ā—ā–≤–ĺ—Ä–Ķ–Ĺ–Ĺ—Ź 24.07.2011 –ź–≤—ā–ĺ—Ä –∑–ĺ–Ī—Ä–į–∂–Ķ–Ĺ–Ĺ—Ź Amakuha –õ—Ė—Ü–Ķ–Ĺ–∑—Ė—Ź –ī–ł–≤. –Ĺ–ł–∂—á–Ķ –£–≤–į–≥–į: —Ü–Ķ –∑–ĺ–Ī—Ä–į–∂–Ķ–Ĺ–Ĺ—Ź –Ĺ–Ķ –ľ–ĺ–∂–Ķ –Ī—É—ā–ł –∑–į–≤–į–Ĺ—ā–į–∂–Ķ–Ĺ–Ķ –ī–ĺ ...

Heritage-listed building in Sydney, Australia Haymarket Post OfficeHaymarket Post Office, 633‚Äď635 George Street, SydneyLocation633‚Äď635 George Street, Sydney, City of Sydney, New South Wales, AustraliaCoordinates33¬į52‚Ä≤42‚Ä≥S 151¬į12‚Ä≤19‚Ä≥E / 33.8784¬įS 151.2052¬įE / -33.8784; 151.2052Built1927‚Äď1928ArchitectE. Henderson New South Wales Heritage RegisterOfficial nameKing George Hotel (former) and Haymarket Post OfficeTypestate heritage (complex / group)Designat...

ŚłēŚįĒśĚúś≤≥ÁēĒŚú£ŚÖčť≤ĀśĖĮSanta Cruz do Rio PardoŚłāťēጳēŚįĒśĚúś≤≥ÁēĒŚú£ŚÖčť≤ĀśĖĮŚú®Ś∑īŤ•ŅÁöĄšĹćÁĹģŚĚźś†áÔľö22¬į53‚Ä≤56‚Ä≥S 49¬į37‚Ä≤58‚Ä≥W / 22.8989¬įS 49.6328¬įW / -22.8989; -49.6328ŚõĹŚģ∂Ś∑īŤ•ŅŚ∑ěŚú£šŅĚÁĹóŚ∑ěťĚĘÁßĮ ‚ÄĘ śÄĽŤģ°1,116.377 ŚĻ≥śĖĻŚÖ¨ťáĆÔľą431.036 ŚĻ≥śĖĻŤčĪťáĆԾȜĶ∑śčĒ467 ŚÖ¨ŚįļÔľą1,532 ŤčĪŚįļԾȚļļŚŹ£Ôľą2009ÔľČ ‚ÄĘ ÁłĹŤ®ą43,483šļļ ‚ÄĘ ŚĮÜŚļ¶39šļļ/ŚĻ≥śĖĻŚÖ¨ťáĆÔľą101šļļ/ŚĻ≥śĖĻŤčĪťáĆÔľČ ŚłēŚįĒśĚúś≤≥ÁēĒŚú£ŚÖ...

SMA Negeri 11 Tangerang SelatanInformasiDidirikan26 Juni 2006JenisNegeriAkreditasiAKepala SekolahDrs. RodaniJurusan atau peminatanIPA dan IPSRentang kelasX, XI IPA, XI IPS, XII IPA, XII IPSKurikulumKurikulum Tingkat Satuan PendidikanAlamatLokasiJl. Sumatra 1, Rawa Lele, Jombang, Ciputat, Tangerang Selatan, Banten, IndonesiaTel./Faks.(021) 98292460MotoMotoSPORTIF SMA Negeri 11 Tangerang Selatan, merupakan salah satu Sekolah Menengah Atas Negeri yang ada di Provinsi Banten, Indonesia. Sama...

East German coxswain Dietmar DomnickDomnick (top) in 1957SportSportRowingClubASK Vorw√§rts Berlin Medal record Men's rowing Representing East Germany European Rowing Championships 1957 Duisburg Coxed four Dietmar Domnick is a retired East German coxswain who won the 1957 European Rowing Championships title in coxed four, together with Lothar Wundratsch, Gerhard M√ľller, Egon Meyer and Heinz Dathe.[1] The men rowed for ASK Vorw√§rts Berlin.[2] It was the first time that E...

2 Raja-raja 1Kitab Raja-raja (Kitab 1 & 2 Raja-raja) lengkap pada Kodeks Leningrad, dibuat tahun 1008.KitabKitab 2 Raja-rajaKategoriNevi'imBagian Alkitab KristenPerjanjian LamaUrutan dalamKitab Kristen12← 1 Raja-raja 22 pasal 2 → 2 Raja-raja 1 (atau II Raja-raja 1, disingkat 2Raj 1) adalah pasal pertama Kitab 2 Raja-raja dalam Alkitab Ibrani dan Perjanjian Lama di Alkitab Kristen. Dalam Alkitab Ibrani termasuk Nabi-nabi Awal atau Nevi'im Rishonim [◊†◊Ď◊ô◊ź◊ô◊Ě ◊®◊ź◊©◊ē◊†◊ô◊Ě] ...

Surrealist text The Solar Anus AuthorGeorges BatailleOriginal titleL'anus solaireIllustratorAndr√© MassonLanguageFrenchPublisher√Čditions de la Galerie SimonPublication date1931OCLC8937274 The Solar Anus (French: L'anus solaire) is a short surrealist text by the French writer Georges Bataille, written in 1927 and published with drawings by Andr√© Masson in 1931.[1] Albeit elliptically, its aphorisms refer to decay, death, vegetation, natural disasters, impotence, frustration, enn...

Nippon Ishin no Kai Parteivorsitz (daihyŇć) Nobuyuki Baba Parteivorsitzender Stellvertretender Vorsitz kyŇćdŇć-daihyŇć:Hirofumi Yoshimurafuku-daihyŇć:Junko Tsuji[1] Generalsekret√§r Fumitake Fujita[1] Exekutivratsvorsitz Hirofumi Yanagase[1] PARC-Vorsitz Shun Otokita[1] Gr√ľndung November 2015 Hauptsitz 1-7-16 Shimanouchi, ChŇęŇć, Osaka, Osaka Mitglieder 14.457 (2016)[2] Farbe(n) Gr√ľn Abgeordnete im ShŇęgiin 41/465(April 2023)...