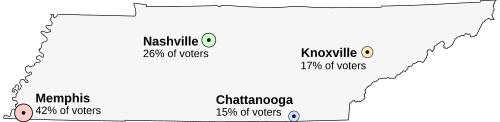
Copeland's method
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Read other articles:

Untuk kegunaan lain, lihat Kembali (disambiguasi). KembaliAlbum studio karya GarasiDirilisJuli 2011GenreAlternative RockLabelTrinity Optima ProductionMiles MusicKronologi Garasi Garasi II (2008)Garasi II2008 Kembali(2011) Kembali adalah album musik ketiga karya Garasi yang dirilis tahun 2011. Berisi 8 buah lagu dengan Hidup Hanya Sekali sebagai lagu utama album ini. Dalam album ini, Garasi menampilkan formasi baru, dengan Higin Ayuga sebagai vokalis (yang menggantikan Ayu Ratna) dan Wembr...

Monarch AirlinesBerkas:MonarchAirlinesNew.svg IATA ICAO Kode panggil ZB MON MONARCH Didirikan1967Berhenti beroperasi2017PenghubungBandar Udara Internasional BirminghamBandar Udara London LutonBandar Udara London GatwickBandara Internasional ManchesterProgram penumpang setiaVantage ClubArmada31Tujuan20Kantor pusatLuton, England, Britania RayaTokoh utamaBill Hodgson, Don Peacock, Alan Snudden, CBESitus webMonarch Airlines Monarch Airlines Boeing 757-200 in the old livery, Alicante Airport, Spai...

Зникний спрей — речовина, що застосовується у спортивних змаганнях, переважно в футболі, щоб намалювати тимчасову лінію для користування гравцями та суддею з метою забезпечення чесної гри. Спрей наноситься з аерозольного балону та виглядає як біла пляма або лінія, як...

?Лемінги Lemmus lemmus Біологічна класифікація Домен: Еукаріоти (Eukaryota) Царство: Тварини (Animalia) Тип: Хордові (Chordata) Клас: Ссавці (Mammalia) Ряд: Гризуни (Rodentia) Родина: Хом'якові (Cricetidae) Підродина: Щурові (Arvicolinae) Триба: Lemmini Роди Dicrostonyx лемінг (Lemmus) Болотяні лемінги (Synaptomys) Myopus Посилання Вікіс

Oskar Schindler Untuk kegunaan lain, lihat Schindler dan Schindler. Oskar Schindler (28 April 1908 – 9 Oktober 1974) adalah seorang pengusaha yang terkenal karena upaya-upayanya untuk menyelamatkan buruh-buruh Yahudinya dari Holocaust. Ia menyelamatkan hingga 1.200 orang Yahudi dengan menyuruh mereka bekerja di pabrik amunisinya di daerah yang kini menjadi wilayah Polandia. Schindler dengan sengaja memproduksi amunisi yang buruk dalam upayanya merugikan militer Jerman dalam peperangan. Masa...

Das Generalkommando 51 war ein Großverband der Armee des Deutschen Kaiserreiches im Ersten Weltkrieg. Inhaltsverzeichnis 1 Gliederung 2 Geschichte 3 Kommandierender General 4 Einzelnachweise Gliederung Es handelte sich beim Generalkommando 51 um ein Generalkommando z. b. V. (Generalkommando zur besonderen Verwendung). Diese entstanden ab 1916 und waren reine Kommandostellen, die militärischen Einheiten wurden ihr nach Bedarf zugeordnet. Geschichte Albert von Berrer Eberhard von Ho...

Romanian folk song Map of the region of Bukovina, divided between Romania and Ukraine Cântă cucu-n Bucovina or Cântă cucu în Bucovina (transl. Sings the Cuckoo in Bukovina) is a Romanian folk song, more precisely a doină, composed in 1904 by Constantin Mandicevschi [de; ru; uk]. The lyrics are original, while the melody is a modified Bukovinian mourning song. Mandicevschi composed it at the request of Spiru Haret for the 400th anniversary of the death of Prince of Mol...

Roti lapis bakon, telur dan kejuRoti lapis bakon, telur dan keju yang terbukaJenisRoti lapis sarapanBahan utamaRoti, bakon, telur, keju Roti lapis bakon, telur dan keju adalah menu sarapan roti lapis populer di Amerika Serikat dan Kanada. Makanan ini terbuat dari bakon, telur (biasanya digoreng atau diorak-arik), keju dan roti, bisa juga diolesi mentega dan dipanggang. Banyak jenis roti lapis yang menggunakan produk alternatif bakon atau menggunakan berbagai jenis keju dan roti.[1] Va...

StarterPublication informationPublisherDupuisFormatBande DessinéeGenreFranco-BelgePublication date1952Main character(s)StarterPipetteCreative teamCreated byAndré FranquinJidéhemWritten byJidéhemArtist(s)Jidéhem Starter is a Franco-Belgian comics series created by André Franquin (drawings) and Jidéhem (drawings and scripts) in Spirou. History It originated in from a similarly titled periodical column about automobiles, written by Jacques Wauters in Spirou from 1952 on.[1] In 195...

United States Article I court United States Army Court of Criminal Appeals(A.C.C.A.)LocationFort Belvoir(Fairfax County, Virginia)Appeals toCourt of Appeals for the Armed ForcesEstablished1968AuthorityArticle I tribunalCreated byUniform Code of Military Justicewww.jagcnet.army.mil/ACCA In the United States military, the Army Court of Criminal Appeals (ACCA) is an appellate court that reviews certain court martial convictions of Army personnel. Jurisdiction In the United States, cour...

American swimmer Cierra RungeRunge in 2014Personal informationNational team United StatesBorn (1996-03-07) March 7, 1996 (age 27)Cochranville, Pennsylvania, U.S.Height6 ft 2.5 in (189 cm)Weight187 lb (85 kg)SportSportSwimmingStrokesFreestyleClubNorth Baltimore Aquatic ClubCollege teamUniversity of California, Berkeley (2014–2015)University of Wisconsin–Madison (2016–2017)Arizona State University (2018–2020)CoachBob Bowman Medal record Women's sw...

American actress (1893–1985) Leatrice JoyJoy c. 1920BornLeatrice Johanna Zeidler(1893-11-07)November 7, 1893New Orleans, Louisiana, U.S.DiedMay 13, 1985(1985-05-13) (aged 91)New York City, U.S.Resting placeSaint Savior Episcopal ChurchyardOther namesBeatrice JoyOccupationActressYears active1915–1954Spouses John Gilbert (m. 1922; div. 1925) William S. Hook (m. 1931; div. 1944)...

This article relies excessively on references to primary sources. Please improve this article by adding secondary or tertiary sources. Find sources: Bank Asya – news · newspapers · books · scholar · JSTOR (August 2011) (Learn how and when to remove this template message) Bank AsyaTypeParticipation BankIndustryFinancial servicesFoundedOctober 24th, 1996DefunctJuly 22, 2016 (2016-07-22)HeadquartersIstanbul, TurkeyKey peopleİsmail GÜLER -...

Original author(s)Walt Disney StudiosHome EntertainmentOperating systemiOS, Web browserAvailable inEnglishTypeEntertainmentWebsitedisneysecondscreen.com Disney Second Screen was an interactive application, released on a computer (via Flash) or iPad (as native app). This app provides onscreen film feature accessible download that provided additional content, and user can views a film released by the Walt Disney Studios Home Entertainment.[1][2] The movie linked with the viewer'...

British musicologist (1888–1959) Eric BlomBlom photographed by Howard Coster in 1942Born(1888-08-20)20 August 1888Bern, SwitzerlandDied11 April 1959(1959-04-11) (aged 70)NationalitySwiss and British Eric Walter Blom CBE (20 August 1888 – 11 April 1959) was a Swiss-born British-naturalised[1] music lexicographer, music critic and writer. He is best known as the editor of the 5th edition of Grove's Dictionary of Music and Musicians (1954). Early life Blom was born ...

الحزب الليبرالي الكندي (بالإنجليزية: Liberal Party of Canada)، (بالفرنسية: Parti libéral du Canada) البلد كندا التأسيس تاريخ التأسيس 1 تموز 1867 المؤسسون جورج براون قائد الحزب جاستن ترودو (14 أبريل 2013–) القادة جاستن ترودو المقرات المقر الرئيسي 81 شارع متكاف، أوتوا الأفكار الأيديول�...

Wasserstraßen- und Schifffahrtsamt Trier(WSA Trier) Staatliche Ebene Bund Stellung Unterbehörde Aufsichtsbehörde Generaldirektion Wasserstraßen und Schifffahrt Gründung 1952 Auflösung 13. Juni 2019 Nachfolger Wasserstraßen- und Schifffahrtsamt Mosel-Saar-Lahn Hauptsitz Trier Das Wasserstraßen- und Schifffahrtsamt Trier (WSA Trier) war ein Wasserstraßen- und Schifffahrtsamt in Deutschland. Es gehörte zum Dienstbereich der Generaldirektion Wasserstraßen und Schifffahrt, vormals Wasse...

Jundiaí Estação JundiaíPlataforma da estação Uso atual Estação de trens metropolitanos Proprietário Governo do Estado de São Paulo Administração SPR (1867-1946)EFSJ (1948-1969)RFFSA (1975–1984) CBTU (1984–1994) CPTM (1994–atualmente) Linhas Rubi Expresso Turístico Sigla JUN Movimento em 2014 14,2 mil Serviços Informações históricas Inauguração 16 de fevereiro de 1867 (156 anos) Localização Localização Estação Jundiaí Endereço Av. União dos Ferroviár...

Dinguiraye Prefectura Coordenadas 11°18′03″N 10°43′05″O / 11.300833333333, -10.718055555556Entidad Prefectura • País Guinea • Región FaranahSuperficie • Total 14 500 km² [editar datos en Wikidata] Dinguiraye es una prefectura de la región de Faranah de Guinea, con una población censada en marzo de 2014 de 196 469 habitantes. Está formada por las siguientes subprefecturas, que igualmente se muestran...

2016 studio album by Watskyx InfinityStudio album by WatskyReleasedAugust 19, 2016 (2016-08-19)Recorded Seahorse Studios, Los Angeles, California Grand St. Recording, Brooklyn, New York Aqua Vibea, Hadley, Massachusetts GenreAlternative hip hopLength 65:42 (standard version) 75:22 (deluxe version) LabelSteel Wool Media, EmpireProducer Watsky Kush Mody Russell Simmons Anderson Paak Frans Mernick Mikos Da Gawd Daniel Riera Wax Andrew Oedel Watsky chronology All You Can Do...