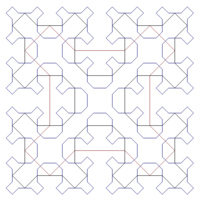
Sierpiński curve
|
Read other articles:

Artikel ini perlu diwikifikasi agar memenuhi standar kualitas Wikipedia. Anda dapat memberikan bantuan berupa penambahan pranala dalam, atau dengan merapikan tata letak dari artikel ini. Untuk keterangan lebih lanjut, klik [tampil] di bagian kanan. Mengganti markah HTML dengan markah wiki bila dimungkinkan. Tambahkan pranala wiki. Bila dirasa perlu, buatlah pautan ke artikel wiki lainnya dengan cara menambahkan [[ dan ]] pada kata yang bersangkutan (lihat WP:LINK untuk keterangan lebih lanjut...

У этого термина существуют и другие значения, см. Беатриче д’Эсте. Беатриса д'Эсте королева Венгрии 1234 — 1235 Предшественник Иоланда де Куртене Преемник Мария Ласкарина Рождение 1215(1215)Феррара, герцогство Феррара Смерть после 8 мая 1245 Род Арпады Отец Альдобрандино I д'Эст...

Kim Seok-jin김석진Jin saat pada 1 Mei 2019.Informasi latar belakangNama lahirKim Seok-jinLahir4 Desember 1992 (umur 30)Gwacheon, Gyeonggi-do, Korea SelatanGenreK-popPop-rockBaladaPekerjaanPenyanyiPenulis laguInstrumenVokalTahun aktif2013 (2013)–sekarangLabel Big Hit Artis terkaitBTSNama KoreaHangul김석진 Hanja金碩珍 Alih AksaraGim Seok-jinMcCune–ReischauerKim Sŏk-chin Kim Seok-jin (bahasa Korea: 김석진, lahir 4 Desember 1992) atau lebih dikenal dengan mononimnya...

Sports season2014 CFL seasonDurationJune 26 – November 8, 2014East championsHamilton Tiger-CatsWest championsCalgary Stampeders102nd Grey CupDateNovember 30, 2014VenueBC Place, VancouverChampionsCalgary Stampeders CFL seasons← 20132015 → LionsStampedersElksRoughridersBlue BombersArgonautsTiger-CatsAlouettesRedblacksclass=notpageimage| Locations of the active CFL teams West East The 2014 CFL season was the 61st season of modern-day Canadian football. It was the 57th Canadi...

دينيسي إيمرسون معلومات شخصية الميلاد 13 مايو 1960 (63 سنة) الجنسية أستراليا الحياة العملية الفرق منتخب أستراليا للكريكت (1984–1987) المهنة لاعبة كريكت الرياضة الكريكت بلد الرياضة أستراليا تعديل مصدري - تعديل دينيسي إيمرسون (13 مايو 1960 - ) (بالإنجليزية: Denise Emerson)

Artikel ini tidak memiliki referensi atau sumber tepercaya sehingga isinya tidak bisa dipastikan. Tolong bantu perbaiki artikel ini dengan menambahkan referensi yang layak. Tulisan tanpa sumber dapat dipertanyakan dan dihapus sewaktu-waktu.Cari sumber: Skala waktu nuklir – berita · surat kabar · buku · cendekiawan · JSTOR Di dalam astrofisika, skala waktu nuklir adalah skala waktu yang dihabiskan oleh sebuah bintang dalam membakar bahan bakar nuklirnya...

Нуристан Координати 38°29′21″ пн. ш. 65°38′47″ сх. д. / 38.48916666669477848° пн. ш. 65.64638888891678903° сх. д. / 38.48916666669477848; 65.64638888891678903Координати: 38°29′21″ пн. ш. 65°38′47″ сх. д. / 38.48916666669477848° пн. ш. 65.64638888891678903° сх. д. / 38.48916666669477848; 65.64...

Penciptaan Terang, karya Gustave Doré Kegelapan, adalah kebalikan langsung dari kebercahayaan, didefinisikan sebagai kurangnya penerangan, tidak adanya cahaya tampak, atau permukaan yang menyerap cahaya, seperti hitam atau coklat. Penglihatan manusia tidak dapat membedakan warna dalam kondisi pencahayaan yang sangat rendah. Sel-sel fotoreseptor sensitif warna pada retina tidak aktif ketika tingkat cahaya tidak mencukupi, dalam kisaran persepsi visual yang disebut sebagai penglihatan skotopik...

Este artículo o sección necesita referencias que aparezcan en una publicación acreditada.Este aviso fue puesto el 28 de diciembre de 2014. Norþanhymbra(Norðimbraland) Reino de Northumbria Heptarquía anglosajona ← ← ← 653-954 → → → Ubicación de {{{nombre_común}}}Reino de Northumbria, c. 700 Capital Norte: Bamburgh (Bebbanburg)Sur: York (Eoferwic) Idioma oficial Northumbrio (dialecto del inglés antiguo), latín Otros idiomas Britónico, cúmbrico Gobierno Monarquía Rey de ...

لمعانٍ أخرى، طالع الرفد (توضيح). قرية الرفد - قرية - تقسيم إداري البلد اليمن المحافظة محافظة أبين المديرية مديرية رصد العزلة عزلة القارة السكان التعداد السكاني 2004 السكان 30 • الذكور 17 • الإناث 13 • عدد الأسر 4 • عدد المساكن 6 معلومات أخرى ا�...

Political party in Australia Centre Party Centre Reform GroupAbbreviationCPLeaderEric CampbellFounderEric CampbellFounded4 December 1933Dissolvedc. 1935HeadquartersSydney, New South WalesNewspaper • New Guard • LibertyArmed wingNew GuardMembership (1933)c. 15,000IdeologyAustralian fascism[1] • Militarism • Monarchism[2] • Anti-communism[2] • Antisemitism[3] R...

Hiệp định thương mại tự do EU EU có hiệu lực áp dụng tạm thời ký nhưng chưa áp dụng thỏa thuận nhưng chưa ký đang đàm phán Liên minh châu Âu ký kết các hiệp định thương mại tự do (FTA) [1] và các thoả thuận khác có một phần về thương mại với nhiều nước trên thế giới và đang đàm phán với nhiều nước khác.[2] ...

French jurist and political philosopher (c. 1530–1596) Jean BodinBornc. 1530Angers, Maine-et-Loire, FranceDied1596Laon, Aisne, FranceEraRenaissance philosophyRegionWestern philosophySchoolMercantilismMain interestsLegal philosophy, political philosophy, economyNotable ideasQuantity theory of money, absolute sovereignty Jean Bodin (French: [ʒɑ̃ bɔdɛ̃]; c. 1530 – 1596) was a French jurist and political philosopher, member of the Parlement of Paris and professor of ...

British actress (1938-2014) Topsy Jane as Audrey in The Loneliness of the Long Distance Runner (1962) Topsy Jane (2 December 1938 – 4 January 2014) was a British actress of the 1960s. She was in The Loneliness of the Long Distance Runner (1962) and was cast as Liz (the role eventually played by Julie Christie) in the 1963 film Billy Liar but was forced to pull out owing to mental health issues.[1][2][3][4] Early life Topsy Jane made an early appearance at...

Radio station in Puerto PrincesaBrigada News FM Palawan (DWYO)Puerto PrincesaBroadcast areaPalawanBranding103.1 Brigada News FMProgrammingLanguage(s)FilipinoFormatContemporary MOR, News, TalkNetworkBrigada News FMOwnershipOwnerBrigada Mass Media Corporation(Baycomms Broadcasting Corporation)HistoryFirst air date1993 (as Bay Radio) May 8, 2014 (as Brigada News FM)Technical informationLicensing authorityNTCPower10,000 wattsRepeater(s)Coron: 101.3 MHzBrooke's Point: 95.7 MHzNarra: 96.5 MHzQuezon...

This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Medan Independent School – news · newspapers · books · scholar · JSTOR (December 2022) (Learn how and when to remove this template message) Private international school in Medan, Sumatra, IndonesiaMedan Independent SchoolAddressJalan Tali Air No.5 / Jamin Ginti...

Консульский диптих Флавия Руфия Геннадия Проба Ореста Флавий Руфий Геннадий Проб Орест (лат. Rufius Gennadius Probus Orestes) — римский консул в 530 году на Западе. Со-консулом на Востоке был Флавий Лампадий. В следующие 531—533 годы сохранялся их пост-консулат. Вероятно, он был сын�...

2012 video game 2012 video gameDeadlightXbox Live Arcade cover artDeveloper(s)Tequila WorksPublisher(s)Microsoft StudiosDeep Silver (Director's Cut)Director(s)Raúl Rubio MunárrizProducer(s)Amaia Markuleta ArrulaIsaac Barrón HermanDesigner(s)Raúl Rubio MunárrizArtist(s)César Sampedro GuerraWriter(s)Antonio RojanoComposer(s)David García DíazEngineUnreal Engine 3[4]Platform(s)Xbox 360, Microsoft Windows, PlayStation 4, Xbox OneReleaseXbox 360WW: August 1, 2012[1]WindowsWW...

For the Britney Spears song, see Gimme More. This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Give Me More – news · newspapers · books · scholar · JSTOR (October 2018) (Learn how and when to remove this template message) 2012 single by Tara McDonaldGive Me MoreSingle by Tara McDonaldReleased2012 (2012)St...

This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Obscenity band – news · newspapers · books · scholar · JSTOR (September 2019) (Learn how and when to r...