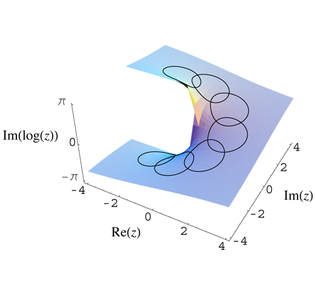
Geometric function theory
|
Read other articles:

Rosy LoversPoster promosi untuk Rosy LoversGenreRomansa Keluarga Komedi DramaDitulis olehKim Sa-kyungSutradaraYoon Jae-moon Jung Ji-inPemeranLee Jang-woo Han SunhwaPenata musikJeon Chang-yeopNegara asalKorea SelatanBahasa asliKoreaJmlh. episode52ProduksiProduser eksekutifKim Dong-gu Byun Jong-eunProduserNoh Do-chul Kwak Ji-hoonLokasi produksiKoreaSinematografiKim Il-manPenyuntingKim Gyu-dongDurasi60 menit Sabtu dan Minggu pukul 20:45 (WSK)Rumah produksiDK E&M Yedang EntertainmentRil...

Fresno California TempleNumber78DedicationApril 9, 2000, by Gordon B. HinckleySite2.34 acres (0.95 ha)Floor area10,700 sq ft (990 m2)Height71 ft (22 m)Official website • News & imagesChurch chronology ←Palmyra New York Temple Fresno California Temple →Medford Oregon Temple Additional informationAnnouncedJanuary 8, 1999, by Gordon B. HinckleyGroundbreakingMarch 20, 1999, by John B. DicksonOpen houseMarch 25 – April 4, 2000Current presidentCliff...

Knut Magne Valle Información personalOtros nombres Phantom FX, MøllarnNacimiento 5 de agosto de 1974 (49 años)Gjerstad, Noruega Nacionalidad NoruegaInformación profesionalOcupación Guitarrista, compositor, productorAños activo 1995 - ActualidadSeudónimo Phantom FX, MøllarnGénero Metal góticoBlack metal sinfónicoMetal industrialMúsica electrónicaInstrumento GuitarraMiembro de Arcturus [editar datos en Wikidata] Knut Magne Valle (nacido el 5 de agosto de 1974 en...

Wappen Deutschlandkarte 48.81083333333311.500833333333389Koordinaten: 48° 49′ N, 11° 30′ O Basisdaten Bundesland: Bayern Regierungsbezirk: Oberbayern Landkreis: Eichstätt Höhe: 389 m ü. NHN Fläche: 55,69 km2 Einwohner: 9875 (31. Dez. 2022)[1] Bevölkerungsdichte: 177 Einwohner je km2 Postleitzahl: 85092 Vorwahl: 08456 Kfz-Kennzeichen: EI Gemeindeschlüssel: 09 1 76 139 LOCODE: DE KHG Marktgliederung: 6 Geme...

Gedsted Parochie van Denemarken Situering Bisdom Bisdom Viborg Gemeente Vesthimmerland Coördinaten 56°41'16,001NB, 9°20'2,000OL Algemeen Inwoners (2004) 1147 Leden Volkskerk (2004) 1081 Overig Kerken Gedsted Kirke Proosdij Vesthimmerlands Provsti Pastoraat Gedsted-Fjelsø Foto's Portaal Denemarken Gedsted is een parochie van de Deense Volkskerk in de Deense gemeente Vesthimmerland. De parochie maakt deel uit van het bisdom Viborg en telt 1081 kerkleden op een bevolking van 114...

Гнат Рошкович Народження 28 вересня 1854(1854-09-28)[1][2][3]Славковце, Михайлівці, Кошицький край, СловаччинаСмерть 29 листопада 1915(1915-11-29) (61 рік) Будапешт, Австро-УгорщинаПоховання КерепешіКраїна Австро-УгорщинаЖанр сакральний живопис, портрет, пейзажНавчання �...

1976 Canadian film by William Fruet This article relies largely or entirely on a single source. Relevant discussion may be found on the talk page. Please help improve this article by introducing citations to additional sources.Find sources: Death Weekend – news · newspapers · books · scholar · JSTOR (May 2018) Death Weekend1977 USA film poster (AIP)Directed byWilliam FruetWritten byWilliam FruetProduced byIvan ReitmanStarringBrenda VaccaroDon StroudChu...

Indian film series Singam Franchiseofficial logoCreated byHariOriginal workSingamOwnerSun Pictures2D EntertainmentStudio GreenFilms and televisionFilm(s)Singam (2010)Singam II (2013)Si3 (2017) Singam (transl. Lion) is an Indian Tamil-language action film series centered around the title character Duraisingam, a tempered honest police officer who aims to eliminate corruption from the society.[1] The film series began in 2010 with the release of Singam, directed by Hari and starri...

Species of plant Piper retrofractum Scientific classification Kingdom: Plantae Clade: Tracheophytes Clade: Angiosperms Clade: Magnoliids Order: Piperales Family: Piperaceae Genus: Piper Species: P. retrofractum Binomial name Piper retrofractumVahl Synonyms Piper officinarum (Miq.) C.DC. Piper retrofractum, the Balinese long pepper or Javanese long pepper, is a flowering vine in the family Piperaceae, cultivated for its fruit, which is usually dried and used as a spice and seasoning. The ...

British fragrance brand Jo Malone LondonTypeSubsidiaryIndustryCosmeticsFoundedLondon, England (1990)FoundersJo Malone CBEProductsFragrance, Bath & BodyParentEstée Lauder Companies (1999–present)WebsiteJo Malone London Jo Malone boutique in Norwich, England Jo Malone Store in Cardiff, Wales Jo Malone London is a British multinational cosmetics company, perfume and scented candle brand,[1] founded by Jo Malone in 1990.[2] It has been owned since 1999 by Estée Lauder.[...

Sekelompok bluefin trevally mendatangi sekelompok anchovies yang membentuk bola impan jika mereka merasa terancam Bola umpan terjadi saat sekawanan ikan kecil membentuk formasi bundar. Tindakan tersebut adalah sebuah tindakan pertahanan terakhir yang dilakukan oleh ikan berkelompok kecil saat mereka terancam oleh para predator. Ikan berkelompok kecil disantap oleh banyak jenis predator, dan untuk alasan itu, mereka disebut ikan umpan atau ikan mangsa. Catatan Referensi Burgess EA (2006) Forag...

Шипіцин Олег Олександровичрос. Олег Александрович Шипицин Народився 17 липня 1974(1974-07-17)Республіка Комі, РРФСР, СРСРПомер 18 березня 2022(2022-03-18) (47 років)Маріуполь, Донецька область, УкраїнаПоховання КосіхаДіяльність військовослужбовецьУчасник Громадянська війна в Таджикис...

ثنائي أكسيد الكبريت ثنائي أكسيد الكبريت ثنائي أكسيد الكبريت الاسم النظامي (IUPAC) ثنائي أكسيد الكبريت أسماء أخرى ثاني أكسيد الكبريت، أكسيد الكبريت الرباعي المعرفات رقم CAS 7446-09-5 بوب كيم 1119 مواصفات الإدخال النصي المبسط للجزيئات O=S=O[1] المعرف الكيميائي الدولي InChI=1S/O2S/c1...

K.P.H. NotoprojoK. P. H. NotoprojoInformasi latar belakangNama lahirWasi JolodoroNama lainKi CokrowasitoK.R.T. WasitodipuroK.R.T. WasitodiningratLahir(1909-03-17)17 Maret 1909 Yogyakarta, Hindia BelandaGenreGamelan, Musik Rakyat, TradisionalPekerjaanMusisi karawitan, Komposer, Dosen, Penata SendratariInstrumenGamelan, Rebab, Saron, Kendhang, BonangTahun aktif1934 - 1992LabelNonesuch Records, CMP RecordsArtis terkaitOrkes Gamelan Pura Paku Alaman, Daya Pradangga, Mardi Wirama Wikiquote memilik...

Chinese Sports Wear Company Anta Sports Products LimitedNative name安踏体育用品有限公司TypePublicTraded asSEHK: 2020Hang Seng IndexISINKYG040111059IndustrySports equipment, textileFounded1991 (1991)FounderDing ShizhongHeadquartersJinjiang, Fujian, ChinaArea servedWorldwideKey peopleDing Shizhong (Chairman)ProductsSneakers, clothing, accessoriesRevenue US$7.7 billion (2021)[1]OwnerAnta International (BVI) (61.84%)Public (38.16%)SubsidiariesAmer SportsWebsiteanta.com...

Esta página cita fontes, mas que não cobrem todo o conteúdo. Ajude a inserir referências. Conteúdo não verificável pode ser removido.—Encontre fontes: ABW • CAPES • Google (N • L • A) (Outubro de 2020) Star Wars: Episódio V - O Império Contra-Ataca[1][2] Star Wars: Episódio V – O Império Contra-AtacaPôster promocional feito por Roger Kastel No Brasil Guerra nas Estrelas: O Império Contra-Ataca[3] Em Portugal Star...

У этого термина существуют и другие значения, см. Нимфа (значения). «Нимфе» SMS[~ 1] Nymphe «Нимфе» в 1899 Служба Германия Класс и тип судна Бронепалубный крейсер типа «Газелле» Изготовитель Germaniawerft, Киль Строительство начато 1898 Спущен на воду 21 ноября 1899 Введён в эксплуатацию...

First airline of the Kingdom of Italy Aero Espresso Italiana flew the Macchi 24bis with 8 passengers in 1927 The Aero Espresso Italiana,[1] called also AEI and Aeroespresso del Levante, was the first airline of the Kingdom of Italy. It was created as a private company for the route Brindisi-Athens-Istanbul; later a second route to Rodhes was added[2] History The airline company, founded in Rome in December 1923, was operating with flights only from 1926. It was based in Rome a...

Eastern Catholic eparchy in Maharashtra, India This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Syro-Malabar Catholic Eparchy of Chanda – news · newspapers · books · scholar · JSTOR (September 2019) (Learn how and when to remove this template message) Eparchy of Chandaचांदा च्या बिशप...

1992 book by Denise Giardina This article may contain an excessive amount of intricate detail that may interest only a particular audience. Please help by spinning off or relocating any relevant information, and removing excessive detail that may be against Wikipedia's inclusion policy. (August 2022) (Learn how and when to remove this template message)This article may be written from a fan's point of view, rather than a neutral point of view. Please clean it up to conform to a higher standard...