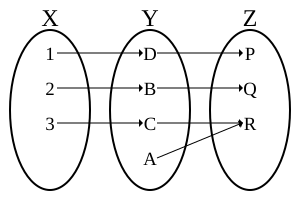
Birebir örten fonksiyon
|
Read other articles:

Type of road sign An example of a British direction sign on the approach to a roundabout. Directional road signs in Luxembourg Road sign on M39 south of Tashkent, showing destinations as far away as Hamburg (Gamburg) and Karachi A direction sign, more fully defined as a direction, position, or indication sign by the Vienna Convention on Road Signs and Signals, is any road sign used primarily to give information about the location of either the driver or possible destinations, and are consider...

Romanian handball club in Bucharest CS Dinamo BucureștiFull nameClubul Sportiv Dinamo BucureștiNickname(s)Câinii Roşii (The Red Dogs)Short nameDinamoFounded1953; 70 years ago (1953)ArenaSala Polivalentă Dinamo (domestic matches) Sala Polivalentă (international matches)Capacity2,538 5,300President Ionuț PopaHead coach Xavier Pascual FuertesLeagueLiga Națională2022–23Liga Națională, 1st of 14 (champions)Club colours Home Away Websi...

MP5 MP5A3 Jenis Pistol mitraliur Negara asal Jerman Sejarah pemakaian Masa penggunaan 1966–sekarang Sejarah produksi Perancang Tilo Möller, Manfred Guhring,Georg Seidl, Helmut Baureuter Tahun 1964 Produsen Heckler & Koch Diproduksi 1966— Spesifikasi Berat 2,54 kg (MP5A2)2,88 kg (MP5A3) Panjang Popor tetap: 680 mm (26.8 in) Popor lipat: 490 mm (masuk) 660 mm (keluar) Panjang laras 225 mm Peluru 9 x 19 mm Luger Mekanisme Semburan Kebelakang, bolt tertutup Rata² tem...

Perang Pembebasan SwediaGustav Vasa memasuki StockholmCarl Larsson, 1908Tanggal1521–23LokasiSkandinaviaHasil Kemenangan SwediaPerubahanwilayah Swedia meraih kemerdekaannya Pembubaran Uni Kalmar Swedia mencabut klaim atas Skania dan BlekingePihak terlibat Swedia Kota Merdeka Lübeck (dari tahun 1522) Uni Kalmar Denmark Norwegia Tokoh dan pemimpin Gustav Vasa Christina Gyllenstierna Anna Eriksdotter Raja Christian II Raja Frederik I ...

Тарасюк Олексій Васильович Старший солдат Загальна інформаціяНародження 12 січня 1990(1990-01-12)КовельСмерть 29 серпня 2014(2014-08-29) (24 роки)НовокатеринівкаВійськова службаПриналежність УкраїнаВид ЗС Збройні силиФормування 51 ОМБрВійни / битви Війна на сході України�...

Town in the state of Florida, United States For other uses, see Hastings (disambiguation). Unincorporated community in Florida, United StatesHastingsUnincorporated communityPotato Growers Association building in Hastings, FloridaLocation in St. Johns County and the state of FloridaCoordinates: 29°43′0″N 81°30′30″W / 29.71667°N 81.50833°W / 29.71667; -81.50833Country United StatesState FloridaCounty St. JohnsSettled1890Incorporated1909Dissolve...

1995 studio album by VangelisVoicesStudio album by VangelisReleasedOctober 24, 1995Recorded1995GenreElectronicaLength54:25LabelEast West (Europe) / Atlantic (USA)ProducerVangelisVangelis chronology Foros Timis Ston Greco(1995) Voices(1995) Oceanic(1996) Professional ratingsReview scoresSourceRatingAllmusic[1] Voices is a studio album by Greek electronic composer Vangelis, released in 1995. Overview Caroline Lavelle sings and plays cello on the song Come To Me, Paul Young sings...

Ocean inlet in Florida, US Location and details of the Ponce de Leon Inlet. Aerial view The Ponce de Leon Inlet is a natural opening in the barrier islands in central Florida that connects the north end of the Mosquito Lagoon and the south end of the Halifax River to the Atlantic Ocean. The inlet originally was named Mosquito Inlet. In 1926 the Florida Legislature changed the name from Mosquito Inlet to Ponce de Leon Inlet. There was precedent for the change. Mosquito County had long before b...

Austrian footballer Sophie Maierhofer Maierhofer in 2015Personal informationDate of birth (1996-08-09) 9 August 1996 (age 27)Place of birth Graz, AustriaHeight 1.70 m (5 ft 7 in)[1]Position(s) Right-backTeam informationCurrent team Sturm GrazNumber 15College careerYears Team Apps (Gls)2016–2019 Kansas Jayhawks 61 (7)Senior career*Years Team Apps (Gls)2015–2016 Werder Bremen 9 (0)2018 UMF Selfoss 5 (1)2020 Aston Villa 1 (0)2020–2021 MSV Duisburg 12 (0)2021– ...

الشرط هو مزاج نحوي، صفة من صفات الكلام الذي يشير إلى موقف المتحدث تجاهه. تُستخدم الأشكال الشرطية من الأفعال عادةً للتعبير عن حالات مختلفة من عدم الواقعية مثل: الرغبة أو العاطفة أو الاحتمال أو الحكم أو الرأي أو الالتزام أو الشك أو الفعل الذي لم يحدث بعد ؛ تختلف المواقف المحد�...

This article is an orphan, as no other articles link to it. Please introduce links to this page from related articles; try the Find link tool for suggestions. (July 2022) Ottoman–Jewish figure Yakup YahyaPersonal detailsBorn1475Lisbon, PortugalDiedOttoman Empire Yakup ben David Tam ibn Yahya (Hebrew: יאקופ יחיא) was born David into a Marrano family in Lisbon, Portugal, in 1475. He later moved to Istanbul to practice his religion (Judaism) more freely, and was regarded as the spirit...

Siegel der Association of American Universities Die Association of American Universities (AAU, deutsch Vereinigung der Amerikanischen Universitäten) ist eine Organisation führender nordamerikanischer Forschungsuniversitäten, die sich für die Förderung der akademischen Forschung und Lehre in Nordamerika einsetzt. Inhaltsverzeichnis 1 Geschichte 2 Mitglieder 2.1 Staatliche Universitäten (38) 2.2 Private Universitäten (31) 2.3 Kanadische Universitäten (2) 2.4 Ehemalige Mitglieder 3 ...

Defunct American robotics and artificial intelligence startup This article is about American robotics and technology company. For the flashcard-based memorization software, see Anki (software). For the Finnish rug manufacturer, see Anki (Finnish company). AnkiTypePrivateIndustryRobotics and artificial intelligenceFounded2010FounderBoris Sofman, Mark Palatucci, and Hanns TappeinerDefunctMay 2019; 4 years ago (2019-05)[1]FateAcquiredHeadquartersSan Francisco, Cali...

2006 American documentary film American ScaryTheatrical release posterDirected byJohn E. HudgensWritten by Sandy Clark John E. Hudgens Produced bySandy ClarkStarring John Zacherle Maila Nurmi Ernie Anderson Distributed byCinema Libre StudioRelease date October 2006 (2006-10) (Hollywood Film Festival) Running time92 minutesCountryUnited StatesLanguageEnglish American Scary is a 2006 American documentary film about the history and legacy of classic television horror hosts, written...

Covert US military operation in VietnamViet Cong soldier with a Type 2 AK-47 rifle. Only one sabotaged cartridge would be placed in a magazine or case of good ammunition Project Eldest Son (also known as “Italian Green” or “Pole Bean”) was a program of covert operations conducted by the United States' Studies and Observation Group (SOG) during the Vietnam War. The project focused on placement of exploding cartridges into supplies used by communist combat forces in southeast Asia. Unit...

For the episode of A Ghost Story for Christmas, see Lost Hearts (film). Short story by M.R. JamesLost HeartsShort story by M.R. JamesGhost Stories of an AntiquaryCountryEnglandLanguageEnglishGenre(s)HorrorPublicationPublication date1895 Sculpture of Mithras sacrificing the bull, of the type described as having been brought from the Levant to Aswarby by Mr. Abney Biblical magician Simon Magus whom Mr. Abney describes as having acquired magical powers through the sacrifice of a boy Hermes T...

Untuk orang lain dengan nama yang sama, lihat John Chiang. Ini adalah nama Tionghoa; marganya adalah Chiang (江). John ChiangBendahara California ke-33PetahanaMulai menjabat 5 Januari 2015GubernurJerry BrownPendahuluBill LockyerKontroler California ke-31Masa jabatan8 Januari 2007 – 5 Januari 2015GubernurArnold SchwarzeneggerJerry BrownPendahuluSteve WestlyPenggantiBetty YeeAnggota Badan Kesetaraan Negara Bagian Californiadari distrik ke-4Masa jabatan3 Januari 1997 – ...

Американский стратегический бомбардировщик B-52 «Stratofortress» (1955). Советский Ту-95 (1956) Американский стратегический бомбардировщик B-1B (1986). Советский Ту-160 (1987). Советский дальний бомбардировщик Ту-22М3. Стратегический бомбардировщик — боевой самолёт, способный нести авиацио�...

1999 greatest hits album by Wildside RecordsBest of WildsideGreatest hits album by Wildside RecordsReleased1999LabelWildside Records Best of Wildside is a 71 minute New Zealand compilation album featuring artists under the record label, Wildside Records, released in 1999.[1][2] It appeared in the New Zealand's top ten compilation albums charts for eight weeks between 29 August and 17 October 1999, peaking at #3.[3][4] Track listing La La Land - Shihad W...

The main rivers of Zimbabwe with their catchment areas This is a list of rivers in Zimbabwe. This list is arranged by drainage basin, with respective tributaries indented under each larger stream's name. Rivers Zambezi River Luenha River Mazowe River (Mazoe River) Ruya River (Luia River) Gairezi River (Cauresi River) Messenguézi River (Umsengedsi River) Mecumbura River (Mkumvura River) Kadzi River Manyame River (Panhame River) (Hunyani River) Angwa River Sanyati River (Umniati River) Munyati...