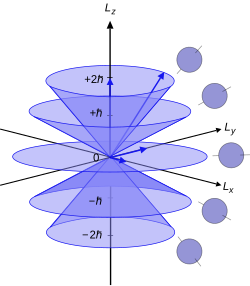
Magnetic quantum number
| |||||||||||||||||||||||||||||||
Read other articles:
Software AdviceTypePublic companyTraded asNYSE: ITFounded2005FounderDon FornesAustin MerrittHeadquartersAustin, TexasUnited StatesKey peopleBlake Clark (General Manager)[1]Websitewww.softwareadvice.com Software Advice is a company that provides advisory services, research, and user reviews on software applications for businesses in over 300 market categories including medical, CRM, HR, construction, business intelligence and marketing automation.[2][3] Co-founded ...

«Aran» redirige aquí. Para la protagonista de Metroid, véase Samus Aran. Islas Aran Oileáin Árann · Aran Islands Costa de Inis Mór, la principal de las islas AranUbicación geográficaMar Océano AtlánticoGolfo bahía de GalwayContinente EuropaCoordenadas 53°07′00″N 9°42′00″O / 53.116666666667, -9.7Ubicación administrativaPaís IrlandaDivisión ConnachtSubdivisión Condado de GalwayDatos geográficosN.º de islas 3Islas Inis Mór Inis Meáin Inis

Місто Браддівіллангл. Braddyville Координати 40°34′43″ пн. ш. 95°01′47″ зх. д. / 40.57861111113877683° пн. ш. 95.02972222224978793° зх. д. / 40.57861111113877683; -95.02972222224978793Координати: 40°34′43″ пн. ш. 95°01′47″ зх. д. / 40.57861111113877683° пн. ш. 95.02972222224978793° зх. д.&...

Rait Ärm Persoonlijke informatie Geboortedatum 13 maart 2000 Geboorteplaats Saku, Estland Sportieve informatie Huidige ploeg Go Sport-Roubaix Lille Métropole Discipline(s) Weg Specialisatie(s) Sprinten Ploegen 20202021-20222023- Tartu 2024-Balticchaincycling.comEquipe continentale Groupama-FDJGo Sport-Roubaix Lille Métropole Portaal Wielersport Rait Ärm (Saku, 13 maart 2000) is een Estisch wielrenner die anno 2020 rijdt voor Go Sport-Roubaix Lille Métropole. Carrière Ärm i...

Overview of archaeological claims of the Book of Mormon This article possibly contains original research. Please improve it by verifying the claims made and adding inline citations. Statements consisting only of original research should be removed. (February 2015) (Learn how and when to remove this template message) Part of a series on theBook of Mormon Origin Cumorah Golden plates Joseph Smith Emma Smith Oliver Cowdery Sidney Rigdon David Whitmer Martin Harris Lucy Harris Book of Mormon witn...

1938 Poirot novel by Agatha Christie Hercule Poirot's Christmas First UK editionAuthorAgatha ChristieCover artistNot knownCountryUnited KingdomLanguageEnglishGenreDetective fictionPublisherCollins Crime ClubPublication date19 December 1938Media typePrint (hardback & paperback)Pages256 first edition, hardbackPreceded byAppointment with Death Followed byMurder is Easy Hercule Poirot's Christmas is a work of detective fiction by British writer Agatha Christie, ...

Horn ParkHorn Park December 2011, looking west, along Gavestone CrescentLocation of Horn Park in the Royal Borough of GreenwichTypePublic parkLocationHorn Park in the Royal Borough of Greenwich, United KingdomCoordinates51°26′35″N 0°01′34″E / 51.443°N 0.026°E / 51.443; 0.026Area16 acres (6.5 ha)Createdc.1940sOwned byRoyal Borough of GreenwichOpen06:00-20:00 or 24 hoursStatusOpen all yearAwardsGreen Flag Award 2012-2021Public transit accessbuses: 2...

هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (أبريل 2018) حصاة برازية حصاة برازية مشاراً إليها بالسهم والتي تسببت بالتهاب في الزائدة الدودية.حصاة برازية مشاراً إليها بالسهم والتي تسببت بالتهاب في الزائدة الدودية. �...

Wakil Perdana MenteriLambang Pemerintah JepangPetahanaLowongsejak 4 Oktober 2021GelarTuan Wakil Perdana MenteriYang MuliaAnggotaKabinetDewan Keamanan NasionalDitunjuk olehPerdana Menteridibuktikan oleh KaisarMasa jabatanTidak ada istilah tetapPejabat perdanaKijuro ShideharaDibentuk3 Mei 1947SuksesiPertamaGaji¥20,916,000 Wakil Perdana Menteri Jepang (副総理code: ja is deprecated , Fuku-sōri) adalah pejabat tertinggi kedua dari cabang eksekutif pemerintah Jepang setelah Perdana Menter...

Chanel 2.55 Chanel 2.55 — легендарна класична сумка, створена будинком моди Chanel. Зміст 1 Історія створення 2 Особливості 3 Різновиди 4 Джерела 5 Посилання Історія створення «Я втомилась носити ридикюли в руках, до того ж я їх постійно гублю», — заявила в 1954 році Коко Шанель. У люто

US indie rock band For other uses, see Mount Joy (disambiguation). Mt. JoyMt. Joy. From left to right: Cooper, Miclau, Byrnes, Quinn, EliopoulosBackground informationOriginPhiladelphia, Pennsylvania, U.S.Genres Alternative rock indie rock folk rock blues rock Americana Years active2016–presentLabels Island R&R[1] Members Matt Quinn Sam Cooper Michael Byrnes Sotiris Eliopoulos Jackie Miclau Websitemtjoyband.com Mt. Joy is an American five-piece indie rock band based in Los Angele...

Diskografi BoAAlbum studio20Album kompilasi6Singel76 Daftar ini berisi diskografi BoA, penyanyi Korea yang berkarier di Korea Selatan, Jepang, dan Amerika Serikat. Kariernya di industri rekaman dimulai pada 25 Agustus 2000 di Korea Selatan. Hingga kini, ia telah merilis 10 album berbahasa Korea, 3 album mini berbahasa Korea, 9 album berbahasa Jepang, 3 album kompilasi di Jepang (satu album berisi lagu berbahasa Korea), dua album remix di Jepang, 28 singel berbahasa Jepang, 5 singel berbahasa ...

Politics of Ukraine Constitution Human rights Presidency President Volodymyr Zelenskyy Office of the President National Security and Defence Council Presidential representatives Presidential symbols Executive Prime Minister Denys Shmyhal Cabinet Shmyhal Government Legislature Verkhovna Rada (parliament) Chairman: Ruslan Stefanchuk Committees People's Deputy of Ukraine Imperative mandate Judiciary Constitutional Court Supreme Court Prosecutor General Local government Local state administration...

Danny Burch England Burch in seinen ersten Jahren in Europa. Personalia Geburtsname Martin Harris Geburtstag 31. Dezember 1981 Geburtsort London, England Karriereinformationen Ringname(n) Danny BurchJoe RiotMartin Stone Körpergröße 183 cm Kampfgewicht 86 kg Angekündigt aus London, England Debüt 2003 Martin Harris (* 31. Dezember 1981 in London, England) ist ein englischer Wrestler. Er ist derzeit Free Agent. Sein bislang größter Erfolg ist der Erhalt der NXT Tag Team Champion...

Border dispute Cambodian–Thai border disputeThe Preah Vihear TempleDate22 June 2008 – 15 December 2011(3 years, 5 months, 3 weeks and 2 days)LocationCambodia–Thailand borderResult Stalemate ICJ decision awards promontory of Preah Vihear to Cambodia[1]Belligerents Cambodia ThailandCommanders and leaders Hun Sen Abhisit VejjajivaCasualties and losses 19 soldiers killed[2]3 civilians killed[3] 16 soldiers killed[4]2 civilians ...

Nigerian charter airline Kabo Air IATA ICAO Callsign N9[1] QNK[1] KABO[1] Founded1980Ceased operations2016HubsMallam Aminu Kano International AirportFleet size1Parent companyKabo Holdings[1]HeadquartersKano, NigeriaKey peopleSaidu Mohammed, Managing director[1]Websitewww.flykabo.com Kabo Air was a Nigerian charter airline headquartered in Kano, Kano State[2] and based at Mallam Aminu Kano International Airport.[3] History A former Kabo A...

Cet article est une ébauche concernant la politique et l’Allemagne. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. TU Berlin Les mouvements sociaux de 1968 en Allemagne et Europe de l'Est surviennent à la suite de manifestations étudiantes contre la guerre du Viêt Nam. À l'est de l'Europe, dans les pays communistes (c'est-à-dire ceux dominés par l'URSS, sans liberté, sans élections, etc. et se fo...

12 Wojskowy Oddział Gospodarczy Odznaka pamiątkowa 12 WOG Historia Państwo Polska Sformowanie 2012 Patron gen. Karol Kniaziewicz Dowódcy Obecny płk Grzegorz Gotowicz Organizacja Dyslokacja Toruń Rodzaj wojsk Logistyka Podległość 1 RBLog. Strona internetowa 12 Wojskowy Oddział Gospodarczy im. gen. dyw. Karola Otto Kniaziewicza (12 WOG) – jednostka logistyczna Sił Zbrojnych Rzeczypospolitej Polskiej. Realizuje zadania zabezpieczenia finansowego i logistycznego jednostek i ins...

Lebanese-American designer and art critic This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article is an orphan, as no other articles link to it. Please introduce links to this page from related articles; try the Find link tool for suggestions. (October 2016) This biography of a living person needs additional citations for verification. Please help by adding reliable sources. Contenti...

See also Culture of South Korea, Korean literature until 1945, and North Korean literature Byeolmadang Library at Starfield COEX Mall in seoul South Korean literature is literature written or produced in South Korea following the division of Korea into North and South in 1945.[1] South Korean literature is primarily written in Korean, though English loanwords are prevalent.[2] Literature by genre Mainstream fiction Part of a series on theCulture of Korea Society History People...