Read other articles:

هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (أكتوبر 2017)  مدينة سمنود هي العاصمة الفرعونية للوجه البحري قديماً وواحدة من أهم المدن التاريخية الفريدة بمحافظة الغربية وإقليم وسط الدلتا.[1] ومع عظم شأنها وتاريخ

Koenraad Goudeseune leest voor uit eigen werk tijdens Poëzie in de Pastorie op 2 juli 2017 Koenraad Jules Cornelius Goudeseune (Ieper, 23 februari 1965 – Gent, 9 december 2020) was een Vlaamse dichter, prozaschrijver, auteur van brieven en recensent. Zijn poëzie wordt gekenmerkt door een mengsel van ironie en sarcasme. In zijn brieven, recensies en verhalen zet Goudeseune zich af tegen de 'ons-kent-onsmentaliteit' in het literaire wereldje. Hij meet zichzelf graag de rol van 'miskende out...

Montañas de Kirthar Ubicación geográficaCoordenadas 27°00′00″N 67°09′20″E / 27, 67.15555556Ubicación administrativaPaís  PakistánDivisión BaluchistánSindhCaracterísticasMáxima cota (2,15 km, 1,42 km, 884 m y 1,34 km)Mapa de localización Montañas de Kirthar Ubicación (Pakistán).[editar datos en Wikidata] Las montañas de Kirthar (en idioma sindhi: کير ٿر جبل) son una cordillera de Pakistán, situada en las provi...

1942 engagement in the American Theatre of World War II Bombardment of Fort StevensPart of the American theater and the Pacific Theater of World War IIAmerican servicemen inspecting a shell crater after the Japanese attack on Fort Stevens.Date21 June 1942LocationFort Stevens, Oregon, Pacific OceanResult Indecisive Japanese retreat successfulBelligerents  United States  JapanCommanders and leaders unknown Akiji TagamiStrength Land:2 artillery pieces1 fortAir:1 aircraft 1 submarineCas...

Dieser Artikel behandelt hauptsächlich den Okkultismus des späten 19. und frühen 20. Jahrhunderts. Zu älteren und jüngeren esoterischen Richtungen, die mitunter auch als Okkultismus oder okkultistisch bezeichnet werden, siehe Esoterik. Okkultismus (von lateinisch occultus ‚verborgen‘, ‚verdeckt‘, ‚geheim‘) ist eine unscharfe Sammelbezeichnung für verschiedenste Phänomenbereiche, Praktiken und weltanschauliche Systeme, wobei okkult etwa gleichbedeutend mit esoterisch, parano...

Pour les articles homonymes, voir Le Prisonnier de Zenda. Le Prisonnier de Zenda Données clés Titre original The Prisoner of Zenda Réalisation John Cromwell Scénario John L. BalderstonWells RootEdward E. Rose Acteurs principaux Ronald ColmanMadeleine CarrollDouglas Fairbanks Jr. Sociétés de production Selznick International Pictures Pays de production États-Unis Genre Aventure Durée 101 minutes Sortie 1937 Pour plus de détails, voir Fiche technique et Distribution Le Prisonnier ...

إليزابيث كاسون معلومات شخصية الميلاد 14 أبريل 1881  تاريخ الوفاة 17 ديسمبر 1954 (73 سنة)   مواطنة ويلز  الحياة العملية المدرسة الأم جامعة برستل  المهنة طبيبة  اللغات الإنجليزية  الجوائز  عضو رتبة الإمبراطورية البريطانية  [لغات أخرى]‏   تعديل مصدري - تعد

Esta página cita fontes, mas que não cobrem todo o conteúdo. Ajude a inserir referências. Conteúdo não verificável pode ser removido.—Encontre fontes: ABW  • CAPES  • Google (N • L • A) (Março de 2019) Primeira Guerra Ítalo-Etíope Data 1895 – 1896 Local Etiópia Desfecho Vitória etíope Tratado de Adis Abeba Beligerantes  Reino da Itália Império Etíope Rússia França Comandantes Humberto I Oreste Ba...

Mathematics developed and used in Ancient Egypt Mathematics in Ancient Egypt redirects here. For the book by Annette Imhausen, see Mathematics in Ancient Egypt: A Contextual History. Ancient Egyptian mathematics is the mathematics that was developed and used in Ancient Egypt c. 3000 to c. 300 BCE, from the Old Kingdom of Egypt until roughly the beginning of Hellenistic Egypt. The ancient Egyptians utilized a numeral system for counting and solving written mathematical problems, often inv...

Cet article est une ébauche concernant l’Alaska. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Pour les articles homonymes, voir Kenai. Kenai Peninsula Borough, Alaska Le glacier de l'ours dans la péninsule de Kenai. Administration Pays États-Unis État Alaska Chef-lieu Soldotna Démographie Population 55 400 hab. (2010) Densité 1,3 hab./km2 Géographie Coordonnées 60° 12′ nor...

Stasiun Mino-Matsuyama美濃松山駅Stasiun Mino-Matsuyama pada September 2007LokasiNanno-cho Matsuyama, Kaizu-shi, Gifu-ken 503-0535JepangKoordinat35°09′38″N 136°38′00″E / 35.1606°N 136.6332°E / 35.1606; 136.6332Koordinat: 35°09′38″N 136°38′00″E / 35.1606°N 136.6332°E / 35.1606; 136.6332Pengelola Yōrō RailwayJalur■ Jalur YōrōLetak dari pangkal11.9 km dari KuwanaJumlah peron2 peron sampingJumlah jalur2Informasi lai...

Stage Door RecordsFounded2007; 16 years ago (2007)FounderTim HuttonDistributor(s)Proper NoteGenreMusical theatre (cast albums)Solo albums by musical theatre performersRestored vintage performancesCountry of originUnited KingdomLocationLondon, United KingdomOfficial websitehttp://www.stagedoorrecords.com/ Stage Door Records is a UK-based label founded in 2007[1] by Tim Hutton that specializes in cast recordings and vocalist albums from stars of stage and screen.[2...

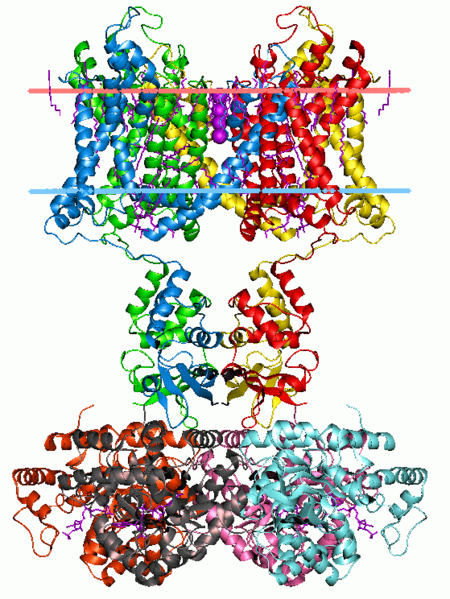
Class of transport proteins Eukaryotic potassium channelPotassium channel, structure in a membrane-like environment. Calculated hydrocarbon boundaries of the lipid bilayer are indicated by red and blue dots.IdentifiersSymbolIon_transPfamPF00520InterProIPR005821SCOP21bl8 / SCOPe / SUPFAMTCDB1.A.1OPM superfamily8OPM protein2a79Membranome217Available protein structures:Pfam  structures / ECOD  PDBRCSB PDB; PDBe; PDBjPDBsumstructure summary Ion channel (bacterial)Potassium channel KcsA....

Japanese baseball player Baseball player Jirō NoguchiPitcher, Outfielder, InfielderBorn: (1920-01-06)January 6, 1920Nagoya, Aichi, JapanDied: May 21, 2007(2007-05-21) (aged 87)Takarazuka, Hyōgo, JapanBatted: RightThrew: RightJBL debut1939, for the Tokyo SenatorsLast NPB appearance1953, for the Hankyu BravesJBL/NPB career pitching statisticsWins-losses237–139Earned run average1.96Strikeouts1,395JBL/NPB career hitting statisticsBatting average.248Home runs9Run batte...

XV Segunda División B de España 1991/92Datos generalesFecha 1 de septiembre de 199128 de junio de 1992PalmarésPrimero G-I. UD SalamancaG-II. UE Sant AndreuG-III. Cartagena FCG-IV. Atlético MarbellaSegundo G-I. CD LugoG-II. ADC ManlleuG-III. Villarreal CFG-IV. CD BadajozDatos estadísticosParticipantes 80 equipos Intercambio de plazas Ascenso(s): CD BadajozCD LugoAtlético MarbellaVillarreal CF Descenso(s): UD AlziraCD BinéfarClub Juventud CambadosFabril DeportivoUD FragaCD FuengirolaCF G...

This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: List of Neon Genesis Evangelion episodes – news · newspapers · books · scholar · JSTOR (June 2014) (Learn how and when to remove this template message) The Platinum Edition box set released in North America by A.D. Vision. Neon Genesis Evangelion is an anime se...

Капская винная змея Научная классификация Домен:ЭукариотыЦарство:ЖивотныеПодцарство:ЭуметазоиБез ранга:Двусторонне-симметричныеБез ранга:ВторичноротыеТип:ХордовыеПодтип:ПозвоночныеИнфратип:ЧелюстноротыеНадкласс:ЧетвероногиеКлада:АмниотыКлада:ЗавропсидыКласс:...

In fisica, il cammino libero medio è la distanza media percorsa da una particella (ad esempio un atomo, una molecola o un fotone) fra due urti successivi[1][2]. Questo parametro è descritto dalla formula generale λ = v m Z {\displaystyle \lambda ={\frac {v_{m}}{Z}}} , dove vm è la velocità media e Z la frequenza di collisione. L'interpretazione rigorosa è pertinenza della meccanica statistica. Spesso il cammino libero medio viene indicato con la lettera greca λ. I...

 シンガポールの政党シンガポール労働者党Workers' Party工人党Parti Pekerja 中国語の名称 新加坡工人党マレー語の名称 Parti Pekerja Singapura (ڤرتي ڤكرجا)タミル語の名称 சிங்கப்பூர் தொழிலாளர் கட்சி議長 シルビア・リム創立 1957年 (1957)本部所在地 216-G Syed Alwi Road #02-03 Singapore 207799青年部 Workers Party Youth Wing党員・党友数 不明政治的思想 社�...

Pour les articles homonymes, voir Bataille de Ramla. Cet article est une ébauche concernant les croisades. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Bataille de Ramla Informations générales Date 27 août 1105 Lieu Ramla Issue Victoire des croisés Belligérants Fatimides Baudouin Ier de Jérusalem Forces en présence Fatimides: 6 300 dont 5 000 fantassins et 1 300 archers turcs Francs: 2&...