進歩党 (日本 1896-1898)
| |||||||||||||||||
Read other articles:

Association of auto racing teams This article is about the team association which existed from 2008 to 2014. For the 1970s and 1980s organisation, see Formula One Constructors' Association. Formula One Teams AssociationThe logo of FOTAAbbreviationFOTAFormation2008Dissolved2014HeadquartersGeneva, SwitzerlandRegion served International Formula One Season summaries 2023 Formula One World Championship 2024 Formula One World Championship Related articles History of Formula One Formula One racing F...

1993 novel by Daniel Blythe The Dimension Riders AuthorDaniel BlytheCover artistJeff CumminsSeriesDoctor Who book:Virgin New AdventuresRelease number20SubjectFeaturing:Seventh DoctorAce, BernicePublisherVirgin BooksPublication dateNovember 1993ISBN0-426-20397-6Preceded byBlood Heat Followed byThe Left-Handed Hummingbird The Dimension Riders is an original novel written by Daniel Blythe and based on the long-running British science fiction television series Doctor Who...

2021 single by Jason Aldean and Carrie UnderwoodIf I Didn't Love YouSingle by Jason Aldean and Carrie Underwoodfrom the album Macon, Georgia ReleasedJuly 23, 2021 (2021-07-23)GenreCountry rock[1]Length3:32Label Broken Bow Macon Capitol Nashville Songwriter(s) Tully Kennedy John Morgan Kurt Allison Bernardis Hughes Producer(s)Michael Knox[2]Jason Aldean singles chronology Blame It On You (2020) If I Didn't Love You (2021) Trouble with a Heartbreak (2022) Carrie U...

Luis Miguel Sánchez CerroPresiden Peru ke-71Masa jabatan27 Agustus 1930 – 1 Maret 1931PendahuluManuel PoncePenggantiRicardo Leoncio ElíasPresiden Republik Peru ke-75Masa jabatan8 Desember 1931 – 30 April 1933PendahuluDavid SamanezPenggantiOscar R. Benavides Informasi pribadiLahir(1889-08-12)12 Agustus 1889Piura, PeruMeninggal30 April 1933(1933-04-30) (umur 43)Lima, PeruKebangsaanPeruPartai politikUnión RevolucionariaSunting kotak info • L • B Luis M...

Dieser Artikel behandelt allgemein Katalysatoren; zur Autoabgaskatalyse siehe Fahrzeugkatalysator. Katalysator (von der Katalyse – griechisch κατάλυσις katálysis, deutsch ‚Auflösung‘ mit lateinischer Endung) bezeichnet in der Chemie einen Stoff, der die Reaktionsgeschwindigkeit durch die Senkung der Aktivierungsenergie einer chemischen Reaktion erhöht, ohne dabei selbst verbraucht zu werden. Er beschleunigt die Hin- und Rückreaktion gleichermaßen und ändert somit ...

Coordenadas: 37° 43' N 12° 29' E Petrosino Comuna Localização PetrosinoLocalização de Petrosino na Itália Coordenadas 37° 43' N 12° 29' E Região Sicília Província Trapani Características geográficas Área total 44 km² População total 7 215 hab. Densidade 164 hab./km² Altitude 16 m Outros dados Comunas limítrofes Marsala, Mazara del Vallo Código ISTAT 081024 Código cadastral M281 Código postal 91020 Prefixo tele...

Міністерство енергетики та вугільної промисловості України (Міненерговугілля України) Загальна інформаціяКраїна УкраїнаДата створення 9 грудня 2010 рокуПопередні відомства Міністерство палива та енергетики України Міністерство вугільної промисловості УкраїниДата ска

Artikel ini tidak memiliki referensi atau sumber tepercaya sehingga isinya tidak bisa dipastikan. Tolong bantu perbaiki artikel ini dengan menambahkan referensi yang layak. Tulisan tanpa sumber dapat dipertanyakan dan dihapus sewaktu-waktu.Cari sumber: BISS – berita · surat kabar · buku · cendekiawan · JSTOR Basic Interoperable Scrambling System (terjemahan: Sistem Pengacakan Interoperabel Dasar), biasanya dikenal sebagai BISS, adalah sistem pengacakan...

You can help expand this article with text translated from the corresponding article in German. (February 2009) Click [show] for important translation instructions. View a machine-translated version of the German article. Machine translation, like DeepL or Google Translate, is a useful starting point for translations, but translators must revise errors as necessary and confirm that the translation is accurate, rather than simply copy-pasting machine-translated text into the English Wikip...

This biography of a living person includes a list of references, related reading, or external links, but its sources remain unclear because it lacks inline citations. Contentious material about living persons that is unsourced or poorly sourced must be removed immediately, especially if potentially libelous or harmful. Please help to improve this article by introducing more precise citations. (May 2023) (Learn how and when to remove this template message) Indian politician M. VincentMember of...

Thin coat designed to resist wind chill and light rain For a lighter non-waterproof zip-up jacket, see Fleece jacket. For other uses, see Windbreaker (disambiguation). This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Windbreaker – news · newspapers · books · scholar · JSTOR (February 2017) (Learn how and when...

1989 film by John Frankenheimer Dead BangTheatrical release posterDirected byJohn FrankenheimerWritten byRobert FosterProduced byStephen J. RothRobert L. RosenStarring Don Johnson Penelope Ann Miller William Forsythe Bob Balaban Tim Reid CinematographyGerry FisherEdited byRobert F. ShugrueMusic byGary ChangProductioncompanyLorimar Film Entertainment[1]Distributed byWarner Bros.[1]Release date March 24, 1989 (1989-03-24) (United States) Running time105 minute...

Singaporean table tennis player Goi Rui XuanNative name魏睿萱Born (2001-02-26) 26 February 2001 (age 22)SingaporeTable tennis career Playing styleRight-handed Shakehand grip Medal record Women's Table Tennis Representing Singapore Asian Championships 2019 Yogyakarta Team 2021 Doha Team Southeast Asian Games 2021 Vietnam Team 2019 Philippines Doubles Goi Rui Xuan (born 26 February 2001) is a Singaporean table tennis player. Her highest career ITTF ranking was 188.[1...

Обкладинка грамоти Нагрудний знак до Грамоти Верховної Ради України Грамота Верховної Ради України є відзнакою Верховної Ради України за вагомий внесок у будь-яку сферу життєдіяльності держави, визначну громадсько-політичну діяльність, заслуги перед Українським наро�...

Singaporean TV series or program NijangalTitle Screen for Season 3நிஜங்கள்GenreFamily DramaCreated byRajarethinam Tamilmaran and Abbas AkbarWritten byJaya RathakrishnanStarringArvind Naidu Soundarajan Bharathi Rani Varman Nishmen NairSharon Sangeetha Bachan Kaur Shyamala Kaushik Nithya Rao Ruban Jitenram Karthikeyan Somasundaram Gayathri Segaran Divya Raveen Harikrishnan PragalathanOpening themeIthu Nijangal by Chandramohan (Season 1)Nijangal Pothumae by Shabir (Season 2)...

Bulgarian Folk Songs, collected by the Miladinov Brothers Dimitar and Konstantin and published by Konstantin in Zagreb at the printing house of A. Jakic, 1861. A letter from Dimitar Miladinov to Victor Grigorovich from February 25th, 1846 about his search for Bulgarian folk songs and artifacts in Macedonia.[1] Letter from Konstantin Miladinov to Georgi Rakovski from 8 January 1861 to explain the use of the term Bulgarian in the title of the collection.[note 1] Announcement of ...

Indian singer Barbie MaanBackground informationBirth nameJasmeet Kaur MaanAlso known asBarbie MaanBornFirozpur, PunjabGenresOccupation(s) Singer Instrument(s)VocalsYears active2018–presentLabelsGold Media RecordsWebsiteBarbie Maan on Facebook, Barbie Maan on InstagramMusical artist Jasmeet Kaur Maan, better known as Barbie Maan, is an Indian singer associated with Punjabi music. Maan is best known for singles Teri Gali written by Guru Randhawa.[1] Music career Born in Firozpur, Punj...

Neem het voorbehoud bij medische informatie in acht.Raadpleeg bij gezondheidsklachten een arts. Syndroom van Korsakov Synoniemen Latijn Korsakovi syndromum[1] Psychosis polyneuritica Nederlands Syndroom van KorsakoffChronisch alcoholdeliriumAlcoholpsychose Coderingen ICD-10 F10 ICD-9 291.1, 294.0 DiseasesDB 14107 eMedicine med/2405 MeSH D020915 Portaal Geneeskunde Het syndroom van Korsakov, ook wel de ziekte van Korsakov genoemd, is een blijvende geheugenstoornis die voor...

Study and creation of maps of imagined places or events A map of the fictional kingdom of Aredia, which is a generic campaign setting used in role-playing games A Visualization of The Cartographic Process A map of the fictional Island of Sodor from The Railway Series by Rev. W. Awdry Fantasy cartography, fictional map-making, or geofiction is a type of map design that visually presents an imaginary world or concept, or represents a real-world geography in a fantastic style.[1] Fantasy...
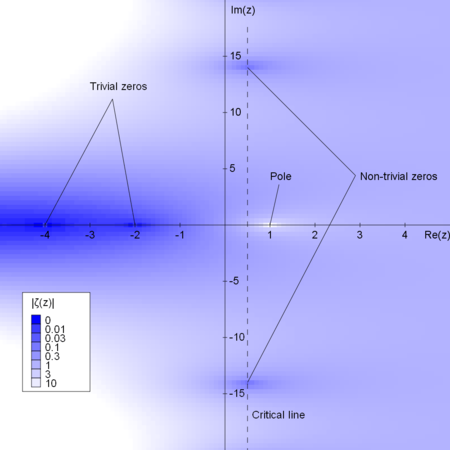
Conjecture on zeros of the zeta function For the musical term, see Riemannian theory. This plot of Riemann's zeta (ζ) function (here with argument z) shows trivial zeros where ζ(z) = 0, a pole where ζ(z) = ∞ {\displaystyle \infty } , the critical line of nontrivial zeros with Re(z) = 1/2 and slopes of absolute values. The real part (red) and imaginary part (blue) of the Riemann zeta function ζ(s) along the critical line in the complex plane with real part Re(s) = 1/2. The first non...
