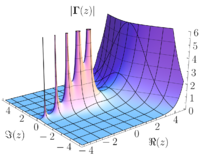
Picard theorem
|
Read other articles:

Изображение было скопировано с wikipedia:en. Оригинальное описание содержало: Зміст 1 Summary 2 Licensing 3 Fair use in You Wanted the Best, You Got the Best!! 4 Source Summary Cover for You Wanted the Best, You Got The Best by KISS. Licensing Це зображення є обкладинкою музичного альбому або синглу. Найімовірніше, авторськими правами на обкладин

هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (أبريل 2019) رون باريت معلومات شخصية الميلاد 25 يوليو 1937 (86 سنة) مواطنة الولايات المتحدة الحياة العملية المدرسة الأم ثانوية الفنون والتصميم [لغات أخرى] ا

2016年夏季奥林匹克运动会苏里南代表團苏里南国旗IOC編碼SURNOC苏里南奥林匹克委员会網站surolympic.org(英文)2016年夏季奥林匹克运动会(里約熱內盧)2016年8月5日至8月21日運動員6參賽項目4个大项旗手开幕式:苏林·奥普提(羽毛球)[1]闭幕式:里约奥组委志愿者[2]历届奥林匹克运动会参赛记录(总结)夏季奥林匹克运动会19601964196819721976198019841988199219962000200420082...

A sinopse deste artigo pode ser extensa demais ou muito detalhada. Por favor ajude a melhorá-la removendo detalhes desnecessários e deixando-a mais concisa. (Junho de 2022) Sucker Punch Sucker Punch - Mundo Surreal (PRT/BRA) Sucker Punch - Mundo SurrealPôster promocional Estados Unidos Canadá2011 • cor • 110 min Gênero açãodramafantasiasteampunk Direção Zack Snyder Produção Deborah SnyderZack Snyder Roteiro Zack SnyderSteve Shibuya História Za...

المجلس التأسيسي الكويتي القيادة رئيس المجلس التأسيسي عبداللطيف محمد الغانممنذ 20 يناير 1962 نائب رئيس المجلس التأسيسي الدكتور أحمد محمد الخطيبمنذ 20 يناير 1962 الأعضاء 50 عضو منتخب من الشعب الكويتيرئيس مجلس الوزراء والوزراء بحكم مناصبهم نظام انتخابي 10 دوائر أنتخابية تنتخب كل دا

Instituciones políticas de la Unión EuropeaSede del Parlamento Europeo en Estrasburgo.Interior del Edificio Europa (Bruselas), sede principal del Consejo Europeo, del Consejo de la Unión Europea y de otros órganos de alto nivel de la UE.[1]Sede de la Comisión Europea. El marco institucional[2] de la Unión Europea (UE) se compone de las siete altas instituciones a las que los Estados miembros atribuyen competencias de gobierno para el ejercicio comunitario de parte de sus p...

هذه المقالة تحتاج للمزيد من الوصلات للمقالات الأخرى للمساعدة في ترابط مقالات الموسوعة. فضلًا ساعد في تحسين هذه المقالة بإضافة وصلات إلى المقالات المتعلقة بها الموجودة في النص الحالي. (سبتمبر 2019) وكالة الأنباء العمانية تفاصيل الوكالة الحكومية البلد سلطنة عمان تأسست 1986&#...

Love is MoveSingel oleh Secretdari album Moving in SecretDirilis18 Oktober 2011 (2011-10-18)FormatCD single, digital downloadDirekam2011GenrePop, K-popDurasi3:20LabelTS EntertainmentPenciptaKang Jiwon, Kim KibumProduserKang Jiwon, Kim Kibum Love is Move (Korean: 사랑은 MOVE, bergaya sebagai Love is MOVE) adalah lagu oleh girlband Korea Selatan, Secret. Singel ini dirilis sebagai singel pemimpin untuk album studio pertama mereka, Moving in Secret. Lagu ini ditulis oleh Kang Jiwon dan K...

Joshua Michael Stern Información personalNacimiento 12 de enero de 1961 (62 años)Nacionalidad EstadounidenseInformación profesionalOcupación Director de cine, guionistaAños activo 1996–presenteObras notables Neverwas, Swing Vote, Jobs[editar datos en Wikidata] Joshua Michael Stern es un director de cine y guionista estadounidense. Ha dirigido tres películas: Neverwas (2005), Swing Vote (2008) y Jobs, basada en la vida de Steve Jobs, fundador de Apple Computer.[1] Film...

اقتصاد بورتوريكوعامالدولة الولايات المتحدة عملة دولار أمريكي الإحصائياتنمو الناتج الإجمالي -1.8 نسبة مئوية[1](2016) نصيب الفرد من الناتج الإجمالي 30518 دولار أمريكي[2](2016) التضخم الاقتصادي (CPI) -0.2 نسبة مئوية[3](2016) تعديل - تعديل مصدري - تعديل ويكي بيانات سان خوان صنف البن...

BrañoseraMunisipalitas LambangNegara SpanyolKomunitas otonom Castile and LeónProvinsiPalenciaMunisipalitasBrañoseraLuas • Total61,97 km2 (23,93 sq mi)Ketinggian1.220 m (4,000 ft)Zona waktuUTC+1 (CET) • Musim panas (DST)UTC+2 (CEST)Situs webSitus web resmi Brañosera adalah sebuah munisipalitas yang terletak di provinsi Palencia, Castilla y León, Spanyol. Menurut sensus 2004,[1] munisipalitas ini berpenduduk sebesar 284 jiw...

Acer Aspire 8920. Acer Aspire adalah sebuah seri komputer oleh Acer yang ditujukan untuk pengguna rumahan atau keperluan bisnis kecil. Seri Aspire terdiri dari komputer desktop dan komputer jinjing (bahasa Inggris: laptop). Model Acer Aspire Aspire 1570 Aspire 1640/1642NWLMi Aspire 2930 Aspire 3023 WLMi Aspire 3410 Aspire 3620 Aspire 3610 Aspire 3680 Aspire 3690 Aspire 3810 T Aspire 3935 Aspire 4315 Aspire 4520 Aspire 4530 Aspire 4540 Aspire 4710 Aspire 4715 Z Aspire 4720 Z Aspire 4730 Z ...

AquitoNama lahirLourenço CabralLahir Timor PortugisPengabdian Timor LesteDinas/cabang Falintil Pasukan Pertahanan Timor LestePangkat Letnan FDTLPerang/pertempuranPendudukan Indonesia di Timor Timur Letnan FDTL Aquito adalah tokoh militer Timor Leste. Dia pernah menjabat sebagai Komandan Peleton Falintil.[1] Kepangkatan Lambang Pangkat Tanggal Letnan FDTL 2 Februari 2022 — sekarang Referensi ^ Jornal da República: Edisi 20 Desember 2006 (PDF; 403 kB), diakses pada 23 Maret 2018. (d...

Абрахам Хондіус. «Авантюрна подорож в Арктику», 1677. Музей Фіцвільяма Золота доба голландського живопису — найвидатніша доба в живопису в Нідерландах, що приблизно припадає на усе 17 століття. Зміст 1 Історичні умови 2 Утрехтські караваджисти 3 Голландці і колоніалізм 4 �...

2022 single by SiMThe RumblingSingle by SiMfrom the EP Beware LanguageEnglishReleasedFebruary 7, 2022 (2022-02-07)Recorded2021Genre Heavy metal[1] metalcore[2] symphonic metal Length3:40LabelPony CanyonComposer(s)SiMLyricist(s)MAHSiM singles chronology Captain Hook (2020) The Rumbling (2022) Under the Tree (2023) Audio samplefilehelpMusic videoThe Rumbling on YouTubeAlternate coverTV size version cover The Rumbling is a single recorded by Japanese alternative me...

Dominican Republic basketball player (born 1981) In this Spanish name, the first or paternal surname is Garcia and the second or maternal family name is Gutiérrez. Francisco GarcíaGarcía with the Dominican national team in 2011Personal informationBornDecember 31, 1981[a]San Francisco de Macoris, Dominican RepublicNationalityDominicanListed height6 ft 7 in (2.01 m)Listed weight195 lb (88 kg)Career informationHigh schoolThe Winchendon School(Winchendo...

Альтеро Маттеоліітал. Altero Matteoli Ім'я при народженні італ. Altero MatteoliНародився 8 вересня 1940(1940-09-08) (83 роки)Чечина, ТосканаПомер 18 грудня 2017(2017-12-18)[1] (77 років)Капальбіо, Провінція Гроссето, Тоскана, Італія·дорожньо-транспортна пригодаКраїна Італія Королівство ІталіяД...

Localização dos arquipélagos que constituem Kiribati no Oceano Pacífico Esta é a lista das ilhas de Kiribati. A República de Kiribati consta de 32 atóis e uma ilha de coral. Estas ilhas estão dispersas ao longo dos três grupos de ilhas que formam Kiribati: Ilhas Gilbert Ilhas Fénix Ilhas da Linha (ou Espórades Equatoriais) Todas as Ilhas Fénix, menos a ilha Canton que tem uma baixa densidade populacional, estão desabitadas. As restantes Ilhas Fénix são uma zona marinha protegid...

1956 film by Walter Lang This article is about the 1956 film. For other uses, see The King and I (disambiguation). The King and ITheatrical release poster by Tom ChantrellDirected byWalter LangScreenplay byErnest LehmanBased on The King and I1951 musicalby Richard Rodgers and Oscar Hammerstein II Anna and the King of Siam1944 novelby Margaret Landon Produced byCharles BrackettStarring Deborah Kerr Yul Brynner Rita Moreno Martin Benson Rex Thompson CinematographyLeon ShamroyEdited byRobert Sim...

Для улучшения этой статьи желательно: Проставить сноски, внести более точные указания на источники.После исправления проблемы исключите её из списка. Удалите шаблон, если устранены все недостатки. Нью-джек-свинг Направление Современный ритм-н-блюз Истоки Соул, Фанк, Хип-...