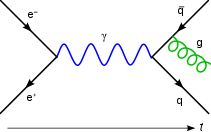
Ghost (physics)
|
Read other articles:

Cet article est une √©bauche concernant un a√©ronef et l‚ÄôURSS. Vous pouvez partager vos connaissances en l‚Äôam√©liorant (comment ?) selon les recommandations des projets correspondants. Tupolev ANT-20 ¬ę Maxime Gorki ¬Ľ Constructeur Tupolev R√īle Avion de propagande/transport Premier vol 19 mai 1934[1] Mise en service 1934 Date de retrait 14 d√©cembre 1942 Nombre construits 2 √Čquipage 11 Motorisation Moteur Mikouline AM-34 Nombre 8 Type 12 cylindres en V Puissance unitaire ...

–ě–Ņ–ł—Ā —Ą–į–Ļ–Ľ—É –ě–Ņ–ł—Ā –ě–Ī–ļ–Ľ–į–ī–ł–Ĺ–ļ–į —Ā–ł–Ĺ–≥–Ľ—É Tha 'Mai Allios –Ē–∂–Ķ—Ä–Ķ–Ľ–ĺ http://en.wikipedia.org/wiki/File:Paparizou-Tha_Mai_Allios.JPG –ß–į—Ā —Ā—ā–≤–ĺ—Ä–Ķ–Ĺ–Ĺ—Ź 2009 –ź–≤—ā–ĺ—Ä –∑–ĺ–Ī—Ä–į–∂–Ķ–Ĺ–Ĺ—Ź Sony Music Greece/RCA –õ—Ė—Ü–Ķ–Ĺ–∑—Ė—Ź –ī–ł–≤. –Ĺ–ł–∂—á–Ķ –ě–Ī“Ď—Ä—É–Ĺ—ā—É–≤–į–Ĺ–Ĺ—Ź –ī–ĺ–Ī—Ä–ĺ–Ņ–ĺ—Ä—Ź–ī–Ĺ–ĺ–≥–ĺ –≤–ł–ļ–ĺ—Ä–ł—Ā—ā–į–Ĺ–Ĺ—Ź –Ĺ–Ķ –≤–ļ–į–∑–į–Ĺ–ĺ –Ĺ–į–∑–≤—É —Ā—ā–į—ā—ā—Ė [?] –ě–Ņ–ł—Ā –ě–Ī–ļ–Ľ–į–ī–ł–Ĺ–ļ–į —Ā–ł–Ĺ–≥–Ľ—É Tha 'Mai Allios –Ē–∂–Ķ—Ä–Ķ–Ľ–ĺ http://...

For other uses, see Islam and slavery (disambiguation). Harem Scene with Mothers and Daughters in Varying Costumes from Qajar Iran, late 19th or early 20th century[1] The Aurut Bazaar, or Slave Market, c. 1836 Concubinage in the Muslim world was the practice of Muslim men entering into intimate relationships without marriage,[2] with enslaved women,[3] though in rare, exceptional cases, sometimes with free women.[4][5][6] If the ...

–í—Ā–Ķ—Ā–≤—Ė—ā–Ĺ—Ė–Ļ –ļ—É—Ä—É–Ľ—ā–į–Ļ (–ļ–ĺ–Ĺ–≥—Ä–Ķ—Ā) –Ī–į—ą–ļ–ł—Ä—Ė–≤ (–Ī–į—ą–ļ. –Ď”©—ā”ô –ī–ĺ–Ĺ—ä—Ź –Ī–į—ą“°–ĺ—Ä—ā—ā–į—Ä—č “°–ĺ—Ä–ĺ–Ľ—ā–į–Ļ—č (–ļ–ĺ–Ĺ–≥—Ä–Ķ—Ā—č)) ‚ÄĒ –ľ—Ė–∂–Ĺ–į—Ä–ĺ–ī–Ĺ–ł–Ļ —Ā–ĺ—é–∑ –≥—Ä–ĺ–ľ–į–ī—Ā—Ć–ļ–ł—Ö –ĺ—Ä–≥–į–Ĺ—Ė–∑–į—Ü—Ė–Ļ, –Ņ–ĺ–ļ–Ľ–ł–ļ–į–Ĺ–ł–Ļ –≤–ł—Ä—Ė—ą—É–≤–į—ā–ł –∑–į–≤–ī–į–Ĺ–Ĺ—Ź –ĺ–Ī'—Ē–ī–Ĺ–į–Ĺ–Ĺ—Ź, –Ķ—ā–Ĺ–ĺ–ļ—É–Ľ—Ć—ā—É—Ä–Ĺ–ĺ–≥–ĺ —Ä–ĺ–∑–≤–ł—ā–ļ—É —ā–į –ĺ–Ĺ–ĺ–≤–Ľ–Ķ–Ĺ–Ĺ—Ź –Ī–į—ą–ļ–ł—Ä—Ė–≤. –®—ā–į–Ī-–ļ–≤–į—Ä—ā–ł—Ä–į —Ä–ĺ–∑—ā–į—ą–ĺ–≤–į–Ĺ–į –≤ –Ď–į—ą–ļ–ĺ—Ä—ā–ĺ—Ā—ā–į–Ĺ

śŹźÁ§ļÔľöś≠§śĚ°ÁõģÁöĄšłĽťĘėšłćśėĮ1948ŚĻīšł≠ŤŹĮśįĎŚúčÁęčś≥ēŚßĒŚď°ťĀłŤąČ„Äā ŚúčśįĎŚ§ßśúÉÁ¨¨šłÄŚĪÜŚúčśįĎŚ§ßśúÉšĽ£Ť°®ťĀłŤąČ ← 1918 1947ŚĻī11śúą21śó•Ôľć1947ŚĻī11śúą23śó• 1969 → Ś§öśēłťĽ® ŚįĎśēłťĽ® Á¨¨šłČŚ§ßťĽ® ťĘÜŤĘĖ ŤĒ£šł≠ś≠£ śõĺÁź¶ ŚĺźŚāÖťúĖ śĒŅŚÖö ŚúčśįĎťĽ® ťĚíŚĻīťĽ® śįĎÁ§ĺťĽ® šĽ£Ť°®ťÄČŚĆļ śĶôśĪüŚ•ČŚĆĖ ŚõõŚ∑ĚťöÜśėĆ ŚĽ£śĚĪŚíĆŚĻ≥ ŤĶĘŚĺóŚł≠ś¨° 2901 76 68 ŚúčśįĎŚ§ßśúÉÁ¨¨šłÄŚĪÜŚúčśįĎŚ§ßśúÉšĽ£Ť°®ťĀłŤąČÁāļšł≠ŤŹĮśįĎŚúčÁöĄŚõĹšľöŤģģŚĎėťĀłŤąČԾƌ֮Śú茟ĄŚúįŚąÜ

Vivi Kreutzberger Vivi Kreutzberger en 2018Informaci√≥n personalNombre de nacimiento Vivian Nina Kreutzberger MuchnickNacimiento 28 de agosto de 1965 (58 a√Īos)Santiago, ChileNacionalidad ChilenaFamiliaPadres Teresa Muchnick Rosenblum y Mario Kreutzberger BlumenfeldC√≥nyuge Robert Wilkins (2003-actualidad)Hijos Ilan, Amir, Nicole, Yael y SharonEducaci√≥nEducada en Instituto Hebreo Informaci√≥n profesionalOcupaci√≥n Presentadora de televisi√≥nCanal Canal 13 (1999-2009)Mega (2010-2012)TV+ ...

Pour les articles homonymes, voir IRSN. Institut de radioprotection et de s√Ľret√© nucl√©aireLogo de l'Institut de radioprotection et de s√Ľret√© nucl√©aire.HistoireFondation 2001CadreType Agence fran√ßaise de s√©curit√© sanitaireForme juridique √Čtablissement public national √† caract√®re industriel ou commercial dot√© d'un comptable publicDomaine d'activit√© Radioprotection, S√Ľret√© nucl√©aireSi√®ge 31 avenue de la Division-Leclerc, Fontenay-aux-RosesPays FranceOrganisationEffectif 1...

Scottish Canals(Scottish Gaelic: Canàlan na h-Alba)Scottish Canals logoPredecessorBritish WaterwaysFormation2 July 2012 (2012-07-02)Typeexecutive non-departmental public body of the Scottish GovernmentHeadquartersGlasgow, Scotland, UKRegion served ScotlandChairman of BoardMaureen Campbell[1]Chief ExecutiveCatherine Topley[1]Budget £19.2m[2]Staff 284[2]Websitewww.scottishcanals.co.ukFormerly calledBritish Waterways (Scotland) Scottish Canals (Sc...

Transit of Venus beralih ke halaman ini. Untuk kegunaan lain, lihat Transit of Venus (disambiguasi). 171 ångströms (17.1 nm)Spektrum tampak kontinu.Foto ultraviolet dan spektrum kontinu pada transit Venus 2012, diambil dari Solar Dynamics Observatory milik NASA. Transit Venus melintasi Matahari terjadi ketika planet Venus melintas di antara Matahari dan Bumi (atau planet lain), membuatnya terlihat di atas keping surya. Dalam sebuah transit, Venus dapat terlihat dari Bumi sebagai titik hitam...

German fairy tale The Water of LifeArthur Rackham, 1916Folk taleNameThe Water of LifeAarne‚ÄďThompson groupingATU 551CountryGermanyPublished inGrimms' Fairy TalesRelated The Brown Bear of the Green Glen The King of England and his Three Sons The Water of Life (German: Das Wasser des Lebens) is a German fairy tale collected by the Brothers Grimm, tale number 97.[1] It is Aarne-Thompson type 551.[2] John Francis Campbell noted it as a parallel of the Scottish fairy tale, The Bro...

Midpeninsula Regional Open Space District This article includes a list of references, related reading, or external links, but its sources remain unclear because it lacks inline citations. Please help to improve this article by introducing more precise citations. (January 2023) (Learn how and when to remove this template message) One of the many trails at Los Trancos Openspace Preserve Los Trancos Open Space Preserve is a 274-acre (1.11 km2) open space preserve, located in San Mateo and S...

Japanese anime television series Rampo Kitan: Game of LaplaceKey visual for the anime featuring the main protagonists, Akechi (top left), Kobayashi (right), and Hashiba (bottom left).šĻĪś≠©Ś•áŤ≠ö Game of LaplaceGenreMystery,[1] psychological[2]Created byEdogawa Ranpo Anime television seriesDirected bySeiji KishiWritten byMakoto UezuMusic byMasaru YokoyamaStudioLercheLicensed byAUS: Madman EntertainmentNA: FunimationOriginal networkFuji TV (Noitamina)En...

American politician John JanasMember of the Massachusetts House of Representatives for the 15th Middlesex districtIn office1963‚Äď1969Preceded byCornelius DesmondMayor of Lowell, MassachusettsIn office1954‚Äď1955Preceded byHenry BeaudrySucceeded bySamuel S. Pollard Personal detailsBornSeptember 4, 1910Lowell, MassachusettsDiedDecember 5, 1969 (aged 59)Lowell, MassachusettsPolitical partyRepublicanAlma materLowell Technological InstituteOccupationReal estate and insurance broker[1] Joh...

Belimbing sayur Klasifikasi ilmiah Kerajaan: Plantae Divisi: Magnoliophyta Kelas: Magnoliopsida Ordo: Oxalidales Famili: Oxalidaceae Genus: Averrhoa Spesies: Averrhoa bilimbi. L Buah Belimbing Wuluh Belimbing sayur (dikenal pula dengan nama belimbing wuluh, belimbing buluh, belimbing botol, belimbing besi, atau belimbing asam) (Averrhoa Bilimbi Linn) merupakan sejenis pohon kecil yang diperkirakan berasal dari Kepulauan Maluku, dan dikembangbiakkan serta tumbuh bebas di Indonesia, Filipi...

Cet article est une √©bauche concernant un √©conomiste et un sociologue allemand. Vous pouvez partager vos connaissances en l‚Äôam√©liorant (comment ?) selon les recommandations des projets correspondants. Pour les articles homonymes, voir Oppenheimer. Franz OppenheimerFranz Oppenheimer sur un timbre de la Deutsche Bundespost.BiographieNaissance 30 mars 1864BerlinD√©c√®s 30 septembre 1943 (√† 79 ans)Los Angeles (√Čtats-Unis)S√©pulture S√ľdfriedhof (d)Nationalit√© allemandeFormation ...

Áü≥Ś∑ĚÁúĆŚįŹśĚ匳āŚúėŚćĀťÉéŤäłŤ°ďŚäጆī„ĀÜ„āČ„āČ Ś§ĖŤ¶≥ śÉÖŚ†ĪťÄöÁßį ŚúėŚćĀťÉéŤäłŤ°ďŚäጆī„ĀÜ„āČ„āČ[1][2][3]ś≠£ŚľŹŚźćÁßį Áü≥Ś∑ĚÁúĆŚįŹśĚ匳āŚúėŚćĀťÉéŤäłŤ°ďŚäጆī„ĀÜ„āČ„āČ[4][5][6][7]śóߌźćÁßį Áü≥Ś∑ĚÁúĆ„Āď„Āĺ„Ā§ŤäłŤ°ďŚäጆī„ĀÜ„āČ„āČ[1][5][6][8]ťĖ蝧® 2004ŚĻī3śúą14śó•ťĖ蝧®ŚÖ¨śľĒ śĚĺÁęĻŚ§ßś≠ĆŤąěšľé[9]ŚģĘŚł≠śēį 851Śł≠ÔľąŚ§ß„Éõ„Éľ„ÉęÔľČ250Śł≠ÔľąŚįŹ„Éõ„Éľ„ÉęԾȌĽ∂ŚļäťĚĘÁ©ć 7791,35m²Ť®≠Śāô „Éõ„Éľ„ÉęÔ...

Amsal 12Kitab Amsal lengkap pada Kodeks Leningrad, dibuat tahun 1008.KitabKitab AmsalKategoriKetuvimBagian Alkitab KristenPerjanjian LamaUrutan dalamKitab Kristen20← pasal 11 pasal 13 → Amsal 12 (disingkat Ams 12) adalah bagian dari Kitab Amsal dalam Alkitab Ibrani dan Perjanjian Lama di Alkitab Kristen.[1][2] Teks Naskah sumber utama: Masoretik, Septuaginta dan Naskah Laut Mati. Pasal ini terdiri dari 28 ayat. Berisi amsal-amsal raja Salomo bin Daud.[3] St...

Radio station in Calgary CKRY-FMCalgary, AlbertaBroadcast areaCalgary Metropolitan RegionFrequency105.1 MHz (FM) (HD Radio)BrandingCountry 105ProgrammingFormatCountryHD2: CHQRAffiliationsPremiere NetworksOwnershipOwnerCorus Entertainment(Corus Radio Company)Sister stationsCHQR, CFGQ-FM, CICT-DT, CISA-DTHistoryFirst air dateJuly 9, 1982Call sign meaningC(K)algaRY (broadcast area) orC(K)ountRY (format)Technical informationClassCERP100,000 wattsHAAT298.5 meters (979 ft)Transmitter coordinat...

1937 single by The Raymond Scott QuintettePowerhouseBrunswick 78 rpm issue of Raymond Scott's PowerhouseSingle by The Raymond Scott QuintetteB-sideThe Toy TrumpetReleased1937RecordedFebruary 20, 1937Genre Novelty Length2:56LabelMaster RecordsBrunswickColumbiaSongwriter(s)Raymond Scott Powerhouse (1937) is an instrumental musical composition by Raymond Scott, perhaps best known today as the assembly line music in animated cartoons released by Warner Bros. History In scripted comments read on t...

It has been suggested that this article be merged into Siberia. (Discuss) Proposed since November 2023. See also: Siberia and European Russia Subregion of the Asian continent North AsiaArea13,100,000 km2 (5,100,000 sq mi)Population37 million (2021 census)Population density2.6 per km2 (7.4 per mi2)GDP (nominal)$480 billion (2021)[1]GDP per capita$13,000 (2021)Ethnic groupsMajority SlavicMinority Tungusic, Mongolic and Turkic peoplesReligionsMajority Orthodox ChristianDem...
![{\displaystyle S=\int d^{4}x\left[aX^{2}-bX\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/a5bb1a2b5155bf9fd4f4d21caf598f6aec317a88)

