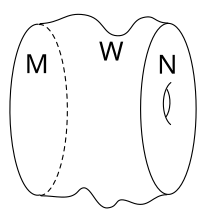
Differential topology
|
Read other articles:

Species of stick insect Common walkingstick Scientific classification Domain: Eukaryota Kingdom: Animalia Phylum: Arthropoda Class: Insecta Order: Phasmatodea Family: Diapheromeridae Genus: Diapheromera Species: D. femorata Binomial name Diapheromera femorata(Say, 1824) Diapheromera femorata The common walkingstick or northern walkingstick (Diapheromera femorata) is a species of phasmid or stick insect found across North America. The average length of this species is 75mm (3 in) for...

Para otros usos de este término, véase Roosevelt.Parque Nacional de los Derechos de los Niños y Niñas - Franklin Delano Roosevelt Monumento Histórico Nacional SituaciónPaís UruguayUbicación Avenida de las AméricasDepartamento CanelonesCiudad Ciudad de la CostaEcorregión San José de CarrascoCoordenadas 34°51′29″S 56°01′18″O / -34.85816667, -56.02171389Datos generalesAdministración Gobierno de CanelonesGrado de protección Monumento Histórico Nac...

كبير المحققين Inquisitor Generalis كبير المحققين البلد إسبانيا الإمبراطورية الإسبانية عن المنصب المعين ألملك تأسيس المنصب 1483 آخر حامل للمنصب خيرونيمو كاستيون إي سالاس تعديل مصدري - تعديل كبير المحققين أو المحقق الأكبر (باللاتينية: Inquisitor Generalis) هو كبير موظفي ديوان التحقيق (محا

Artikel ini perlu dikembangkan agar dapat memenuhi kriteria sebagai entri Wikipedia.Bantulah untuk mengembangkan artikel ini. Jika tidak dikembangkan, artikel ini akan dihapus. Artikel ini tidak memiliki referensi atau sumber tepercaya sehingga isinya tidak bisa dipastikan. Tolong bantu perbaiki artikel ini dengan menambahkan referensi yang layak. Tulisan tanpa sumber dapat dipertanyakan dan dihapus sewaktu-waktu.Cari sumber: Pulau Saseel – berita · surat kabar · ...

Volleyball Championship 2021 Boys' U19 World Championshipوالیبال قهرمانی زیر ۱۹ سال پسران جهانتهران ۲۰۲۱Tournament detailsHost nation IranCityTehranDates24 August – 2 SeptemberTeams20 (from 5 confederations)Venue(s)2 (in 1 host city)Champions Poland (2nd title)Runners-up BulgariaThird place IranFourth place RussiaTournament awardsMVP Tytus NowikBest Setter Stoil PalevBest OH Aleksandar Nikolov Kamil SzymenderaBest MB...

For other uses, see Heart Like a Wheel (disambiguation). 1974 studio album by Linda RonstadtHeart Like a WheelStudio album by Linda RonstadtReleasedNovember 19, 1974 (1974-11-19)RecordedJune–September 1974StudioThe Sound Factory and Clover Recorders, Los Angeles, Track Recorders, Maryland, Record Plant and The Hit Factory, New York City, Trident Studios and AIR Studios, London[1]Genre Country rock pop rock[2] soft rock[2] Length31:40LabelCapito...

María Pilar Fernández Otero Información personalNacimiento 1937 Bandeira, PontevedraNacionalidad EspañolaEducaciónEducada en Universidad de Santiago de Compostela Información profesionalCargos ocupados Catedrático de universidad (desde 1970) Empleador Universidad de NavarraUniversidad de BarcelonaUniversidad de Santiago de Compostela Miembro de Real Academia Nacional de Farmacia [editar datos en Wikidata] María Pilar Fernández Otero, (Bandeira, Pontevedra, 1937), es ca...

Daftar ini belum tentu lengkap. Anda dapat membantu Wikipedia dengan mengembangkannya. Peta Jaringan Nasional Jaringan Nasional (atau Jaringan Truk Nasional ) adalah jaringan jalan raya negara bagian dan antarnegara bagian yang disetujui untuk pengemudi truk komersial di Amerika Serikat . Undang -Undang Bantuan Transportasi Permukaan tahun 1982 mengizinkan pembentukan jaringan jalan raya nasional yang ditujukan untuk digunakan oleh truk- truk besar . Di jalan raya ini, batas lebar dan panjang...

Lenguas saharianasDistribución geográfica Sahara, África centralPaíses Chad Níger Nigeria Sudán CamerúnFiliación genética Nilo-sahariano SaharianoSubdivisiones Sahariano orientalSahariano occidental [editar datos en Wikidata] Las lenguas saharianas constituyen una pequeña familia de lenguas habladas a lo largo del Sáhara oriental, extendiéndose del noroeste de Darfur al sur de Libia, además de por el norte y centro de Chad, el e...

هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (يناير 2022) إيفيكا كالينيتش معلومات شخصية الميلاد 26 مارس 1956 (67 سنة) سبليت مركز اللعب مدافع الجنسية كرواتيا مسيرة الشباب سنوات فريق NK Solin [الإنجليزية] المسي

Nature Reviews Cancer Disiplin ilmuRiset kankerBahasaInggrisDetail publikasiPenerbitNature Publishing Group (Britania Raya)Sejarah penerbitanOkt. 2001-sekarangFaktor dampak37.4 (2014)PengindeksanISSN1474-175X (print)1474-1768 (web)Pranala Journal homepage Nature Reviews Cancer adalah jurnal tinjauan bulanan yang membahas berbagai hal dalam bidang onkologi. Lihat pula Nature lbsGeorg von Holtzbrinck Publishing GroupHoltzbrinckPenerbit S. Fischer Rowohlt Kiepenheuer & W...

Artikel ini membutuhkan rujukan tambahan agar kualitasnya dapat dipastikan. Mohon bantu kami mengembangkan artikel ini dengan cara menambahkan rujukan ke sumber tepercaya. Pernyataan tak bersumber bisa saja dipertentangkan dan dihapus.Cari sumber: Kapurung – berita · surat kabar · buku · cendekiawan · JSTOR Kapurung di Makassar Kapurung (Bahasa Tae': Pugalu) adalah salah satu makanan khas tradisional di Sulawesi Selatan, khususnya masyarakat daerah Luw...

جزء من سلسلة مقالات حولالإسلام حسب البلد الإسلام في إفريقيا أنغولا بنين بوتسوانا بوركينا فاسو بوروندي الكاميرون الرأس الأخضر أفريقيا الوسطى نشاد الجزائر جزر القمر الكونغو الديمقراطية الكونغو ساحل العاج جيبوتي مصر غينيا الاستوائية إريتريا إثيوبيا الغابون غامبيا غانا غي...

One on OneSutradara Kim Ki-duk Produser Kim Ki-duk Kim Soon-mo Ditulis oleh Kim Ki-duk PemeranMa Dong-seok Kim Young-min Lee Yi-kyungSinematograferKim Ki-dukPenyuntingKim Ki-dukPerusahaanproduksiKim Ki-duk FilmTanggal rilis 22 Mei 2014 (2014-05-22) (Korea Selatan) Durasi122 menitNegara Korea Selatan Bahasa Korea One on One (Hangul: 일대일; RR: Il-dae-il) adalah film Korea Selatan tahun 2014 yang disutradarai oleh Kim Ki-duk.[1][2][3] A...

Indian politician This biography of a living person needs additional citations for verification. Please help by adding reliable sources. Contentious material about living persons that is unsourced or poorly sourced must be removed immediately from the article and its talk page, especially if potentially libelous.Find sources: Avinash Rai Khanna – news · newspapers · books · scholar · JSTOR (April 2010) (Learn how and when to remove this template messag...

Временное правительство Амурской области Глава правительства — А. Н. Алексеевский Общая информация Страна Российская республика Российское государство Дата создания 18 сентября 1918 года Предшественник 1) Бюро самоуправлений Амурской области 2) Амурский Совет Народный К�...

WildWorksThe current logoFormerlySmart Bomb InteractiveTypePrivateIndustryGame developerFounded2003FounderKris Johnson Clark Stacey Jeff AmisHeadquartersSalt Lake City, Utah, USProductsAnimal Jam ClassicSnoopy Flying AceWebsitewildworks.com WildWorks (formerly Smart Bomb Interactive) is an American game development studio based in Salt Lake City, Utah. The studio was assembled from game industry veterans, co-founded by Kris Johnson, Clark Stacey, and Jeff Amis. The studio has developed titles...

2018 Filipino filmMLDirected byBenedict Mique Jr.[1]Written byBenedict Mique Jr.Produced byRoselle Lorenzo, Benedict Mique Jr.Starring Eddie Garcia[2] Tony Labrusca[3] CinematographyAnne MonzonEdited byMikael PestanoMusic byPearlsha Abubakar-QuebralDistributed bySolar PicturesRelease date August 3, 2018 (2018-08-03) (Cinemalaya) Running time90 minutes[4]CountryPhilippinesLanguageFilipino ML is a 2018 Philippine independent psychological horro...

Академия просвещения университета им. Витаутаса ВеликогоVytauto Didžiojo universiteto Švietimo akademija Международное название Vytautas Magnus University Education Academy Год основания 1935 Ректор Альгирдас Гайжутис Студенты 12 500 Расположение Литва Юридический адрес Studentų g. 39 Vilnius Сайт svietimas.vdu.lt Медиа...

American neuroscientist, psychologist, economist This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article contains wording that promotes the subject in a subjective manner without imparting real information. Please remove or replace such wording and instead of making proclamations about a subject's importance, use facts and attribution to demonstrate that importance. (August 2023) (Le...


![{\displaystyle [0,1]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/738f7d23bb2d9642bab520020873cccbef49768d)