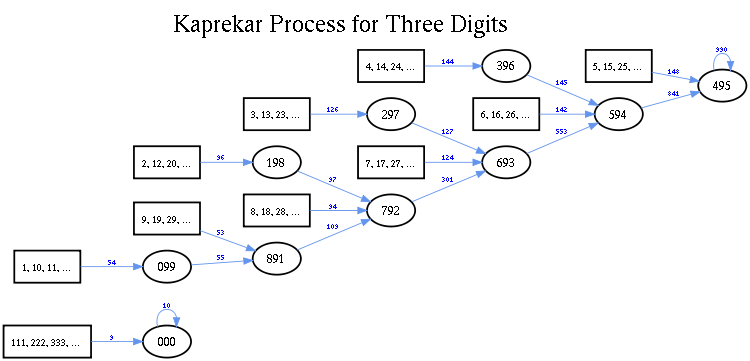
6174
|
Read other articles:

Questa voce sull'argomento centri abitati del Texas è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Blue Moundcity(EN) City of Blue Mound, Texas LocalizzazioneStato Stati Uniti Stato federato Texas ConteaTarrant TerritorioCoordinate32°51′18″N 97°20′17″W / 32.855°N 97.338056°W32.855; -97.338056 (Blue Mound)Coordinate: 32°51′18″N 97°20′17″W / 32.855°N 97.338056°W32.855; -97.338056 (Bl...

Plat dua fabel J. J. Grandville dengan tema serupa dalam fabel La Fontaine, 1838 Merpati dan Semut (bahasa Inggris: The Dove and the Ant) adalah sebuah cerita tentang balasan dari perilaku kesetiakawanan. Tercantum dalam Fabel Aesop, kisah tersebut bernomor 235 dalam Perry Index.[1] Fabel Terdapat sedikit ragam dalam fabel tersebut sejak cerita tersebut pertama kali dicatat dalam sumber-sumber Yunani. Seekor semut yang jatuh ke sungai dan seekor merpati yang memberikan penyelamata...

District of Brunei District in BruneiBelait District Daerah Belait (Malay)دأيره بلأيت (Jawi)District Clockwise from top left: Seria, Labi, Liang, Kuala BelaitCountryBruneiAdministrative townKuala BelaitArea[1] • Total2,727 km2 (1,053 sq mi)Population (2016)[2] • Total69,062 • Estimate (2020)[2]74,800 • Density25/km2 (66/sq mi)Time zoneUTC+8 (BNT)PostcodeKArea code3Websitewww.belait....
Тарт — термін, який має кілька значень. Ця сторінка значень містить посилання на статті про кожне з них.Якщо ви потрапили сюди за внутрішнім посиланням, будь ласка, поверніться та виправте його так, щоб воно вказувало безпосередньо на потрібну статтю.@ пошук посилань саме...

City in Wisconsin, United StatesWest AllisCityLocation of West Allis in Milwaukee County, Wisconsin.West AllisLocation in the United StatesCoordinates: 43°0′29″N 88°1′6″W / 43.00806°N 88.01833°W / 43.00806; -88.01833Country United StatesStateWisconsinCountyMilwaukeeGovernment • TypeMayor-Council • MayorDan DevineArea[1] • Total11.40 sq mi (29.54 km2) • Land11.38 sq mi (29....

2011 Indian filmSeniorsTheatrical release posterDirected byVysakhWritten bySachi-SethuProduced byVaishakh RajanStarringJayaramKunchacko BobanBiju MenonManoj K. JayanCinematographyShaji KumarEdited byMahesh NarayananMusic byJassie GiftAlex PaulAlphons JosephProductioncompanyVaishaka CynymasDistributed byVaishaka ReleaseRelease date 7 May 2011 (2011-05-07) (India) Running time150 minutesCountryIndiaLanguageMalayalamBudget₹3crore[1]Box office₹16 crore[1] Se...

Докладніше: Герой Радянського Союзу У цьому списку представлені, по районах, Герої Радянського Союзу, які народились на території сучасної Одеської області. Список містить інформацію про дату Указу про присвоєння звання, номер медалі «Золота Зірка» рід військ, посаду т

Jean de MenasceDe gauche à droite : Jean de Menasce, Vanessa Bell, Duncan Grant et Eric Siepmann ; photographie d'Ottoline Morrell, 1922.BiographieNaissance 24 décembre 1902AlexandrieDécès 24 novembre 1973 (à 70 ans)ParisNationalité françaiseActivités Orientaliste, théologienAutres informationsA travaillé pour École pratique des hautes étudesOrdre religieux Ordre des Prêcheursmodifier - modifier le code - modifier Wikidata Jean de Menasce, né à Alexandrie le 24 d...

لجنة الالتماسات (بالإنجليزية: Committee on Petitions) والمعروفة اختصاراً بـ PETI هي لجنة دائمة تابعة للبرلمان الأوروبي لإدارة عملية تقديم الالتماسات بما في ذلك بوابة إلكترونية لإنشاء العرائض وقبولها. الحق في تقديم الالتماس هو أحد الحقوق الأساسية للمواطني الاتحاد الأوروبي والمقيمين

Премія «Оскар» за найкращий грим та зачіски Academy Award for Best Makeup and Hairstyling Оскар (94-та церемонія вручення)Засновник(и) Академія кінематографічних мистецтв і наукКраїна СШАВручення Театр «Долбі», Голлівуд, Лос-Анджелес, Каліфорнія, СШАПрезидент Девід РубінОстаннє нагородже

إعصار دينس Category 4 major hurricane (SSHWS/NWS) المعلومات تكون July 4, 2005 تلاشى July 18, 2005 الموسم موسم أعاصيرالمحيط الأطلسي لعام 2005 الفئة hurricane أدنى ضغط جوي 930 المناطق المتأثرة جزر أنتيل ويندوارد جزر الأنتيل الكبرى (particularly كوبا) جنوب شرق الولايات المتحدة (particularly فلوريدا Great Lakes region Canada الخسائر الو

Constituency of the National Assembly of France 6th constituency of Hauts-de-SeineinlineConstituency of the National Assembly of FranceDeputyConstance Le GripREDepartmentHauts-de-SeineCantonsNeuilly-sur-Seine Nord, Neuilly-sur-Seine Sud, Puteaux.Registered voters73,407 Politics of France Political parties Elections Previous Next The 6th constituency of the Hauts-de-Seine is a French legislative constituency in the Hauts-de-Seine département. Description Hauts-de-Seine's 6th constituency lies...

Halaman ini berisi artikel tentang ekonom. Untuk sutradara, lihat Justin Lin. Untuk korban pembunuhan berjuluk Justin Lin, lihat Lin Jun. Untuk politikus, lihat Lin Yi-fu. Justin Yifu LinLahir15 Oktober 1952 (umur 71)Kabupaten Yilan, Provinsi Taiwan, Republik TiongkokKebangsaanTiongkokRRT dari 1979ROC sampai 1979InstitusiBank DuniaUniversitas PekingBidangEkonomi politikAlma materAkademi Militer Republik Tiongkok (Infanteri) Universitas Chengchi Nasional (MA)Universitas Peking (MA) U...

Tsjecho-Slowaakse parlementsverkiezingen 1990 Datum 8 juni en 9 juni 1990[1] Land Tsjechische en Slowaakse Federale Republiek Te verdelen zetels 650 Opkomst 96,7% Opvolging verkiezingen ← 1986 1992 → Portaal Politiek politiek Jan Urban (OF) Ján Budaj (VPN) Ján Čarnogurský (KDH) Josef Bartončík (KDU, ČSL) De Tsjecho-Slowaakse parlementsverkiezingen van 1990 vonden op 5 en 6 juni van dat jaar plaats.[1] Het waren de eerste vrije federale ver...

1976 Manly-Warringah Sea Eagles seasonNSWRFL championsNSWRFL Rank1st1976 recordWins: 18; draws: 0; losses: 7Points scoredFor: 544; against: 300Team informationSecretary Ken ArthursonCoach Frank StantonCaptain Bob FultonStadiumBrookvale OvalTop scorersTries Bob Fulton (21)Goals Graham Eadie (103)Points Graham Eadie (233) < 1975 1977 > The 1976 Manly-Warringah Sea Eagles season was the 30th in the club's history since their entry into the then New South ...

Religion in Mozambique (2020 estimate)[1] Christianity (62%) Islam (19%) No religion (14%) Other (5%) Maputo Cathedral A Muslim worshipper awaits by the door of a mosque Hindu temple in Salamanga Mozambique is a secular state with a majority Christian population and substantial minorities of the adherents of traditional faiths and Islam.[2] Religious demography Inhambane Cathedral Early 2000s In the early 2000s, religious communitie...

Minor league baseball teamMilwaukee Chicks(1944) Milwaukee, Wisconsin Team logo Cap insignia Minor league affiliationsPrevious leaguesAll-American Girls Professional Baseball LeagueMinor league titlesLeague titles 1944Team dataNameBrewettes, SchnittsColorsBlack, red, gold, gray Previous parksBorchert FieldOwner(s)/Operator(s)AAGPBL The Milwaukee Chicks were a women's professional baseball team which played in the All-American Girls Professional Baseball League during...

This article may require cleanup to meet Wikipedia's quality standards. The specific problem is: it reads more like a blog post or an advertisement than a factual article. Please help improve this article if you can. (March 2016) (Learn how and when to remove this template message) Dolac Bronze statue of a market woman at Dolac Market Dolac (pronounced [dɔ̌lat͡s]) is a farmers' market located in Gornji Grad - Medveščak city district of Zagreb, Croatia. Dolac is the most visited a...

Baystate HealthTypeNot-for-profit corporationIndustryHealth careGenreHealth care systemFounded1883 (Originally Springfield Hospital)HeadquartersSpringfield, Massachusetts, United StatesArea servedWestern MassachusettsConnecticutNumber of employees12,000Websitebaystatehealth.org Baystate Health is a not-for-profit integrated health system headquartered in Springfield, Massachusetts, serving Western Massachusetts and the Knowledge Corridor Region of Massachusetts and Connecticut. The system has...

Charles-Louis de Secondat, barone di La Brède e di MontesquieuLa separazione dei poteri (o divisione dei poteri), nel diritto, è uno dei principi giuridici fondamentali dello Stato di diritto e della democrazia liberale. Consiste nell'individuazione di tre funzioni pubbliche principali nell'ambito della sovranità dello Stato (legislazione, amministrazione e giurisdizione) e nell'attribuzione delle stesse a tre distinti poteri dello stato, intesi come organi o complessi di organi dello Stat...