Álxebra multilinear
|
Read other articles:

Public university in Florence, Italy This article includes a list of general references, but it lacks sufficient corresponding inline citations. Please help to improve this article by introducing more precise citations. (April 2013) (Learn how and when to remove this template message) University of FlorenceUniversità degli Studi di FirenzeLatin: Florentina Studiorum UniversitasTypePublicEstablished1321; 702 years ago (1321)RectorAlessandra Petrucci[1]Administrative ...

Sporting event delegationRomania at the1968 Summer OlympicsIOC codeROU(RUM used at these Games)NOCRomanian Olympic and Sports CommitteeWebsitewww.cosr.ro (in Romanian, English, and French)in Mexico CityCompetitors82 in 9 sportsFlag bearerAurel VermescuMedalsRanked 12th Gold 4 Silver 6 Bronze 5 Total 15 Summer Olympics appearances (overview)19001904–1920192419281932193619481952195619601964196819721976198019841988199219962000200420082012201620202024 Romania competed at the 1968 Summ...

American Water Tipo negocio, empresa y empresa de capital abiertoISIN US0304201033Industria empresa de servicios públicosForma legal sociedad por accionesFundación 1886, 1914 y 1947Sede central Camden (Estados Unidos)Productos aguaCoordenadas 39°56′56″N 75°07′51″O / 39.948798, -75.130792Sitio web www.amwater.com[editar datos en Wikidata] American Water (NYSE: AWK) es una empresa de servicios básicos que opera en Estados Unidos y Canadá. Fue fundada en 1...

«Сокіл ІІІ» (Станиславів) Повна назва Засновано 1933 Розформовано 1939 Населений пункт Станиславів, Польща Стадіон Міської каси ощадності Ліга Чемпіонат Українського Спортового Союзу Домашня Виїзна «Со́кіл ІІІ» Станиславі́в (пол. Sokił III Stanisławów) — футбольний клуб, засно�...
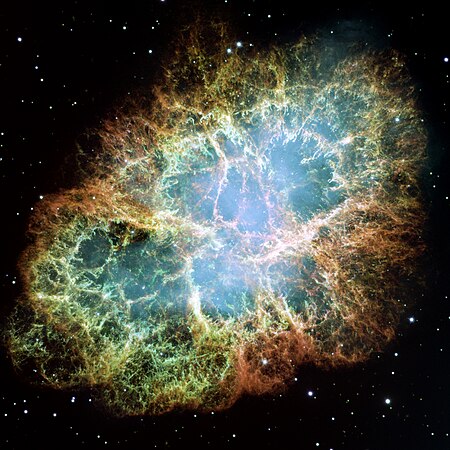
8019 КарачкінаВідкриттяВідкривач Лутц Шмадель,Ф. БернґенМісце відкриття Обсерваторія Карла ШварцшильдаДата відкриття 14 жовтня 1990ПозначенняНазвана на честь Карачкіна Людмила ГеоргіївнаТимчасові позначення 1990 TH12 1981 JZ4 1993 PD5Категорія малої планети Астероїд головного пояс

Evangelische Pauluskirche Außenansicht (2014) Evangelische Pauluskirche, Portal Evangelische Pauluskirche Gemeindesaal-Umbau (Sommer 2010) Evangelische Pauluskirche Innenansicht (2002) Die Evangelische Pauluskirche ist das Kirchengebäude der zur Superintendentur Wien der Evangelischen Kirche Augsburgischen Bekenntnisses in Österreich gehörenden Evangelischen Pfarrgemeinde A.B. Wien-Landstraße und steht im dritten Wiener Gemeindebezirk Landstraße am Sebastianplatz 4. Inhaltsverzeichnis 1...

Untuk tempat lain yang bernama sama, lihat Kepatihan. KepatihanKelurahanKantor Lurah KepatihanNegara IndonesiaProvinsiJawa TimurKabupatenJemberKecamatanKaliwatesKode Kemendagri35.09.19.1006 Kode BPS3509710006 Luas... km²Jumlah penduduk... jiwaKepadatan... jiwa/km² Kepatihan adalah kelurahan di kecamatan Kaliwates, Jember, Jawa Timur, Indonesia. Pembagian wilayah Kelurahan Kepatihan terdiri dari lingkungan: Kauman Kebon Dalem Kepatihan Sawahan Cantikan Tembaan Pranala luar Pemkabjember....

Diagram hipotesis dokumen abad ke-20 Menurut hipotesis dokumen, Elohis (disingkat E) adalah salah satu dari empat dokumen sumber yang menggarisbawahi Taurat,[1] bersama dengan Yahwis Deuteronomis dan sumber Imamat. Dinamai Elohis karena banyak memakai kata Elohim untuk menyebut Allah Israel. Referensi ^ McDermott, John J., Reading the Pentateuch: a historical introduction (Pauline Press, 2002) p. 21. Books.google.com.au. October 2002. ISBN 978-0-8091-4082-4. Retrieved 2010-10-03. Sumb...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada November 2022. Firliana Purwanti (lahir di Jakarta, tanggal 3 Juli 1977) adalah aktivis kesetaraan gender dan inisiator The O Project yang lahir dari rentannya kedaulatan tubuh perempuan karena mitos dan ketabuan.[1] Firli juga aktif sebagai pembicara dengan...

ساره بيات معلومات شخصية الميلاد 6 أكتوبر 1979 (44 سنة) مشهد، وطهران مواطنة إيران إخوة وأخوات سروين بيات أقرباء رضا قوجان منصب نالت بيات منصب سفيرة الصناعة اليدوية الإيرانية حيث أن سارة بيات تعمل مصممة في صناعة السجاد الإيراني الحياة العملية تعلمت لدى برفيز با�...

Abdul Taleb Zakiعبدالطالب ذکیAbdul Taleb ZakiGovernor of Bamyan ProvinceIn office1994–1998 Personal detailsBorn1960Bamyan Province, AfghanistanDied1998 (aged 37–38)Ghazni Province, AfghanistanNationalityAfghanistaniPolitical partyHezbe WahdatOccupationPolitician, military Abdul Taleb Zaki (Dari: عبدالطالب ذکی) was an ethnic Hazara politician and a jihadist from Afghanistan.[1] Abdul Taleb Zaki, son of Abdul Wahid born in 1960 in Bamiyan Province of...

В Википедии есть статьи о других людях с фамилией Фёлькерзам. Густав Ефимович фон Фелькерзамнем. Gustav Johann Georg von Fölkersahm Дата рождения 1799(1799) Дата смерти 1849(1849) Принадлежность Российская империя Род войск инженерные войска Звание генерал-майор Награды и премии Связи...

2010 South Korean television series The Slave HuntersPosterAlso known asChunoGenreAction Historical DramaDeveloped byKBS DramaWritten byChun Sung-ilDirected byKwak Jung-hwanStarringJang HyukOh Ji-hoLee Da-haeGong Hyung-jinLee Jong-hyukComposerKim Jong Chun (김종천) Choi Chul Ho (최철호)Country of originSouth KoreaOriginal languageKoreanNo. of episodes24ProductionExecutive producerChoi Ji Young KBSProducersKi Min SooKim Shin IlProduction locationSouth KoreaRunning timeWednesdays and Thur...

2017 studio album by Helene FischerHelene FischerStudio album by Helene FischerReleased12 May 2017 (2017-05-12)GenreSchlagerpopLabelPolydorUniversalProducerHelene Fischer (exec.)Thorsten BrötzmannMartin FlyDavid GoldRobin GrubertAndreas HerbigMadizinPatrick Pyke SalmySilverjamAli ZuckowskiHelene Fischer chronology Weihnachten(2015) Helene Fischer(2017) Rausch(2021) Singles from Helene Fischer HerzbebenReleased: 30 June 2017 Nur mit dirReleased: 30 June 2017 AchterbahnR...

Israeli jurist Alfred Witkon (Hebrew: אלפרד ויתקון; February 23, 1910 – May 20, 1984) was an Israeli jurist who served as a judge on the Supreme Court of Israel.[1][2] Biography Witkon was born to a wealthy Jewish family in Berlin, Germany. He studied law at the University of Berlin, University of Bonn, and the University of Freiburg, from which he earned a JD. He immigrated to Mandatory Palestine in 1935. His two brothers also immigrated to Palestine. He settled ...

Bilateral relationsPeruvian–American relations Peru United States Diplomatic missionEmbassy of Peru, Washington, D.C.Embassy of the United States, LimaEnvoyPeruvian Ambassador to the United States Hugo de ZelaAmerican Ambassador to Peru Lisa S. Dougherty Kenna Peru and the United States established relations on May 2, 1826, following Peru's independence from Spain. According to Gallup, Inc. polls, Peruvian public perception of the United States is mixed, with 51% of Peruvians viewing the U....

1997 single by T-Spoon This article is about the 1997 song. For 2011 comedy film, see The Inbetweeners Movie. For cocktail, see Sex on the Beach. Sex on the BeachUK cassette artwork (1997)Single by T-Spoonfrom the album The Hit Collection Released4 July 1997 (1997-07-04)Length3:55Label Alabianca (Netherlands) Control (UK) Songwriter(s) Serge Ramaekers Dominic Sas Remy De Groot Humphrey Mijland Shalamon Shamrock Baskin Producer(s) Serge Ramaekers Dominic Sas T-Spoon singles chro...

Greek politician Nikolaos ChountisMEPΝικόλαος ΧουντήςMember of the European Parliamentfor GreeceIncumbentAssumed office 20 July 2015Preceded byManolis GlezosIn office14 July 2009 – 30 June 2014In office15 April 2004 – 19 July 2004Preceded byAlekos AlavanosAlternate Minister of European AffairsIn office27 January 2015 – 13 July 2015Succeeded bySia AnagnostopoulouMember of the Hellenic Parliament for Athens BIn office25 January 2015 ...

Baronía de Mora Corona de barónPrimer titular Félix de Valón y GramontelConcesión Fernando VII23 de agosto de 1817Actual titular Jesús Javier Franco de Espés y Ureta[editar datos en Wikidata] La baronía de Mora es un título nobiliario español creado el 23 de agosto de 1817 por el rey Fernando VII a favor de Félix de Valón y Gramontel,[1] señor de Mas de Mora y regidor de Barbastro.[2] Barones de Mora Titular Periodo Creación por Fernando VII I Félix de V...

وادي هنوممعلومات عامةالبلد إسرائيل تقع في التقسيم الإداري القدس الإحداثيات 31°46′09″N 35°13′41″E / 31.7692°N 35.2281°E / 31.7692; 35.2281 تعديل - تعديل مصدري - تعديل ويكي بيانات 31°46′09″N 35°13′41″E / 31.76916667°N 35.22805556°E / 31.76916667; 35.22805556 القبور في وادي هنوم وادي هنوم أو وادي أب�...









































