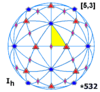
Simetría diédrica en tres dimensiones
| ||||||||||||||||||||||||||||||||
Read other articles:

Coordenadas: 46° 34' N 1° 5' E Saint-Hilaire-sur-Benaize Comuna francesa Localização Saint-Hilaire-sur-BenaizeLocalização de Saint-Hilaire-sur-Benaize na França Coordenadas 46° 34' N 1° 5' E País França Região Centro-Vale do Loire Departamento Indre Características geográficas Área total 32,56 km² População total (2018) [1] 305 hab. Densidade 9,4 hab./km² Código Postal 36370 Código INSEE 36197 Saint-Hilaire-sur...

Turcos de Tracia Occidental Batı Trakya TürkleriΤούρκοι της Δυτικής ΘράκηςDescendencia 50,000–120,000Idioma Turco, griegoReligión Islam sunita[editar datos en Wikidata] La región de Tracia. Tracia Occidental Soldados turcos voluntarios de la República de Tracia Occidental en las calles de Kardzali, 1913 Turcos de Tracia Occidental (en turco: {corrección de cursiva}}, en griego: Τούρκοι της Δυτικής Θράκης, romanizado: Toúrko...

جورج كوهلر (بالألمانية: Georges Jean Franz Köhler) معلومات شخصية الميلاد 17 أبريل 1946[1][2][3] ميونخ الوفاة 1 مارس 1995 (48 سنة) [2] فرايبورغ سبب الوفاة نوبة قلبية[4] مواطنة ألمانيا عضو في الأكاديمية الألمانية للعلوم ليوبولدينا، والمنظمة الأورو

Desert kurrajong Klasifikasi ilmiah Kerajaan: Plantae (tanpa takson): Angiospermae (tanpa takson): Eudikotil (tanpa takson): Rosidae Ordo: Malvales Famili: Malvaceae[a] Genus: Brachychiton Spesies: B. gregorii Nama binomial Brachychiton gregoriiF.Muell., 1862 Brachychiton gregorii, umumnya dikenal sebagai kurrajong gurun, adalah tumbuhan kecil dari genus Brachychiton yang ditemukan di utara, dan barat Australia.[2] Tumbuhan ini tadinya dimasukan ke dalam familia Sterculia...

Mixture of creamed fish or meat with a light egg binding, formed into an egg-like shape For the gesture, see Quenelle (gesture). Quenelle de brochet sauce Nantua A quenelle (French pronunciation: [kə.nɛl]) is a mixture of creamed fish or meat, sometimes combined with breadcrumbs, with a light egg binding, formed into an egg-like shape, and then cooked.[1] The usual preparation is by poaching. Formerly, quenelles were often used as a garnish in haute cuisine. Today, they are ...

Untuk kegunaan lain, lihat Paşcani (disambiguasi). PaşcaniMunisipalitasPaşcaniCountry RumaniaProvinsiIaşiStatusMunisipalitasPemerintahan • Wali kotaGrigore CraciunescuPopulasi (2002) • Total42.172Zona waktuUTC+2 (EET) • Musim panas (DST)UTC+3 (EEST)Situs webhttp://www.pascani.ro/ Paşcani adalah kota yang terletak di Provinsi Iaşi, Rumania, di tepi sungai Siret. Pada tahun 2002, kota ini memiliki jumlah penduduk sebesar 42.172 jiwa. Nama kota...

Cancelled British interceptor aircraft design This article possibly contains original research. Please improve it by verifying the claims made and adding inline citations. Statements consisting only of original research should be removed. (November 2010) (Learn how and when to remove this template message) P.1103 Model of P.1103 on display at the Royal Air Force Museum Cosford Role InterceptorType of aircraft National origin United Kingdom Manufacturer Hawker Aircraft Status Design only Numbe...

Ferntree GullyVictoria—Legislative AssemblyLocation of Ferntree Gully (dark green) in Greater MelbourneStateVictoriaCreated2002Abolished2022MPNick WakelingPartyLiberal PartyElectors41,141 (2018)Area34 km2 (13.1 sq mi)DemographicMetropolitan The electoral district of Ferntree Gully was an electorate of the Victorian Legislative Assembly.[1] It was created in 2002 as a replacement for the abolished electorate of Knox. The district comprised Ferntree Gully and its n...

Belgian politician (1939–2021) Lannoye in 2018 Paul Lannoye (22 June 1939 – 4 December 2021) was a Belgian politician. He was one of the founding members of the Ecolo party and a Member of the European Parliament from 1989 to 2004.[1] Lannoye died on 4 December 2021, at the age of 82, from COVID-19.[2][3] References ^ Home | MEPs | European Parliament. www.europarl.europa.eu. ^ Paul Lannoye (1939-2021), death of a scout. 22 January 2022. ^ Paul Lannoye, m...

背泳ぎ 背泳ぎ(せおよぎ、英: backstroke)は、水泳で、仰向けの姿勢で泳ぐ泳ぎ方である。音読みで「背泳」(はいえい)とも呼ばれるが、競技規則では背泳ぎである。 水泳選手を中心に「バック」という略称が用いられている。 歴史 背泳ぎが行われるようになった時期は明らかでない[1]。古代エジプトのラムセス2世の葬祭殿のレリーフにあるカディシュの戦...

Dutch businessman This biography of a living person needs additional citations for verification. Please help by adding reliable sources. Contentious material about living persons that is unsourced or poorly sourced must be removed immediately from the article and its talk page, especially if potentially libelous.Find sources: Karel Aalbers – news · newspapers · books · scholar · JSTOR (September 2009) (Learn how and when to remove this template message...

An Allied estimate of Japanese units in Borneo at the end of April 1945vteBorneo campaign Agas Semut Tarakan North Borneo Labuan Beaufort Balikpapan Order of battle A map showing the progress of the Borneo campaign This is the complete order of battle of Allied and Japanese forces during the Borneo campaign of 1945. As the campaign was fought in three geographically separate areas and the same air and naval units supported more than one of these battles the order of battle is split into the t...

A Sumerian cylinder seal impression showing the Assyrian Tree of life, the 7-dots glyph (in this case the Pleiades), etc. The 7-dot glyphs (or globes) are first known in Mittanian art (Turkey, or ancient Anatolia),[1] but is possibly older. It appears in the iconography of cylinder seals, and later on reliefs, or other motifs. With origins on cylinder seals, its meanings may come from paleohistory back to the 4th millennium BC, or even further into the 6th to 5th millennium with the o...

特例都道 東京都道430号 新宿停車場前線 路線延長 467 m(実延長) 制定年 1965年 道路の方角 東西 起点 新宿区四谷4丁目交差点 終点 新宿区西新宿1丁目 接続する主な道路(記法) 東京都道4号東京所沢線東京都道5号新宿青梅線 ■テンプレート(■ノート ■使い方) ■PJ道路 単独区間の起点・新宿駅東口交差点 単独区間の終点・新宿大ガード東交差点。左に進むと本�...

This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Munchkin card game – news · newspapers · books · scholar · JSTOR (March 2021) (Learn how and when to remove this template message) Card game spoofing table-top RPG play MunchkinOld box coverPublicationSeptember 2001; 22 years ago (20...

1st Ruler of Saadi Dynasty Abu Abdallah al-Qaimالقائم بأمر الله1st Ruler of Saadi Dynasty Sultan of MoroccoEmir of Sūs(Saadi Sultanate)Reign1509 – 1517Predecessor(State established)SuccessorAhmad al-ArajDiedc. 1517 (aged 30–31)Saadi Sultanate MarrakeshIssueAhmad al-ArajMohammed al-ShaykhEra dates(15th–16th Centuries)DynastyBanū ZaydānReligionSunni Islam Abu Abdallah Muhammad ibn Abd al-Rahman al-Qaim bi-Amr Allah,[1] (Arabic: القائم بأمر الله...

Football tournamentUEFA Youth LeagueOrganising bodyUEFAFounded2013; 10 years ago (2013)RegionEuropeNumber of teams64Qualifier forUnder-20 Intercontinental CupCurrent champions AZ (1st title)Most successful club(s) Barcelona Chelsea(2 titles each)Television broadcastersList of broadcastersWebsiteuefa.com/uefayouthleague 2023–24 UEFA Youth League The UEFA Youth League is an annual club football competition organised by the Union of European Football Associations (UEFA) since...

The Hinckley TimesThe front page of The Hinckley TimesTypeWeekly newspaperFormatTabloidOwner(s)Reach plcFounder(s)Thomas BaxterPublisherReach plcEditorSimon HoldenFoundedJanuary 1889Political alignmentNon-partisanLanguageEnglishHeadquartersLeicesterLeicestershireCityHinckleyCountryEnglandCirculation2,965 (as of 2022)[1]Sister newspapersHinckley Herald (defunct)Leicester MercuryLoughborough EchoNuneaton NewsWebsiteLeicester MercuryMedia of EnglandList of newspapers The Hinckley Tim...

Singaporean-American pornographic actress Annabel ChongChong in 2000BornGrace Quek[1]SingaporeAlma materUniversity of Southern CaliforniaYears active1994–2003Height5 ft 4 in (1.63 m) Grace Quek (Chinese: 郭盈恩; pinyin: Guō Yíng'ēn), known professionally as Annabel Chong,[1] is a Singaporean former pornographic actress who became famous after starring in an adult film that was promoted as the World's Biggest Gang Bang.[1] The fi...

إد أسنر (بالإنجليزية: Ed Asner) معلومات شخصية اسم الولادة (بالإنجليزية: Eddie Asner) الميلاد 15 نوفمبر 1929 كانزاس سيتي، ميزوري الوفاة 29 أغسطس 2021 (91 سنة) طارازانا مواطنة الولايات المتحدة عضو في الاشتراكيون الديمقراطيين في أمريكا الزوجة نانسي سايكس [ل�...