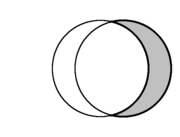
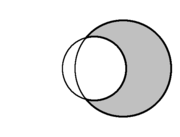
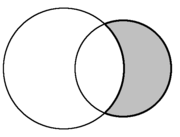
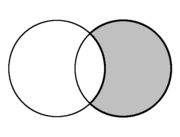
LГәnula (geometrГӯa)
| ||||||||||
Read other articles:

For other uses, see Eadgils (disambiguation). Semi-legendary Swedish king Eadgils pursuing HrГіlfr Kraki on the Fyrisvellir West royal tumulus at Old Uppsala, suggested grave of King EadgilsEadgils, Adils, AГ°ils, Adillus, AГ°Гӯsl at UppsГ¶lum, Athisl, Athislus or Adhel was a semi-legendary king of Sweden, who is estimated to have lived during the 6th century.[1] Beowulf and Old Norse sources present him as the son of Ohthere and as belonging to the ruling Yngling (Scylfing) dynasty. ...

Targa anteriore di una Papamobile con formato in uso da giugno 2002 Le targhe d'immatricolazione della CittГ del Vaticano sono destinate ai veicoli immatricolati nello Stato Vaticano e la loro regolamentazione ГЁ di competenza degli Uffici centrali del Governatorato vaticano[1]. Indice 1 Storia e sistema in uso 2 Formati speciali 2.1 Caratteri rossi 2.2 Targhe provvisorie 3 Note 4 Bibliografia 5 Voci correlate 6 Altri progetti 7 Collegamenti esterni Storia e sistema in uso Targa post...

PP-97 Faisalabad-IConstituencyfor the Provincial Assembly of PunjabRegionChak Jhumra Tehsil of Faisalabad DistrictCurrent constituencyCreated fromPP-51 Faisalabad-I PP-97 Faisalabad-I (ЩҫЫҢ ЩҫЫҢ-97ШҢ ЩҒЫҢШөЩ„ ШўШЁШ§ШҜ-1) is a Constituency of Provincial Assembly of Punjab.[1][2] Election 2013, Azad Ali Tabassum of Pakistan Muslim League (Nawaz) won the seat of MPA from PP вҖ“ 51 FAISALABAD I by collecting 39, 676. Muhammad Ajmal Cheema of Pakistan Tehreek-e-Insaf place second b...

ЩҶЩӮШ·Ш© ШіШұШ¬ЩҠШ© (ШЁШ§Щ„Щ„ЩҲЩҶ Ш§Щ„ШЈШӯЩ…Шұ) Ш№Щ„Щү Ш§Щ„ШұШіЩ… Ш§Щ„ШЁЩҠШ§ЩҶЩҠ Щ„ЩҖ z = x 2 вҲ’y 2 ( Ш§Щ„ЩӮШ·Ш№ Ш§Щ„Щ…ЩғШ§ЩҒШҰ Ш§Щ„ШІШ§ШҰШҜЩҠ) ЩҒЩҠ Ш§Щ„ШұЩҠШ§Ш¶ЩҠШ§ШӘ ШҢ ЩҶЩӮШ·Ш© Ш§Щ„ШіШұШ¬[1] ШЈЩҲ Ш§Щ„ЩҶЩӮШ·Ш© Ш§Щ„ШіШұШ¬ЩҠШ©[2] ШЈЩҲ ЩҶЩӮШ·Ш© Ш§Щ„ШӯШҜ Ш§Щ„ШЈШҜЩҶЩү[3] ЩҮЩҠ ЩҶЩӮШ·Ш© Ш№Щ„Щү ШіШ·Шӯ Ш§Щ„ШұШіЩ… Ш§Щ„ШЁЩҠШ§ЩҶЩҠ Щ„ШҜШ§Щ„Ш© ШӯЩҠШ« ЩҠЩғЩҲЩҶ Ш§Щ„Щ…ЩҠЩ„ (Ш§Щ„Щ…ШҙШӘЩӮШ§ШӘ) ЩҒЩҠ Ш§ШӘШ¬Ш§ЩҮШ§ШӘ Щ…ШӘШ№Ш§Щ…ШҜШ© ЩғЩ„ЩҮШ§ ШөЩҒШұ (ЩҶЩӮШ·Ш© ШӯШұШ¬Ш©)ШҢ ЩҲЩ„ЩғЩҶЩҮШ§ Щ„ЩҠШі...

Chant de Ralliement EspaГұol: Canto de UniГіn InformaciГіn generalHimno de CamerГәn CamerГәnNombre alternativo Г” Cameroun, berceau de nos ancГӘtres Castellano: Oh CamerГәn, cuna de nuestros ancestrosLetra RenГ© Djam AfameSamuel Minkio BambaMoГҜse Nyatte Nko'o, 1978MГәsica RenГ© Djam AfameAdoptado 1957[editar datos en Wikidata] Chant de Ralliement o Canto de UniГіn es el himno nacional de CamerГәn. Se utilizaba desde antes de la independencia y fue oficialmente adoptado en 1957...

Not to be confused with Cherokee County, Alabama. Town in Alabama, United StatesCherokee, AlabamaTownCherokeeLocation of Cherokee in Colbert County, Alabama.Coordinates: 34В°45вҖІ30вҖіN 87В°58вҖІ7вҖіW / 34.75833В°N 87.96861В°W / 34.75833; -87.96861CountryUnited StatesStateAlabamaCountyColbertGovernment вҖў TypeMayorвҖ“council government вҖў MayorMarcus RutlandArea[1] вҖў Total2.22 sq mi (5.74 km2) вҖў Land2...

For related races, see 1914 United States gubernatorial elections. 1914 Alabama gubernatorial election ← 1910 November 3, 1914 1918 → Nominee Charles Henderson John B. Shields E.H Cross Party Democratic Republican Populist Popular vote 61,307 11,773 3,611 Percentage 78.72% 15.11% 4.64% County results Henderson 40вҖ“50% 50вҖ“60% 60вҖ“70% 70вҖ“80% 80вҖ“90% 90вҖ“100% 100% Shields...

FittjaStasiun Stockholms tunnelbanaKoordinat59В°14вҖІ51вҖіN 17В°51вҖІ39вҖіE / 59.24750В°N 17.86083В°E / 59.24750; 17.86083Koordinat: 59В°14вҖІ51вҖіN 17В°51вҖІ39вҖіE / 59.24750В°N 17.86083В°E / 59.24750; 17.86083PemilikStorstockholms LokaltrafikSejarahDibuka1 Oktober 1972Operasi layanan Stasiun sebelumnya Stockholms tunnelbana Stasiun berikutnya Alby Norsborg Jalur T13Masmo Ropsten Lokasi pada petaSunting kotak info вҖў L ...

Chemical experiment that simulated conditions on the early Earth and tested the origin of life The experiment The MillerвҖ“Urey experiment[1] (or Miller experiment[2]) was a chemistry experiment carried out in 1952 that simulated the conditions thought at the time to be present in the atmosphere of the early, prebiotic Earth, in order to test the hypothesis of the chemical origin of life under those conditions. The experiment used water (H2O), methane (CH4), ammonia (NH3), hyd...

British politician (born 1970) This article is about the MP for Monmouth. For the MP for Haltemprice and Howden, see David Davis (British politician). The Right HonourableDavid TC DaviesMPOfficial portrait, 2022Secretary of State for WalesIncumbentAssumed office 25 October 2022Prime MinisterRishi SunakPreceded byRobert BucklandParliamentary Under-Secretary of State for WalesIn office16 December 2019 вҖ“ 25 October 2022Prime MinisterBoris JohnsonLiz TrussPreceded byKevin FosterSuc...

Peruvian politician, lawyer and former journalist In this Spanish name, the first or paternal surname is Mulder and the second or maternal family name is Bedoya. Mauricio MulderChairman of the Peruvian Aprista Party National Political CommissionIncumbentAssumed office July 8, 2017PresidentAlan GarcГӯaCГ©sar TrellesPreceded byJavier VelГЎsquezThird Vice President of CongressIn officeJuly 26, 2017 вҖ“ July 26, 2018PresidentLuis GalarretaPreceded byLuciana LeГіnSucceeded byY...

2010 video game The topic of this article may not meet Wikipedia's notability guidelines for products and services. Please help to demonstrate the notability of the topic by citing reliable secondary sources that are independent of the topic and provide significant coverage of it beyond a mere trivial mention. If notability cannot be shown, the article is likely to be merged, redirected, or deleted.Find sources: Ribbon Hero вҖ“ news В· newspapers В· books В· scho...

РқРө СҒР»РөРҙСғРөСӮ РҝСғСӮР°СӮСҢ СҒ РӨРҫРҪРҫР»РҫРіРёСҸ. РӣРёРҪРіРІРёСҒСӮРёРәР°РҜР·СӢРәРё РјРёСҖаТРөРҫСҖРөСӮРёСҮРөСҒРәР°СҸ лиРҪРіРІРёСҒСӮРёРәР° РӨРҫРҪРҫР»РҫРіРёСҸ РңРҫСҖС„РҫР»РҫРіРёСҸ РЎРёРҪСӮР°РәСҒРёСҒ РӣРөРәСҒРёРәРҫР»РҫРіРёСҸ РӨСҖазРөРҫР»РҫРіРёСҸ РЎРөРјР°РҪСӮРёРәР° (+ Р»РөРәСҒРёСҮРөСҒРәР°СҸ) РҹСҖагмаСӮРёРәР° РҡРҫРіРҪРёСӮРёРІРҪР°СҸ лиРҪРіРІРёСҒСӮРёРәР° Р“РөРҪРөСҖР°СӮРёРІРҪР°СҸ лиРҪРіРІРёСҒСӮРёРәР° Р”РөСҒРәСҖРёРҝСӮРёРІРҪР°СҸ лиРҪРіРІРёСҒСӮРёРәР° РҗРҪСӮСҖРҫРҝРҫР»РҫР...

Railway Station in West Bengal, India аҰ•аҰІаҰ•аҰҫаҰӨаҰҫKOLKATA( аҰҡаҰҝа§ҺаҰӘа§ҒаҰ° / Chitpur )Express train and Passenger train stationKolkata railway stationGeneral informationLocationChitpur, Kolkata, West BengalIndiaCoordinates22В°36вҖІ05вҖіN 88В°23вҖІ03вҖіE / 22.6012775В°N 88.3841474В°E / 22.6012775; 88.3841474Elevation9 metres (30 ft)Owned byIndian RailwaysOperated byEastern RailwayLine(s)Kolkata Circular linePlatforms5Tracks8ConnectionsTaxi stand, Bus stand...

Museum Situs Kota China di Kelurahan Paya PasirMuseum Situs Kota China merupakan nama sebuah situs arkeologi yang terletak di tepi danau Siombak, Kelurahan Paya Pasir, Kecamatan Medan Marelan, Kota Medan. Sejarah Dari abad ke-12 sampai 14 Masehi, Kota China adalah pelabuhan dagang internasional. Kapal-kapal yang berlabuh berasal dari Persia, Hindia dan Tiongkok. Di Kota China ditemukan keramik dari Persia dan Tiongkok, uang koin dari Sri Lanka dan Tiongkok, dan patung Buddha dari Hindia. Situ...

ЩҮШ°ЩҮ Ш§Щ„Щ…ЩӮШ§Щ„Ш© ЩҠШӘЩҠЩ…Ш© ШҘШ° ШӘШөЩ„ ШҘЩ„ЩҠЩҮШ§ Щ…ЩӮШ§Щ„Ш§ШӘ ШЈШ®ШұЩү ЩӮЩ„ЩҠЩ„Ш© Ш¬ШҜЩӢШ§. ЩҒШ¶Щ„ЩӢШ§ШҢ ШіШ§Ш№ШҜ ШЁШҘШ¶Ш§ЩҒШ© ЩҲШөЩ„Ш© ШҘЩ„ЩҠЩҮШ§ ЩҒЩҠ Щ…ЩӮШ§Щ„Ш§ШӘ Щ…ШӘШ№Щ„ЩӮШ© ШЁЩҮШ§. (Щ…Ш§ЩҠЩҲ_2013) Щ…ЩҶШӯЩҶЩү Щ…Ш№Ш§Щ…Щ„ ШөШ№ЩҲШҜ Ш№Ш§ШҜЩҠ. Щ„Ш§ ЩҠЩҶШӘШ¬ Ш§Щ„Ш¬ЩҶШ§Шӯ Ш§Щ„ШӯШ§Щ…Щ„ Ш§Щ„Щ…ЩӮЩҲШі ШөШ№ЩҲШҜЩӢШ§ Ш№ЩҶШҜ ШӘШӯШұЩғЩҮ ШӘШӯШұЩғЩӢШ§ Щ…ЩҲШ§ШІЩҠЩӢШ§ Щ„Щ…ШӯЩҲШұ ЩҠШіЩ…Щү Щ…ШӯЩҲШұ Ш§ЩҶШ№ШҜШ§Щ… Ш§Щ„ШұЩҒШ№ (ШЈЩҲ Ш¬ЩҶШ§Шӯ Ш§ЩҶШ№ШҜШ§Щ… Ш§Щ„ШұЩҒШ№.) Ш№ЩҶШҜ ЩӮЩҠШ§Ші ШІШ§ЩҲЩҠШ© Ш§Щ„Щ…ЩҲШ§Ш¬ЩҮ...

1960, year of the independence of 17 African countries Future Zambians challenge Conservative Party politician Iain Macleod in Northern Rhodesia. 1960 is referred to as the Year of Africa because of a series of events that took place during the yearвҖ”mainly the independence of seventeen African nationsвҖ”that highlighted the growing Pan-African sentiments in the continent. The year brought about the culmination of African independence movements and the subsequent emergence of Africa as a maj...

This biography of a living person needs additional citations for verification. Please help by adding reliable sources. Contentious material about living persons that is unsourced or poorly sourced must be removed immediately from the article and its talk page, especially if potentially libelous.Find sources: George Irumbayam вҖ“ news В· newspapers В· books В· scholar В· JSTOR (August 2014) (Learn how and when to remove this template message) George Irumbayam...

HГҘkon MjГёen HГҘkon MjГёen in gara a Oppdal del 1969 NazionalitГ Norvegia Sci alpino SpecialitГ Slalom gigante, slalom speciale Squadra Oppdal IL Termine carriera 1970 Modifica dati su Wikidata В· Manuale HГҘkon MjГёen (Oppdal, 15 novembre 1944) ГЁ un ex sciatore alpino norvegese. Indice 1 Biografia 2 PalmarГЁs 2.1 Coppa del Mondo 2.2 Campionati norvegesi 3 Note 4 Altri progetti 5 Collegamenti esterni Biografia Attivo alla fine degli anni sessanta, nel 1966 ottenne il 2Вә...

Ш§Щ„ШөЩҒШӯЩҮ ШҜЩү ЩҠШӘЩҠЩ…ЩҮ, ШӯШ§ЩҲЩ„ ШӘШ¶ЩҠЩҒЩ„ЩҮШ§ Щ…ЩӮШ§Щ„Ш§ШӘ Щ…ШӘШ№Щ„ЩӮЩҮ Щ„ЩҠЩҶЩғШ§ШӘ ЩҒЩү ШөЩҒШӯШ§ШӘ ШӘШ§ЩҶЩҠЩҮ Щ…ШӘШ№Щ„ЩӮЩҮ ШЁЩҠЩҮШ§. ШІЩҮШ§ЩҶШ¬ ЪҶЩҠЩҶЩғЩҠЩҲ Щ…Ш№Щ„ЩҲЩ…Ш§ШӘ ШҙШ®ШөЩҠЩҮ Ш§Щ„Щ…ЩҠЩ„Ш§ШҜ ШіЩҶШ© 1936 (Ш§Щ„Ш№Щ…Шұ 87–88 ШіЩҶШ©) ШӘШҙЩҶШ¬ШҜЩҲ Щ…ЩҲШ§Ш·ЩҶЩҮ Ш§Щ„ШөЩҠЩҶ Ш§Щ„ШӯЩҠШ§ЩҮ Ш§Щ„Ш№Щ…Щ„ЩҠЩҮ Ш§Щ„Щ…ШҜШұШіЩҮ Ш§Щ„Ш§Щ… Ш¬Ш§Щ…Ш№Ш© ШӘШіЩҠЩҶШ¬ ЩҮЩҲШ§ Ш§Щ„Щ…ЩҮЩҶЩҮ Щ…ЩҮЩҶШҜШіЩҮ Щ…Ш№Щ…Ш§ШұЩҠЩҮ ШӘШ№ШҜЩҠЩ„ Щ…ШөШҜШұЩҠ - ШӘШ№ШҜЩҠЩ„ ШІЩҮШ§ЩҶШ¬ ЪҶ...