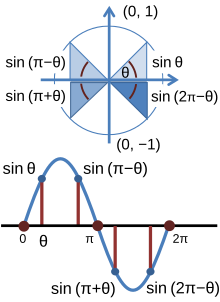
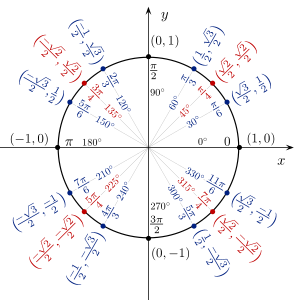
Unit circle
|
Read other articles:

Part of the LGBT rights seriesLegal status ofsame-sex unions Marriage Andorra Argentina Australia Austria Belgium Brazil Canada Chile Colombia Costa Rica Cuba Denmark Ecuador Estonia* Finland France Germany Iceland Ireland Luxembourg Malta Mexico Nepal Netherlands1 New Zealand2 Norway Portugal Slovenia South Africa Spain Sweden Switzerland Taiwan United Kingdom3 United States4 Uruguay Civil unions andregistered partnerships Bolivia Croatia Cyprus Czech Republic Greece Hungary Italy Latvia Lie...

Gereja Santo Silvester PertamaSan Silvestro in Capite (Italia) Sancti Silvestri in Capite (Latin)Bagian depan San Silvestro in Capite, Gereja Nasional di Roma dari Umat Katolik Inggris di Roma, di Piazza San Silvestro.AgamaAfiliasi agamaKatolik RomaEcclesiastical or organizational statusbasilika minor, gereja nasional di Roma dari Britania Raya, titulusKepemimpinanDesmond ConnellLokasiLokasi RomaKoordinat41┬░54ŌĆ▓11.2ŌĆ│N 12┬░28ŌĆ▓50.3ŌĆ│E / 41.903111┬░N 12.480639┬░E /...

ąźąĄčĆčüąŠąĮčüčīą║ąĄ čāčćąĖą╗ąĖčēąĄ ą║čāą╗čīčéčāčĆąĖ ŌĆö ą▓ąĖčēąĖą╣ ąĮą░ą▓čćą░ą╗čīąĮąĖą╣ ąĘą░ą║ą╗ą░ą┤ ąå-ąåąå čĆč¢ą▓ąĮčÅ ą░ą║čĆąĄą┤ąĖčéą░čåč¢čŚ ąĘ ą┐č¢ą┤ą│ąŠč鹊ą▓ą║ąĖ ą╝ąŠą╗ąŠą┤čłąĖčģ čüą┐ąĄčåč¢ą░ą╗č¢čüčéč¢ą▓ ą▓ ą│ą░ą╗čāąĘč¢ ą║čāą╗čīčéčāčĆąĖ. ąŚą░čüąĮąŠą▓ą░ąĮąĄ čā 1944 čĆąŠčåč¢, čÅą║ č鹥čģąĮč¢ą║čāą╝ ą┐ąŠą╗č¢č鹊čüą▓č¢čéąĖ ąĘ ą▓č¢ą┤ą┤č¢ą╗ąĄąĮąĮčÅą╝ąĖ ą▒č¢ą▒ą╗č¢ąŠč鹥čćąĮąŠčŚ čéą░ ą║ą╗čāą▒ąĮąŠčŚ čĆąŠą▒ąŠčéąĖ, ą┐č¢ąĘąĮč¢čłąĄ č鹥čģąĮč¢ą║čāą╝ ą▒čāą▓ ą┐ąĄčĆąĄą╣ą╝ąĄąĮąŠą▓ą░ąĮąĖą╣ ą▓ ą║čāą╗čīčé

Norwegian physician and politician Per Kristian SkulbergPer Kristian Skulberg (born 13 January 1951) is a Norwegian physician and politician. As former State Secretary in the Norwegian Ministry of Environment and in the Norwegian Ministry of Culture he has contributed within the field of heritage interpretation as basis for improved environmental management. Biography On January 13, 1951 Per Kristian Skulberg was born on Skulberg Farm in Spydeberg, Norway to Anton Skulberg and Randi Berg. His...

ąÜčāą▒ąŠą║ ą¤č¢ą▓ąĮč¢čćąĮąŠčŚ ąåčĆą╗ą░ąĮą┤č¢čŚ 2011ŌĆö2012 ą¤ąŠą┤čĆąŠą▒ąĖčåč¢ ąöą░čéą░ ą┐čĆąŠą▓ąĄą┤ąĄąĮąĮčÅ 17 ą▓ąĄčĆąĄčüąĮčÅ 2011 - 5 čéčĆą░ą▓ąĮčÅ 2012 ąÜč¢ą╗čīą║č¢čüčéčī čāčćą░čüąĮąĖą║č¢ą▓ 116 ą¤čĆąĖąĘąŠą▓č¢ ą╝č¢čüčåčÅ ą¦ąĄą╝ą┐č¢ąŠąĮ ąøč¢ąĮčäč¢ą╗ą┤ (42-ą╣ čĆą░ąĘ) ąÆč¢čåąĄč湥ą╝ą┐č¢ąŠąĮ ąÜčĆčāąĘąĄą╣ą┤ąĄčĆčü ąĪčéą░čéąĖčüčéąĖą║ą░ ąŚč¢ą│čĆą░ąĮąŠ ą╝ą░čéčćč¢ą▓ 119 ąŚą░ą▒ąĖč鹊 ą│ąŠą╗č¢ą▓ 493 (4.14 ąĘą░ ą╝ą░čéčć) ŌåÉ 2010ŌĆö2011 2012ŌĆö2013 ŌåÆ ąÜčāą▒ąŠą║ ą¤č¢ą▓ąĮč¢čćąĮąŠčŚ ąåčĆą╗ą...

BBC Hausa language services BBC HausaTypeRadio network and websiteCountryUnited KingdomAvailabilityInternationalOwnerBBCLaunch date13 March 1957Official websitewww.bbc.com/hausa/LanguageHausa BBC Hausa is the Hausa-language service of the BBC World Service meant primarily for Hausa-speaking communities in Nigeria, Ghana, Niger and the rest of Hausa speakers across West Africa. It is part of the BBC's foreign language output of 33 languages, of which five are African languages. The service inc...

Bendera Mongolia dengan wilayah-wilayah historis Wilayah yang diasosiasikan dengan konsep Mongolia Raya Pan-Mongolisme adalah gagasan iredentisme yang ingin menyatukan semua wilayah yang dihuni oleh bangsa Mongol. Wilayah yang diusulkan disebut Mongolia Raya (bahasa Mongol: ąöą░čÅą░čĆ ą£ąŠąĮą│ąŠą╗, Dayaar Mongol) dan mencakup negara Mongolia modern,[1] wilayah Mongolia Dalam dan Dzungaria di Tiongkok, serta Buryatia di Rusia.[2] Kadang-kadang wilayah Tuva, Republik Altai, ...

AnjaliPoster promosional versi pengalihan bahasa HindiSutradara Mani Ratnam Produser G. Venkateswaran Ditulis oleh Mani Ratnam PemeranRaghuvaranRevathiShamiliPrabhuSaranya PonvannanTarunVishnuvardhanPenata musikIlaiyaraajaSinematograferMadhu AmbatPenyuntingB. LeninV. T. VijayanPerusahaanproduksiG. V. FilmsDistributorG. V. FilmsTanggal rilis12 Juli 1990Durasi150 menitNegara India Bahasa Tamil Pendapatankotor$2 juta Anjali adalah sebuah film fitur berbahasa Tamil 1990 yang disutradarai ol...

Gran Premio de Arag├│n de 2014Detalles de carrera 14.┬¬ prueba de 18de la Temporada 2014 del Campeonato. Datos generalesFecha 28 de septiembre de 2014Sede MotorLand Arag├│nCircuitoTipo ylongitud Instalaciones Permanentes5.078 km / 3.155 miMotoGP Pole position Vuelta r├Īpida Marc M├Īrquez1:47.187[1] Jorge Lorenzo1:49.107[1] Podio Jorge Lorenzo Aleix Espargar├│ Cal Crutchlow Moto2 Pole position Vuelta r├Īpida Maverick Vi├▒ales1:54.073[2] Thomas L├╝thi1:54.2...

American actor (1889ŌĆō1960) Walter CatlettCatlett in The Front Page (1931)BornWalter Leland Catlett(1889-02-04)February 4, 1889San Francisco, California, U.S.DiedNovember 14, 1960(1960-11-14) (aged 71)Woodland Hills, California, U.S.Resting placeHoly Cross Cemetery, Culver City, CaliforniaOccupationsActorcomedianYears active1906–1957Spouses Zanetta Watrous (m. 1908; div. 1930) Ruth Verney (divorced)...

Artikel bertopik bandar udara ini perlu dikembangkan agar dapat memenuhi kriteria sebagai entri Wikipedia.Bantulah untuk mengembangkan artikel ini. Jika tidak dikembangkan, artikel ini akan dihapus. Artikel ini tidak memiliki referensi atau sumber tepercaya sehingga isinya tidak bisa dipastikan. Tolong bantu perbaiki artikel ini dengan menambahkan referensi yang layak. Tulisan tanpa sumber dapat dipertanyakan dan dihapus sewaktu-waktu.Cari sumber: Bandar Udara Pulau Panjang ŌĆō...

Private university in Pampanga, Philippines This article relies excessively on references to primary sources. Please improve this article by adding secondary or tertiary sources. Find sources: University of the Assumption ŌĆō news ┬Ę newspapers ┬Ę books ┬Ę scholar ┬Ę JSTOR (February 2013) (Learn how and when to remove this template message) University of the AssumptionPamantasang AssumptionThe oldest Archdiocesan Catholic UniversityFormer namesAssumption Jun...

BMP-1 BMP-1 eks-Irak yang ditangkap oleh pasukan AS di Irak selama Perang Teluk Pertama. Jenis Kendaraan penempur infanteri Negara asal Uni Soviet Sejarah pemakaian Masa penggunaan 1966 ŌĆō sekarang Digunakan oleh Uni Soviet, Rusia, Polandia, Mesir, Mongolia, Siria, RRC, Afghanistan, India, Irak, Jerman, Yunani, dan Swedia. Pada perang Lihat Sejarah penugasan dan Sejarah Pertempuran Sejarah produksi Perancang Pavel Isakov (Biro Rancang ChTZ)[1] Tahun 1961 ŌĆō...

1963 science fiction novel by Clifford D. Simak Way Station First edition coverAuthorClifford D. SimakCover artistRonald FratellCountryUnited StatesLanguageEnglishGenreScience fictionPublisherDoubledayPublication date1963Media typePrint (hardback & paperback)ISBN978-0345284204 Way Station was serialized in Galaxy Science Fiction in 1963 as Here Gather the Stars Way Station is a 1963 science fiction novel by American writer Clifford D. Simak, originally published as Here Gather t...

Chinese geographer and traveller Xu XiakePortrait of Xu XiakeBornXu Hongzu5 January 1587Jiangsu, Ming ChinaDied8 March 1641 (aged 54)Ming ChinaOccupationExplorer Xu XiakeChineseÕŠÉķ£×Õ«óTranscriptionsStandard MandarinHanyu PinyinX├║ Xi├Īk├©WadeŌĆōGilesHs├╝2 Hsia2-k'o4IPA[╔Ģy̌ ╔Ģjǎ.k╩░╔ż̂]Yue: CantoneseYale RomanizationCh├©ui H├ĀahhaakJyutpingCeoi4 Haa4-haak3IPA[ts╩░╔Ąy╦® ha╦É╦®.ha╦Ék╠Ü╦¦] Former residence of Xu Xiake in Jiangyin Tomb of Xu Xiake In this Chinese name,...

Sufi Persian poetry book by Sanai Ghaznavi Traditional printing of Hadiqat al Haqiqa From article series about:Sanai(473ŌĆō545 AH) Works Poetry Diwan Hadiqat al Haqiqa (525 AH) Tariq ut-tahqiq (528 AH) Seir al-Ebad elal-Ma'ad Karnameye Balkh Eshghnameh Aghlnameh Makatib Tahrimatal Ghalam Places Ghazni Balkh Sarakhs Herat Nishapur Mecca Peoples Soltan Mahmud Saboktagin Masoud Ibn Ebrahim GhaznaviŌĆī (492ŌĆō508 AH) Bahram-Shah of Ghazna (511ŌĆō552 AH) Khajeh Asilol Molke Heravi LayKhar Ghavamod...

Category 1 Atlantic hurricane in 1969 Hurricane Martha Hurricane Martha just north of Panama on November 21Meteorological historyFormedNovember 21, 1969DissipatedNovember 25, 1969Category 1 hurricane1-minute sustained (SSHWS/NWS)Highest winds90 mph (150 km/h)Lowest pressure979 mbar (hPa); 28.91 inHgOverall effectsFatalities5 directDamage$30 million (1969 USD)Areas affectedPanama, Costa RicaIBTrACSPart of the 1969 Atlantic hurricane season Hurricane Martha was the...

2012 single by Drake featuring the WeekndCrew LoveSingle by Drake featuring the Weekndfrom the album Take Care ReleasedJuly 30, 2012 (2012-07-30)GenreAlternative R&BLength3:29LabelAspireYoung MoneyCash MoneyRepublicSongwriter(s)Aubrey GrahamAbel TesfayeCarlo MontagneseNoah ShebibAnthony PalmanProducer(s)Noah 40 ShebibIllangeloThe WeekndDrake singles chronology Amen (2012) Crew Love (2012) Enough Said (2012) The Weeknd singles chronology Crew Love(2012) Remember You(...

ž¦┘䞣žĄž¦┘å ž¦┘䞯ž┤┘ćž© The Pale Horse ž║┘䞦┘ü ž¦┘äž▒┘łž¦┘Ŗž® ž¦┘äž░┘Ŗ ┘åž┤ž▒ž¬ ž©┘ć ┘ģ┘å žĘž▒┘ü ž»ž¦ž▒ ž¦┘䞯ž¼┘Ŗž¦┘ä. ┘ģž╣┘ä┘ł┘ģž¦ž¬ ž¦┘ä┘āž¬ž¦ž© ž¦┘ä┘ģžż┘ä┘ü ž¦ž║ž¦ž½ž¦ ┘āž▒┘Ŗž│ž¬┘Ŗ. ž¦┘äž©┘äž» ž¦┘ä┘ģ┘ģ┘ä┘āž® ž¦┘ä┘ģž¬žŁž»ž®. ž¦┘ä┘äž║ž® ž¦┘äžź┘å┘ā┘ä┘Ŗž▓┘Ŗž®. ž¦┘ä┘垦ž┤ž▒ ┘垦ž»┘Ŗ ┘ā┘ł┘ä┘åž▓ ┘ä┘äž¼ž▒ž¦ž”┘ģ. ž¬ž¦ž▒┘Ŗž« ž¦┘ä┘åž┤ž▒ 6 ┘å┘ł┘ü┘ģž©ž▒ 1961. ž¦┘ä┘å┘łž╣ ž¦┘䞯ž»ž©┘Ŗ ž▒┘łž¦┘Ŗž® ž¬žŁ┘é┘Ŗ┘é. ž¦┘䞬┘éž»┘Ŗ┘ģ ž╣ž»ž» ž¦┘䞥┘üžŁž¦ž¬ 256(ž¦┘ä┘åž│ž«ž® ž¦┘䞯žĄ┘ä┘Ŗž®),287(ž¦┘䞬ž▒ž¼┘ģž® ž¦┘äž...

Questa voce sull'argomento hockeisti su ghiaccio svedesi ├© solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Andreas Dackell Nazionalit├Ā Svezia Altezza 178 cm Peso 88 kg Hockey su ghiaccio Termine carriera 2012 Palmar├©s Competizione Ori Argenti Bronzi Olimpiadi 1 0 0 Per maggiori dettagli vedi qui Modifica dati su Wikidata ┬Ę Manuale Andreas Lars Dackell (G├żvle, 29 dicembre 1972) ├© un ex hockeista su ghiaccio svedese. Palmar├©s Olimpi...