Norton's theorem
|
Read other articles:

الخمنة (محلة) تقسيم إداري البلد اليمن المحافظة محافظة إب المديرية مديرية حبيش العزلة عزلة ربع ظلمة القرية قرية القشائي السكان التعداد السكاني 2004 السكان 38 • الذكور 22 • الإناث 16 • عدد الأسر 5 • عدد المساكن 5 معلومات أخرى التوقيت توقيت اليمن (+3 غرينيتش) تعديل

Jaap van de Merwe Van de Merwe in 1971 Algemene informatie Volledige naam Jacob Marinus van de Merwe Geboren 14 september 1924 Overleden 19 februari 1989 Geboorteland Nederland Jaren actief 1959-1989 (en) IMDb-profiel Portaal Cabaret Jaap van de Merwe in Mies en scène in 1968 Jacob Marinus (Jaap) van de Merwe (Rotterdam, 14 september 1924 – Oosterhout, 19 februari 1989) was een Nederlands cabaretier en tekstschrijver. Levensloop Cabaret Hij groeide op in een so...

البسوس بنت منقذ معلومات شخصية الحياة العملية المهنة شاعرة بوابة الأدب تعديل مصدري - تعديل البسوس بنت منقذ التميمية: شاعرة جاهلية يضرب المثل بشؤمها.[1] وهي خالة جساس بن مرة الشيبانيّ.[2] كانت لها (أو لجارها سعد بن شمس الجرمي) ناقة يقال لها سراب، رآها (كليب بن ربيع...

هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (أكتوبر 2019) ويليام جاي أوزوالد معلومات شخصية اسم الولادة (بالإنجليزية: William Joseph Oswald) الميلاد 6 يوليو 1919 كينغ سيتي، مونتيري، كاليفورنيا الوفاة 8 ديسمبر 2005 (86 سن...

إلغاء صداقة: شبكة مظلمةUnfriended: Dark Web (بالإنجليزية) ملصق الفيلممعلومات عامةالصنف الفني فيلم رعب — screenlife (en) المواضيع القائمة ... جريمة حاسوبية[1] — مخترق[1] — انتزاع[1] — ويب مظلم[1] — سادية[1] تاريخ الصدور القائمة ... 9 مارس 2018 (South by Southwest Film Festival (en) )19 يولي�...

Juru gambar teknik sedang bekerja. Juru gambar teknik adalah nama pekerjaan yang bertanggung jawab membuat gambar teknik suatu bangunan atau rancangan lainnya.[1] Seorang juru gambar teknik dapat menggunakan komputer atau sketsa manual dalam menciptakan gambar teknik. Juru gambar teknik biasanya terlibat sejak tahapan konsep desain awal. Jenis-jenis Juru gambar teknik mencakup banyak bidang teknik yang lebih khusus. Klasifikasi Baku Jenis Pekerjaan Indonesia 2002 membagi juru gambar t...

أسامة حسين معلومات شخصية الاسم الكامل أسامة حسين سلطان عبد الله الميلاد 11 أغسطس 1970 (العمر 53 سنة) الكويت الطول 1.81 م (5 قدم 11 1⁄2 بوصة) مركز اللعب مُدَافِع الجنسية الكويت مسيرة الشباب سنوات فريق 1984–1986 النادي العربي المسيرة الاحترافية1 سنوات فريق م. (هـ.) 1987�...

أخبار رائعة النوع كوميديا الموقف بطولة بريغا هيلان[1]، وأندريا مارتن[2]، وآدم كامبل[3]، ونيكول ريتشي[4]، وهوراتيو سانز، وجون مايكل هيغينز[4] البلد الولايات المتحدة لغة العمل الإنجليزية عدد المواسم 1 عدد الحلقات 23 شركة الإنتاج 3 ف�...

Several characters of Gran Torino. From left to right, Walt Kowalski (Clint Eastwood), Thao Vang Lor (Bee Vang), Vu Lor (Brooke Chia Thao), Gran (Chee Thao), and Sue Lor (Ahney Her). The following is a list of significant characters who feature in the 2008 film Gran Torino, directed by Clint Eastwood. Casting calls were held in Fresno, California, Detroit, Michigan, and Saint Paul, Minnesota, from which Eastwood selected ten Hmong American lead actors and supporting actors. Of them, only one ...

This article is about buildings made predominantly out of steel. For other uses, see Steel building (disambiguation). This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Steel building – news · newspapers · books · scholar · JSTOR (July 2010) (Learn how and when to remove this template message) Steel building on...

For the film based on the novel, see Live by Night (film). 2012 Book by Dennis Lehane This article's lead section may be too short to adequately summarize the key points. Please consider expanding the lead to provide an accessible overview of all important aspects of the article. (January 2020) Live by Night AuthorDennis LehaneCountryUnited StatesLanguageEnglishGenreCrime fictionPublisherWilliam Morrow and CompanyPublication dateJanuary 1, 2012 (11 years ago) (2012-01-01)Media&#...

الدوري الهولندي الممتاز تفاصيل الموسم 1976-1977 النسخة 21 البلد هولندا التاريخ بداية:22 أغسطس 1976 المنظم الاتحاد الملكي الهولندي لكرة القدم البطل أياكس أمستردام مباريات ملعوبة 306 عدد المشاركين 18 أهداف مسجلة 862 الدوري الهولندي الممتاز 1975-1976 الدوري الهولند...

No debe confundirse con Ministerio de Hacienda de Chile. Ministerio de Economía, Fomento y Turismo Logotipo del Ministerio.LocalizaciónPaís ChileDivisión noLocalidad Santiago de ChileInformación generalJurisdicción ChileTipo Ministerio de EconomíaSede Av. Libertador Bernardo O'Higgins 1449, Santiago Downtown Torre II, piso 10, 11 y 12, Santiago de ChileOrganizaciónMinistro de Economía, Fomento y Turismo Nicolás Grau VelosoSubsecretaria de Economía y Empresas de Menor Tamaño Javier...

Japanese baseball player Baseball player Hideki AsaiPitcherBorn: (1984-01-01) January 1, 1984 (age 39)Higashisumiyoshi-ku, Osaka, JapanBatted: RightThrew: RightNPB debutSeptember 24, 2003, for the Osaka Kintetsu BuffaloesLast NPB appearanceOctober 5, 2010, for the Yomiuri GiantsNPB statisticsWin–loss record25-33Earned run average4.09Strikeouts378Saves0Holds4 Teams Osaka Kintetsu Buffaloes (2002–2004) Tohoku Rakuten Golden Eagles (2005–2010) Yomiuri Gia...

This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Glendora High School – news · newspapers · books · scholar · JSTOR (February 2021) (Learn how and when to remove this template message) This article needs to be updated. Please help update this article to reflect recent events or newly available information. (A...
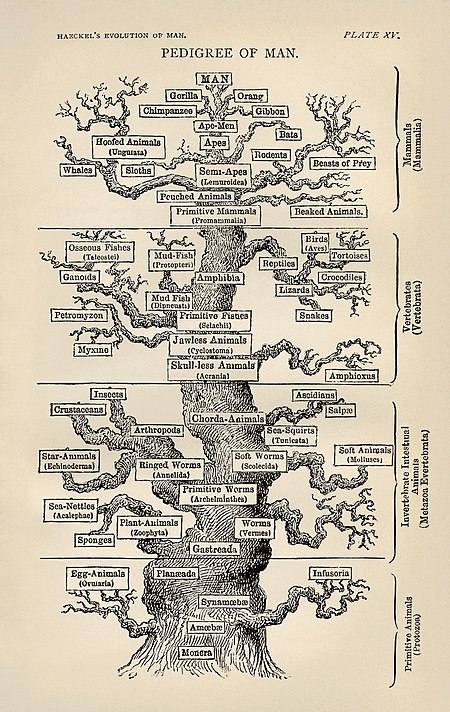
Biologi manusia adalah bidang akademik interdisiplin antara biologi, antropologi fisik, ilmu gizi, dan kedokteran yang terfokus pada manusia. Ilmu ini juga terkait erat dengan biologi primata serta berbagai bidang lain. Istilah humanbiologie (Bahasa Jerman: biologi manusia) pertama kali digunakan oleh Ernst Freiherr von Blomberg dalam bukunya Beiträge zur Studien der Thierverwandlungen (1891). Departemen biologi manusia didirikan pada tahun 1970 di Universitas Stanford. Pranala luar The Prog...

American baseball player (born 1982) Baseball player Colt MortonMorton with the Padres in 2008CatcherBorn: (1982-04-10) April 10, 1982 (age 41)Fort Lauderdale, FloridaBatted: RightThrew: RightMLB debutSeptember 21, 2007, for the San Diego PadresLast MLB appearanceMay 8, 2008, for the San Diego PadresMLB statisticsBatting average.063Home runs0Runs batted in1 Teams San Diego Padres (2007–2008) Kristopher Colt Morton (born April 10, 1982) is an American forme...

WaaijenbergIndustryAutomotiveFounded1966FounderKees WaaijenbergHeadquartersVeenendaal, NetherlandsProductsMicro carsWebsitewww.waaijenberg.com Waaijenberg (pronounced ʋɑjøʏnbɛrɣ) is a Dutch carmaker of micro cars, especially low-speed neighborhood-vehicles for wheelchair users and handicapped drivers. Founded in 1966 by Kees Waaijenberg, the firm is headquartered in Veenendaal, Netherlands. In the 1970s and 1980s, it was popular for importing the Dutch version of Reliant Robin by the Br...

Artist's conception of a mini-Neptune or gas dwarf Planet smaller than Neptune with a gas atmosphere A Mini-Neptune (sometimes known as a gas dwarf or transitional planet) is a planet less massive than Neptune but resembling Neptune in that it has a thick hydrogen-helium atmosphere, probably with deep layers of ice, rock or liquid oceans (made of water, ammonia, a mixture of both, or heavier volatiles).[1] A gas dwarf is a gas planet with a rocky core that has accumulated a thick enve...

PausLusius IIIAwal masa kepausan1 September 1181Akhir masa kepausan25 November 1185PendahuluAleksander IIIPenerusUrbanus IIIInformasi pribadiNama lahirUbaldo AllucingoliLahir1097Lucca, ItaliaMeninggal25 November 1185Verona, Italia Lusius III, nama lahir Ubaldo Allucingoli (Lucca, Italia, 1097 – Verona, Italia, 25 November 1185), adalah Paus Gereja Katolik Roma sejak 1 September 1181 sampai 25 November 1185. lbs Paus Gereja Katolik Daftar paus grafik masa jabatan orang kudus Nama Paus Abdika...



![{\displaystyle {\begin{aligned}I_{\mathrm {no} }&={1\,\mathrm {k} \Omega +1\,\mathrm {k} \Omega \over 1\,\mathrm {k} \Omega +1\,\mathrm {k} \Omega +1\,\mathrm {k} \Omega }\cdot I_{\mathrm {total} }\\[5pt]&=2/3\cdot 5.625\,\mathrm {mA} =3.75\,\mathrm {mA} .\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/6401d8ddf361d0de7567c3581cf46d6937b14d9a)


![{\displaystyle {\begin{aligned}&R_{\rm {th}}=R_{\rm {no}}\\[8pt]&V_{\rm {th}}=I_{\rm {no}}R_{\rm {no}}\\[8pt]&{\frac {V_{\rm {th}}}{R_{\rm {th}}}}=I_{\rm {no}}.\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e34e48d9407799f08f9d9126dea51ea9992b620a)
