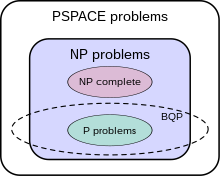
Nondeterministic Turing machine
|
Read other articles:

Paloma perdiz de las Marianas MachoEstado de conservaciónCasi amenazado (UICN 3.1)[1]TaxonomíaReino: AnimaliaFilo: ChordataClase: AvesOrden: ColumbiformesFamilia: ColumbidaeGénero: PampusanaEspecie: P. xanthonura(Temminck, 1823)Sinonimia Gallicolumba xanthonuraAlopecoenas xanthonurus [editar datos en Wikidata] La paloma perdiz de las Marianas o paloma perdiz de Marianas (Pampusana xanthonura) es una especie de ave columbiforme de la familia Columbidae propia de...

Turdoide matorralero Estado de conservaciónPreocupación menor (UICN 3.1)[1]TaxonomíaDominio: EukaryotaReino: AnimaliaFilo: ChordataClase: AvesOrden: PasseriformesFamilia: LeiothrichidaeGénero: TurdoidesEspecie: T. striata(Dumont, 1823)Distribución Sinonimia Turdoides striatusMalacocercus terricolorCossyphus striatusCrateropus canorus [editar datos en Wikidata] Espécimen adulto de la sp. orientalis en Kolkata, West Bengal, India El turdoide matorralero (Turdo...

هذه المقالة عن المملكة النبطية أو مملكة الأنباط. لمعانٍ أخرى، طالع نبطية (توضيح). الأنباط المملكة النبطية القرن الرابع ق.م – 106 م المملكة النبطية في أقصى اتساعها. عاصمة البتراء نظام الحكم ملكية اللغة الرسمية لغة عربية نبطية، والآرامية اللغة آرامية نبطيةع�...

Halaman ini berisi artikel tentang the tertiary education college in Paris. Untuk other uses, lihat Collège de France (disambiguation). Collège de FranceCoat of arms of the Collège de France, given by Louis XIV with letters patent in 1699bahasa Latin: Collegium Franciæ RegiumNama sebelumnyaCollège royal, Collège impérialMotoDocet omnia (Latin)Moto dalam bahasa InggrisTeaches allJenisPublicDidirikan1530; 492 tahun lalu (1530) (royal charter)PendiriFrancis I of FranceAfilia...

2023 South Korean television series AgencyPromotional posterHangul대행사Revised RomanizationDaehaengsaMcCune–ReischauerTaehaengsa GenreWorkplace[1]Written bySong Soo-han[2]Directed byLee Chang-min[2]StarringLee Bo-youngSon Na-eunJo Sung-haHan Joon-wooMusic byKim Hyun-jongAhn So-yeongCountry of originSouth KoreaOriginal languageKoreanNo. of episodes16ProductionExecutive producersKim So-jungShin Seon-joo (CP)Hwang Hyeon-mi (CP)ProducersPark Jin-youngSon Jae-seongRun...

This article may lend undue weight to the opinion of Flyvbjerg et al. Please help improve it by rewriting it in a balanced fashion that contextualizes different points of view. (September 2023) (Learn how and when to remove this template message) Extremely large-scale investment project For a list of megaprojects, see List of megaprojects. For a list of megaprojects specifically related to transportation, see List of transport megaprojects. Itaipu Dam, an example of a 20th-century megaproject...

Political party in the United Kingdom Rock 'n' Roll Loony Party LeaderChris Screwy DriverFounded2000; 23 years ago (2000)Split fromOfficial Monster Raving Loony PartyHeadquartersCannockIdeologySatirePolitics of the United KingdomPolitical partiesElections The Rock 'n' Roll Loony Party was a minor political party in the United Kingdom. Founded in 2000, the group split from the Official Monster Raving Loony Party after the death of Screaming Lord Sutch.[1] Ste...

1995 popular science book Longitude: The True Story of a Lone Genius Who Solved the Greatest Scientific Problem of His Time First edition (US)AuthorDava SobelGenrePopular SciencePublisherWalker & Company (US)Fourth Estate (UK)Publication date1995Pages191ISBN978-0-8027-1529-6OCLC183660066 Longitude: The True Story of a Lone Genius Who Solved the Greatest Scientific Problem of His Time is a 1995 best-selling book by Dava Sobel about John Harrison, an 18th-century clockmaker who created the ...

1979 strategy board game This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Dune board game – news · newspapers · books · scholar · JSTOR (August 2007) (Learn how and when to remove this template message) DuneBox art from the original 1979 release.DesignersBill EberleJack KittredgePeter OlotkaPublishersOri...

Brześć redirects here. For other uses, see Brześć (disambiguation). Brest-Litovsk redirects here. For the treaty, see Treaty of Brest-Litovsk. City in Brest Region, BelarusBrest Брэст (Belarusian)Брест (Russian)CityFrom top, left to right: Fortress church of Saint NicholasFortressOld Bank of Poland buildingSeat of regional authoritiesExaltation of the Holy Cross churchCity History Museum FlagCoat of armsBrestLocation of Brest in BelarusCoordinates: 52°08′05″N 23°...

Bombo FicaBornDaniel Haroldo Fica Roa (1963-09-04) September 4, 1963 (age 60)Purén, ChileNationalityChileanOccupation(s)Humorist, comedian, actorYears active1986–presentPolitical partyCommunist Party of ChileChildren8 Daniel Haroldo Fica Roa, better known by his artistic name Bombo Fica (born 4 September 1963), is a Chilean humorist, comedian and actor. Life and career Daniel Haroldo Fica Roa[1] was born in Purén[2][3] on September 4, 1963.[4] Fic...

Pune Metro's Purple Line terminal metro station in Pimpri-Chinchwad, India PCMC BhavanPune Metro stationGeneral informationLocationMorewadi, Pimpri Colony, Pimpri Chinchwad, Maharashtra 411018Coordinates18°37′46″N 73°48′12″E / 18.6294°N 73.8033°E / 18.6294; 73.8033Owned byMaharashtra Metro Rail Corporation Limited (MAHA-METRO)Operated byPune MetroLine(s)Purple LinePlatformsSide platformPlatform-1 → Civil CourtPlatform-2 → Train Terminates HereTracks2Con...

Indian actress and producer Shweta Shindeat the premiere of Deool BandBornSatara, Maharashtra, IndiaNationalityIndianOccupationsActressModelProducerYears active1998–presentSpouse Sandeep Bhansali (m. 2007)[1]Websiteshwetashindeofficial.com Shweta Shinde is an Indian actress and producer, known for Lagira Zhala Ji's producer and Doctor Don. She launched Vajra Productions with Film director Sanjay Khambe in 2016.[2][3][4] Per...

The Simpsons and Philosophy: The D'oh! of Homer Book coverAuthorWilliam Irwin, Mark T. Conard, Aeon J. SkobleIllustratorJoan Sommers DesignCountryUnited StatesLanguageEnglishSeriesPopular Culture and Philosophy (Vol. 2)SubjectPhilosophy, The SimpsonsGenreNon-fictionPublisherOpen CourtPublication dateFebruary 28, 2001Pages256ISBN0-8126-9433-3Preceded bySeinfeld and Philosophy: A Book about Everything and Nothing Followed byThe Matrix and Philosophy The Simpsons and Philoso...

British crime drama TV series The Murders at White House Farm redirects here. For the events on which the series is based, see White House Farm murders. White House FarmGenre True crime Drama Written by Kris Mrksa Giula Sandler Directed byPaul WhittingtonStarring Freddie Fox Mark Addy Gemma Whelan Mark Stanley Alexa Davies Cressida Bonas Amanda Burton Nicholas Farrell Stephen Graham Alfie Allen Country of originUnited KingdomOriginal languageEnglishNo. of episodes6ProductionExecutive producer...

Shopping mall in New York, United StatesWilton MallExterior of Wilton MallLocationWilton, New York, United StatesCoordinates43°06′08″N 73°44′15″W / 43.10221°N 73.7375°W / 43.10221; -73.7375Address3065 NY Route 50Opening date1990DeveloperWilmorite PropertiesManagementMacerichOwnerMacerichNo. of stores and services70No. of anchor tenants5Total retail floor area708,000 sq ft (65,800 m2)[1]No. of floors1Public transit access 450, 452Websi...

Traditions within Hinduism centered on one or more gods or goddesses Part of a series onHinduism Hindus History Timeline Origins History Indus Valley Civilisation Historical Vedic religion Dravidian folk religion Śramaṇa Tribal religions in India Traditions Major traditions Shaivism Shaktism Smartism Vaishnavism List Deities Trimurti Brahma Vishnu Shiva Tridevi Saraswati Lakshmi Parvati Other major Devas / Devis Vedic: Agni Ashvins Chandra Indra Prajapati Pushan Rudra Surya Ushas ...

Indoor arena in Washington, D.C. United States historic placeUline Ice Company Plant and Arena ComplexU.S. National Register of Historic Places Uline Arena in 2008Location1132, 1140, and 1146 3rd St. NE, Washington, District of ColumbiaCoordinates38°54′18″N 77°0′11″W / 38.90500°N 77.00306°W / 38.90500; -77.00306Area3.9 acres (1.6 ha)Built1941ArchitectKubitz & Koenig; et al.Architectural styleModern MovementNRHP reference No.07000448&...

Filipino historian This article includes a list of references, related reading, or external links, but its sources remain unclear because it lacks inline citations. Please help to improve this article by introducing more precise citations. (June 2012) (Learn how and when to remove this template message) History of the Filipino People redirects here. For Filipino history, see History of the Philippines. In this Philippine name, the middle name or maternal family name is Andal and the ...

Ski jumping competition 2022–23 FIS Ski Jumping World CupDiscipline Men WomenOverall Halvor Egner Granerud (2) Eva PinkelnigNations Cup Austria (20) Austria (5)Ski flying Stefan Kraft (3) —Stage eventsRaw Air Halvor Egner Granerud Ema KlinecFour Hills Tournament Halvor Egner Granerud —Planica7 Stefan Kraft —Silvester Tournament — Eva PinkelnigCompetitionEdition 44th 12thLocations 19 13Individual 33 27Team 5 1Mixed 1 1Rescheduled 1 – ←2021–222023–24→ The 2022–...