Examples of Markov chains
|
Read other articles:

–í –í–∏–∫–∏–ø–µ–¥–∏–∏ –µ—Å—Ç—å —Å—Ç–∞—Ç—å–∏ –æ –¥—Ä—É–≥–∏—Ö –ª—é–¥—è—Ö —Å —Ç–∞–∫–æ–π —Ñ–∞–º–∏–ª–∏–µ–π, —Å–º. –ê–Ω–¥—Ä–µ–µ–≤; –ê–Ω–¥—Ä–µ–µ–≤, –í–∞–¥–∏–º. –í–∞–¥–∏–º –ö–æ–Ω—Å—Ç–∞–Ω—Ç–∏–Ω–æ–≤–∏—á –ê–Ω–¥—Ä–µ–µ–≤ –î–∞—Ç–∞ —Ä–æ–∂–¥–µ–Ω–∏—è 28 —è–Ω–≤–∞—Ä—è 1927(1927-01-28) –ú–µ—Å—Ç–æ —Ä–æ–∂–¥–µ–Ω–∏—è —Å—Ç. –ù–æ–≤–∞—è –ü–æ–∫—Ä–æ–≤–∫–∞, –û—Å—Ç—Ä–æ–≥–æ–∂—Å–∫–∏–π —É–µ–∑–¥, –í–æ—Ä–æ–Ω–µ–∂—Å–∫–∞—è –≥—É–±–µ—Ä–Ω–∏—è, –Ý–°–§–°–Ý, –°–°–°–Ý[1] –î–∞—Ç–∞ —Å–º–µ—Ä—Ç–∏ 1 –∞–≤–≥—É—Å—Ç–∞ 2020(2020-08-01...

PeachesAlbum mini karya KaiDirilis30 November 2021 (2021-11-30)GenreR&BDance-popBahasaKoreanLabelSMDreamusKronologi Kai Kai(2020) Peaches(2021) Singel dalam album Peaches PeachesDirilis: 30 November 2021 Peaches adalah album mini kedua dari penyanyi Korea Selatan Kai. Album ini dirilis pada tanggal 30 November 2021, oleh SM Entertainment. Album mini ini terdiri dari enam lagu termasuk singel utama yang bernama sama. Album fisik tersedia dalam tiga versi yaitu dua versi photobook ...

Rafer Johnson Información personalNacimiento 18 de agosto de 1934 Hillsboro (Estados Unidos) Fallecimiento 2 de diciembre de 2020 Sherman Oaks (Estados Unidos) Causa de muerte Accidente cerebrovascular Nacionalidad EstadounidenseLengua materna Inglés Características físicasAltura 1,9 m Peso 91 kg FamiliaHijos Jenny Johnson Jordan EducaciónEducado en Universidad de California en Los ÁngelesKingsburg High School Información profesionalOcupación Actor, atleta, baloncestista y d...

?–í—ñ–≤—á–∞—Ä–∏–∫ –±–∞–º–±—É–∫–æ–≤–∏–π –û—Ö–æ—Ä–æ–Ω–Ω–∏–π —Å—Ç–∞—Ç—É—Å –ù–∞–π–º–µ–Ω—à–∏–π —Ä–∏–∑–∏–∫ (–ú–°–û–ü 3.1)[1] –ë—ñ–æ–ª–æ–≥—ñ—á–Ω–∞ –∫–ª–∞—Å–∏—Ñ—ñ–∫–∞—Ü—ñ—è –î–æ–º–µ–Ω: –ï—É–∫–∞—Ä—ñ–æ—Ç–∏ (Eukaryota) –¶–∞—Ä—Å—Ç–≤–æ: –¢–≤–∞—Ä–∏–Ω–∏ (Animalia) –¢–∏–ø: –•–æ—Ä–¥–æ–≤—ñ (Chordata) –ö–ª–∞—Å: –ü—Ç–∞—Ö–∏ (Aves) –Ý—è–¥: –ì–æ—Ä–æ–±—Ü–µ–ø–æ–¥—ñ–±–Ω—ñ (Passeriformes) –Ý–æ–¥–∏–Ω–∞: –í—ñ–≤—á–∞—Ä–∏–∫–æ–≤—ñ (Phylloscopidae) –Ý—ñ–¥: –í—ñ–≤—á–∞—Ä–∏–∫ (Phylloscopus) –í–∏–¥: –í—ñ–≤—á–∞—Ä–∏–∫ –±

¬´–ù—ñ–∫–∞¬ª –ü–æ–≤–Ω–∞ –Ω–∞–∑–≤–∞ –ñ—ñ–Ω–æ—á–∏–π —Ñ—É—Ç–±–æ–ª—å–Ω–∏–π –∫–ª—É–±–ù—ñ–∫–∞ (–ú–∏–∫–æ–ª–∞—ó–≤) –ó–∞—Å–Ω–æ–≤–∞–Ω–æ 2014 –ù–∞—Å–µ–ª–µ–Ω–∏–π –ø—É–Ω–∫—Ç –ú–∏–∫–æ–ª–∞—ó–≤, –£–∫—Ä–∞—ó–Ω–∞ –°—Ç–∞–¥—ñ–æ–Ω –ü–∞—Ä–∫ –ü–µ—Ä–µ–º–æ–≥–∏ –í–º—ñ—â—É—î 5 000 –õ—ñ–≥–∞ –í–∏—â–∞ –ª—ñ–≥–∞ –£–∫—Ä–∞—ó–Ω–∏ 2020/21 –î–æ–º–∞—à–Ω—è –í–∏—ó–∑–Ω–∞ –£ –í—ñ–∫—ñ–ø–µ–¥—ñ—ó —î —Å—Ç–∞—Ç—Ç—ñ –ø—Ä–æ —ñ–Ω—à—ñ –∑–Ω–∞—á–µ–Ω–Ω—è —Ü—å–æ–≥–æ —Ç–µ—Ä–º—ñ–Ω–∞: –ù—ñ–∫–∞ (–∑–Ω–∞—á–µ–Ω–Ω—è). –ñ—ñ–Ω–æ—á–∏–π —Ñ—É—Ç–±–æ–ª—å–Ω–...

?–§—É–ª—ñ–≥–æ –∂–æ–≤—Ç–∏–π –§—É–ª—ñ–≥–æ –∂–æ–≤—Ç–∏–π –Ω–∞ –º–µ—Ä—Ç–≤—ñ–π –¥–µ—Ä–µ–≤–∏–Ω—ñ –ë—ñ–æ–ª–æ–≥—ñ—á–Ω–∞ –∫–ª–∞—Å–∏—Ñ—ñ–∫–∞—Ü—ñ—è –¶–∞—Ä—Å—Ç–≤–æ: –ê–º–µ–±–æ–∑–æ—ó (Amoebozoa) –¢–∏–ø: –ú—ñ–∫—Å–æ–º—ñ—Ü–µ—Ç–∏ (Mycetozoa) –ö–ª–∞—Å: Myxogastria –Ý—è–¥: –§—ñ–∑–∞—Ä–∞–ª—å–Ω—ñ (Physarales) –Ý–æ–¥–∏–Ω–∞: –§—ñ–∑–∞—Ä–æ–≤—ñ (Physaraceae) –Ý—ñ–¥: –§—É–ª—ñ–≥–æ (Fuligo) –í–∏–¥: –§—É–ª—ñ–≥–æ –∂–æ–≤—Ç–∏–π –ë—ñ–Ω–æ–º—ñ–∞–ª—å–Ω–∞ –Ω–∞–∑–≤–∞ Fuligo septica(L.) F.H.Wigg[en] (1780) –°–∏–Ω–æ–Ω—ñ–º–∏[1]...

1996 single by Vince GillPretty Little AdrianaSingle by Vince Gillfrom the album High Lonesome Sound B-sideTell Me LoverReleasedOctober 28, 1996GenreCountryLength3:30LabelMCA NashvilleSongwriter(s)Vince GillProducer(s)Tony BrownVince Gill singles chronology Worlds Apart (1996) Pretty Little Adriana (1996) A Little More Love (1997) Pretty Little Adriana is a song written and recorded by American country music artist Vince Gill. It was released in October 1996 as the third single from the album...

ÿπŸÑŸÖ ÿߟÑÿ±Ÿàÿ®Ÿàÿ™ÿßÿ™ ÿߟџܟÖÿßÿ¶Ÿä (DevRob)ÿå ŸàÿߟÑÿ∞Ÿä ŸäŸèÿ∑ŸÑŸÇ ÿπŸÑŸäŸá ŸÅŸä ÿ®ÿπÿ∂ ÿߟÑÿ£ÿ≠ŸäÿßŸÜ ÿπŸÑŸÖ ÿߟÑÿ±Ÿàÿ®Ÿàÿ™ÿßÿ™ ÿߟџàÿ±ÿßÿ´Ÿä ÿߟџÑÿßÿ¨ŸäŸÜŸäÿå ŸáŸà ŸÖŸÜŸáÿ¨Ÿäÿ© ÿ™ÿ≥ÿ™ÿÆÿØŸÖ ÿ£ŸÅŸÉÿßÿ±Ÿãÿß ŸÖÿ≥ÿ™ÿπÿßÿ±ÿ© ŸÖŸÜ ÿߟџܟÖÿßÿ° ÿߟÑÿπÿµÿ®Ÿä ŸàÿπŸÑŸÖ ÿߟџܟÅÿ≥ ÿߟÑÿ™ŸÜŸÖŸàŸä ŸÑÿ™ÿ∑ŸàŸäÿ± ÿπŸÇŸÑ ÿߟÑÿ±Ÿàÿ®Ÿàÿ™ÿßÿ™ ÿߟџÖÿ≥ÿ™ŸÇŸÑÿ©.[1][2][3] ŸàŸäÿ™ŸÖ ÿߟÑÿ™ÿ±ŸÉŸäÿ≤ ÿπŸÑŸâ ÿߟÑÿ±Ÿàÿ®Ÿàÿ™ÿßÿ™ ÿߟÑÿ£ÿ≠ÿßÿØŸäÿ© ÿ£Ÿà ÿߟџÖÿ™ÿπÿØÿØÿ© ŸÖŸÜ ÿÆŸÑÿßŸÑ ŸÖÿ±ÿßÿ...

ABRIXASOrganisasiDeutsches Zentrum für Luft- und Raumfahrt Model teleskopObservatorium antariksaentitas lampau Panjang115 m (377 ft 4 in) Lebar18 m (59 ft 1 in) Massa470 kg (1.040 pon) [sunting di Wikidata] Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala. A Broadband Imaging X...

ماي ليتل بوني: إكويستريا غيرلس: رينبو روكسMy Little Pony: Equestria Girls - Rainbow Rocksmy little pony belongs to hasbroمعلومات عامةالتصنيف فيلم رسوم متحركة الصنف الفني كوميدي، فنتازياتاريخ الصدور 27 سبتمبر 2014مدة العرض 75 دقيقةاللغة الأصلية الإنجليزيةمأخوذ عن مهرتي الصغيرة: الصداقة سحر البلد كندا والولايا...

A fastener providing an expandable metal anchor Molly fastener A molly or molly bolt (often misspelled moly[1]) is a type of screw fastener that fastens objects to plaster or gypsum board hollow walls by providing an anchor to be lodged inside a hole and expanded once in position. Larger sizes permit reasonably heavy objects, such as shelving, flatscreen-TV mounts or central-heating radiators, to be attached to drywall in locations where there is no stud behind the drywall. For heavy ...

Historic house in New York, United States Not to be confused with Charles P. Noyes Cottage. United States historic placeNoyes CottageU.S. National Register of Historic PlacesU.S. Historic districtContributing property Noyes Cottage, November 2007Show map of New YorkShow map of the United StatesLocation16 Helen St., Saranac Lake, Harrietstown, New York, U.S.Coordinates44°19′37″N 74°7′40″W / 44.32694°N 74.12778°W / 44.32694; -74.12778Arealess than one acreBui...

American racing driver NASCAR driver Dave Mader IIIMader during driver intros at Daytona in 2022.BornDavid George Mader III (1955-06-30) June 30, 1955 (age 68)Maylene, AlabamaAchievements1985, 1986, 1987 & 1988 All-American Challenge Series Champion 1978 Snowball Derby WinnerNASCAR Cup Series career10 races run over 5 yearsBest finish45th (1992)First race1988 Busch 500 (Bristol)Last race1992 Coca-Cola 600 (Charlotte) Wins Top tens Poles 0 0 0 NASCAR Xfinity Series career22 races run ...

Church in Michigan, United StatesSt. Mary, Our Lady of Mount Carmel CathedralSt. Mary's Cathedral in 2018Location in Michigan45°02′01″N 84°40′59″W / 45.0335°N 84.6831°W / 45.0335; -84.6831Location606 N. Ohio Ave.Gaylord, MichiganCountryUnited StatesDenominationRoman Catholic ChurchWebsitewww.stmarycathedral.orgHistoryFounded1884DedicationOur Lady of Mount CarmelConsecratedJune 25, 1976ArchitectureHeritage designationMichigan State Historic Site (1901 church...

U.S. Navy's human resources bureau Bureau of Naval PersonnelCurrent logo of the NPCFounded1862; 161 years ago (1862)CountryUnited StatesAllegiance United States of AmericaBranch United States NavyTypeBureauRoleHuman ResourcesWebsitewww.npc.navy.milCommandersChief of Naval PersonnelVADM Richard J. Cheeseman Jr.Military unit The Bureau of Naval Personnel (BUPERS) in the United States Department of the Navy is similar to the human resources department of a corporation...

Australia padaOlimpiadeKode IOCAUSKONKomite Olimpiade AustraliaSitus webwww.olympics.com.auMedali 152 171 193 Total 516 Penampilan Musim Panas18961900190419081912192019241928193219361948195219561960196419681972197619801984198819921996200020042008201220162020Penampilan Musim Dingin193619481952195619601964196819721976198019841988199219941998200220062010201420182022 Berikut ini adalah daftar pembawa bendera yang mewakili Australia pada Olimpiade.[1] Para pembawa bendera membawa bendera n...

Long-duration mission to the International Space Station ISS Expedition 35Promotional PosterMission typeISS Expedition ExpeditionSpace stationInternational Space StationBegan15 March 2013 (2013-03-15)Ended13 May 2013 (2013-05-14)[1]Arrived aboardSoyuz TMA-07MSoyuz TMA-08MDeparted aboardSoyuz TMA-07MSoyuz TMA-08M CrewCrew size6MembersExpedition 34/35:Chris HadfieldThomas MarshburnRoman RomanenkoExpedition 35/36:Christopher CassidyPavel VinogradovAleksandr Misurkin Expedition ...

VRT NWS logo VRT NWS (voorheen Nieuws+) is de digitale radionieuwszender van de VRT. Het is een actualiteitenzender, te beluisteren met een digitaal radiotoestel (DAB), via internet of via digitale televisie. Via DAB zendt Nieuws+ het recentste nieuwsbulletin van Radio 1 in een lus uit. Als men met een digitaal radiotoestel luistert, geeft het scherm van het DAB-toestel de titels van het snelnieuws weer. Ook breaking news wordt direct uitgezonden op VRT NWS. Eind augustus 2017 wijzigde Nieuws...

Nohkancab Osnovni podaci Država Meksiko Savezna država Quintana Roo Opština Felipe Carrillo Puerto Stanovništvo Stanovništvo (2014.) 16[1] Geografija Koordinate 19°48′48″N 87°59′33″W / 19.81333°N 87.9925°W / 19.81333; -87.9925 Vremenska zona UTC-5 Nadmorska visina 13[1] m NohkancabNohkancab na karti Meksika Nohkancab je naselje u Meksiku, u saveznoj državi Quintana Roo, u opštini Felipe Carrillo Puerto. Prema proceni iz 2014. g...

International cricket tour Pakistan cricket team in England in 2010 Pakistan EnglandDates 29 July – 22 September 2010Captains Salman Butt (Tests)Shahid Afridi (ODIs & T20Is) Andrew Strauss (Tests and ODIs)Paul Collingwood (T20Is)Test seriesResult England won the 4-match series 3–1Most runs Umar Akmal (184)[1] Jonathan Trott (404)[1]Most wickets Mohammad Amir (19)[2] James Anderson (23)[2]Player of the series Mohammad Amir (Pak), Jonath...



















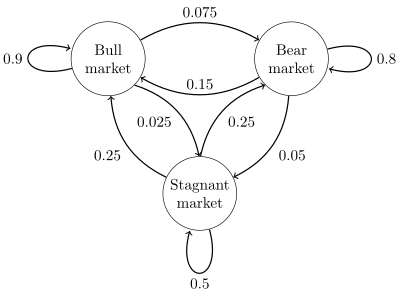


![{\displaystyle {\begin{aligned}x^{(n+3)}&={\begin{bmatrix}0&1&0\end{bmatrix}}{\begin{bmatrix}0.9&0.075&0.025\\0.15&0.8&0.05\\0.25&0.25&0.5\end{bmatrix}}^{3}\\[5pt]&={\begin{bmatrix}0&1&0\end{bmatrix}}{\begin{bmatrix}0.7745&0.17875&0.04675\\0.3575&0.56825&0.07425\\0.4675&0.37125&0.16125\\\end{bmatrix}}\\[5pt]&={\begin{bmatrix}0.3575&0.56825&0.07425\end{bmatrix}}.\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e236058858e3e9fc8c0bd61f7168c236bceedd37)


