Read other articles:

Zoo in Thrissur, Kerala, India Thrissur ZooEntrance of Thrissur Zoo10°31′48″N 76°13′22″E / 10.529965°N 76.2227529°E / 10.529965; 76.2227529Date opened1885[1]LocationThrissur, Kerala, IndiaLand area13.5 acres (5.5 ha)[2]Annual visitors2,000 (Per day) [3]MembershipsCZA[4]Major exhibitsWildlifeWebsitewww.keralamuseumandzoo.org State Museum building inside the Zoo compound War Monument in front of state museum inside the Zoo...

伊東市立宇佐美中学校 2023年2月撮影 北緯35度00分25.0秒 東経139度04分39.0秒 / 北緯35.006944度 東経139.077500度 / 35.006944; 139.077500座標: 北緯35度00分25.0秒 東経139度04分39.0秒 / 北緯35.006944度 東経139.077500度 / 35.006944; 139.077500国公私立の別 公立学校設置者 伊東市設立年月日 1947年4月1日共学・別学 男女共学学期 3学期制学校コード C122210000397 所在地 ...

Marlies Schild Marlies Schild em 2010 Informações pessoais Nome completo Marlies Schild País  Áustria Data de nasc. 31 de maio de 1981 (42 anos) Local de nasc. Estíria, Áustria Altura 1,72 m Jogos Olímpicos Participações 4 Medalhas 3 (0 ouro) Campeonato Mundial Participações 5 Medalhas 7 (2 ouros) Copa do Mundo Temporadas 12 Vitórias 37 Pódios 67 Títulos 0 Por disciplina 5 Quadro de medalhas Jogos Olímpicos Prata Turim 2006 Combinado Prata Vancouver 2010 Slalom P...

Double star in the constellation Cetus NGC 156 SDSS image of NGC 156 (just left of the center) with nearby galaxy NGC 157 Observation dataEpoch J2000      Equinox J2000 Constellation Cetus Right ascension 00h 34m 35.8s[1] Declination −08° 20′ 24″[1] NGC 156 is a double star located in the Cetus constellation. It was discovered on 1882 by Ernst Wilhelm Leberecht Tempel.[2][3] See also New General Catalog...

هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (أبريل 2019) ديفيد أندرو سنكلير   معلومات شخصية الميلاد 26 يونيو 1969 (54 سنة)  سيدني  مواطنة أستراليا  الحياة العملية المدرسة الأم جامعة نيو ساوث ويلز (التخصص:علم الو

SMK Negeri 2 Kota BekasiSekolah Menengah Kejuruan Negeri 2 Kota BekasiInformasiDidirikan27 September 2004JenisNegeriAkreditasiANomor Statistik Sekolah321026503002Nomor Pokok Sekolah Nasional20231741Kepala SekolahB.Agus Wimbadi, M.Pd.Ketua Komite-Jurusan atau peminatanTeknologi, Industri, dan BisnisRentang kelasX - XIIKurikulumKurikulum 2013 RevisiAlamatLokasiJalan Lapangan Bola Rawa Butun, Kelurahan Ciketing Udik, Kecamatan Bantargebang, Kota Bekasi, Jawa Barat, IndonesiaTel./Faks.0...

Josh CooleyCooley di tahun 2019Lahir23 Mei 1979 (umur 44)[1]Berkeley, California, A.SPekerjaanAnimator, sutradara, artis papan cerita, penulis naskah, dan pengisi suaraTahun aktif2003–sekarangTempat kerjaPixar Animation Studios (2003–2020)Dikenal atasToy Story 4Inside OutUpRatatouilleGeorge and A.J.Riley's First Date?Small Fry Josh Cooley adalah seorang animator, sutradara, artis papan cerita, penulis naskah, dan pengisi suara Amerika. Ia dikenal atas karyanya pada film ...

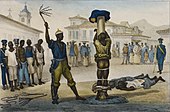
Type of whip For other uses, see Cat o' nine tails (disambiguation). This article's tone or style may not reflect the encyclopedic tone used on Wikipedia. See Wikipedia's guide to writing better articles for suggestions. (April 2023) (Learn how and when to remove this template message) Part of a series onCorporal punishment By place Domestic Judicial School By implementation Amputation Belting Birching Branding Caning Cat o' nine tails Flagellation Foot whipping Knout Paddle Scourge Sjambok S...

Sporting event delegationDominican Republic at the2000 Summer OlympicsFlag of the Dominican RepublicIOC codeDOMNOCDominican Republic Olympic CommitteeWebsitewww.colimdo.org (in Spanish)in SydneyCompetitors13 (11 men and 2 women) in 5 sportsFlag bearer Wanda RijoMedals Gold 0 Silver 0 Bronze 0 Total 0 Summer Olympics appearances (overview)1964196819721976198019841988199219962000200420082012201620202024 The Dominican Republic competed at the 2000 Summer Olympics in Sydney, Australia. ...

This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Cinema of New Zealand – news · newspapers · books · scholar · JSTOR (January 2011) (Learn how and when to remove this template message) Overview of the cinema of New Zealand Cinema of New ZealandNo. of screens411 (2010)[1]Produced feature films (20...

Chan Ho Park is the most recent player to jump from amateur baseball directly to Major League Baseball and become an All-Star. This is a list of baseball players who went directly to the major leagues. They are distinguished as a group by having made their North American professional baseball debut with a Major League Baseball (MLB) franchise without having previously played at the professional level. After their major-league debuts, many of these players appeared in professional leagues othe...

2003 soundtrack album by Various artistsKim PossibleSoundtrack album by Various artistsReleasedJuly 1, 2003Genre Pop dance-pop pop rock R&B LabelWalt Disney The Kim Possible soundtrack is an album released on July 1, 2003 by Walt Disney Records.[1] It contains songs from the Disney Channel series Kim Possible as well as additional songs by contemporary artists. The voice actors for the characters Kim Possible (Christy Carlson Romano), Ron Stoppable (Will Friedle), and Rufu...

A chain of convenience stores operating in the United Kingdom LondisLogo used since 2009A Londis store in Bulwark, ChepstowTypePrivateIndustryRetailFounded1959Area servedUnited KingdomProductsGroceriesParentTesco[1] (via Booker Group[2])Websitewww.londis.co.uk Londis is a symbol group in the United Kingdom with over 2,000 stores nationwide. Tesco owns the brand, following its 2018 purchase of Booker Group.[3][2][1] Although it was formerly a subsidiary,...

ناحية ملح موقع ناحية ملح في محافظة السويداء تقسيم إداري البلد  سوريا[1] المحافظة محافظة السويداء المسؤولون المنطقة منطقة صلخد الناحية ناحية ملح رمز الناحية SY130204 خصائص جغرافية إحداثيات 32°33′21″N 37°04′34″E / 32.555833333333°N 37.076111111111°E / 32.555833333333; 37.076111111111  المسا�...

Jembatan rel batu pasir di atas Hay Street, di samping Belmore Park Papan neon terkenal di Sharpie's Golf House Central adalah sebuah pemukiman urban di sekitar stasiun kereta Central di Sydney, New South Wales, Australia. Terletak di distrik bisnis sentral Sydney dan merupakan bagian dari wilayah pemerintah lokal City of Sydney. Pemukiman ini berada di pinggiran kota terdalam Surry Hills dan Haymarket dan dekat dengan Chinatown. Kode posnya ialah 2000. Sebuah persimpangan bus Sydney terletak...

كالوشا بواليا معلومات شخصية الميلاد 16 أغسطس 1963 (العمر 60 سنة) الطول 180 سنتيمتر  مركز اللعب نصف الجناح  [لغات أخرى]‏  الجنسية زامبيا  المسيرة الاحترافية  سنواتفريقمبارياتأهداف1980–1985 موفوليرا ووندرز -1985–1989 سيركل بروج 95 (30)1989–1994 آيندهوفن 101 (25)1994–1997 أمريكا 8...

County in Georgia, United States County in GeorgiaLincoln CountyCountyLincoln County Courthouse in LincolntonLocation within the U.S. state of GeorgiaGeorgia's location within the U.S.Coordinates: 33°47′N 82°27′W / 33.79°N 82.45°W / 33.79; -82.45Country United StatesState GeorgiaFoundedFebruary 20, 1796; 227 years ago (1796-02-20)Named forBenjamin LincolnSeatLincolntonLargest cityLincolntonArea • Total257 sq mi ...

Railway station in Iida, Nagano Prefecture, Japan Chiyo Station千代駅Chiyo StationGeneral informationLocation732 Chiyo, Iida-shi, Nagano-ken 399-2223JapanCoordinates35°25′44″N 137°49′00″E / 35.4289°N 137.8167°E / 35.4289; 137.8167Elevation380 meters[1]Operated by JR CentralLine(s) Iida LineDistance114.8 km from ToyohashiPlatforms1 side platformOther informationStatusUnstaffedHistoryOpened30 October 1932PassengersFY20151 daily LocationChiyo Statio...

Former diplomatic mission of Russia in USA Former Consulate of Russia in San FranciscoLocationSan FranciscoCoordinates37°47′42″N 122°26′42″W / 37.7951°N 122.4450°W / 37.7951; -122.4450 The Consulate-General of Russia in San Francisco was a diplomatic mission in the 2790 Green Street building in Pacific Heights, San Francisco. It was operated by the Russian Ministry of Foreign Affairs.[1] The building of the former consulate remains government proper...

Not to be confused with Kauffman bracket. In knot theory, the Kauffman polynomial is a 2-variable knot polynomial due to Louis Kauffman.[1] It is initially defined on a link diagram as F ( K ) ( a , z ) = a − w ( K ) L ( K ) {\displaystyle F(K)(a,z)=a^{-w(K)}L(K)\,} , where w ( K ) {\displaystyle w(K)} is the writhe of the link diagram and L ( K ) {\displaystyle L(K)} is a polynomial in a and z defined on link diagrams by the following properties: L ( O ) = 1 {\displaystyle L(O...