Septic equation
|
Read other articles:

Вооружённые силы Республики Хорватиихорв. Oružane snage Republike Hrvatske Годы существования 1991 — настоящее время Страна Хорватия Подчинение Министерство обороны Хорватии Входит в Республику Хорватию Тип вооружённые силы Включает в себя Сухопутные войска ХорватииВоенно-морски

Ця стаття містить перелік посилань, але походження тверджень у ній залишається незрозумілим через практично повну відсутність внутрішньотекстових джерел-виносок. Будь ласка, допоможіть поліпшити цю статтю, перетворивши джерела з переліку посилань на джерела-виноски у...

لمعانٍ أخرى، طالع جون كيلي (توضيح). هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (فبراير 2019) جون كيلي معلومات شخصية تاريخ الميلاد 15 أكتوبر 1930 تاريخ الوفاة 11 أكتوبر 2008 (77 سنة) الجنسية المملكة المتحدة ...

Кванго Водоспад Тезуа на річці Кванго (Ангола) Річка Кванго на мапі басейну Конго 3°22′17″ пд. ш. 17°21′29″ сх. д. / 3.3713888889166777° пд. ш. 17.35805555558377833° сх. д. / -3.3713888889166777; 17.35805555558377833Витік Плато Лунда, центральна АнголаГирло Касаї• координати 3°22′17″...
TychyGedung tipikal di Tychy Lambang kebesaranMotto: Tychy - a good placeNegara PolandiaProvinsi SilesiaCountycity countyDidirikanAbad ke-15Hak kota1951Pemerintahan • Wali kotaAndrzej DziubaLuas • Kota81,64 km2 (3,152 sq mi)Populasi (2009) • Kota129.527 • Kepadatan16/km2 (41/sq mi) • Perkotaan2.746.000 • Metropolitan5.294.000Zona waktuUTC+1 (CET) • Musim panas (DST)UTC+2 (CES...

Este artículo o sección necesita referencias que aparezcan en una publicación acreditada.Este aviso fue puesto el 5 de noviembre de 2009. Diagrama del proceso de Haber-Bosch. En química, el proceso de Haber o proceso de Haber - Bosch es la reacción de nitrógeno e hidrógeno gaseosos para producir amoniaco. La importancia de la reacción radica en la dificultad de producir amoniaco a un nivel industrial. Alrededor del 78,1% del aire que nos rodea es nitrógeno molecular, N2. El elemento ...

This article relies excessively on references to primary sources. Please improve this article by adding secondary or tertiary sources. Find sources: The Big Taboo – news · newspapers · books · scholar · JSTOR (November 2021) (Learn how and when to remove this template message) Big TabooBox cover and game layoutPublishersWinning MovesPlayers4 or more (on teams)Setup time5 minutesPlaying time45-75 minutesChanceLow-MediumAge range12 +SkillsDrawing Languag...

Charles Hepburn-Stuart-Forbes-TrefusisFonctionsMembre de la Chambre des lords10 avril 1866 - 29 mars 1904Membre du 19e Parlement du Royaume-Uni19e Parlement du Royaume-Uni (d)Devon Northern (d)11 juillet 1865 - 10 avril 1866Membre du 18e Parlement du Royaume-Uni18e Parlement du Royaume-Uni (d)Devon Northern (d)28 avril 1859 - 6 juillet 1865Membre du 17e Parlement du Royaume-Uni17e Parlement du Royaume-Uni (d)Devon Northern (d)27 mars 1857 - 23 avril 1859BiographieNaissance 2 mars 1834RomeDéc...

American call center company Expert Global Solutions, Inc. (EGS)TypePrivateIndustryBusiness Process OutsourcingAccounts Receivable ManagementCustomer Relationship ManagementBack Office ServicesFounded2012[1]HeadquartersUnited StatesNumber of locationsOver 100 GloballyRevenueApproximately 2 billion USDOwnerOne Equity PartnersNumber of employees~43,000 (2012)ParentAloricaWebsitewww.egscorp.com The parent company of Expert Global Solutions, after its acquisition by Alorica in June 2016&#...

2002 studio album by Dave DouglasThe InfiniteStudio album by Dave DouglasReleased2002RecordedDecember 16–18, 2001 at Avatar Studios, New York CityGenreJazzLabelRCAProducerDave DouglasDave Douglas chronology Witness(2001) The Infinite(2002) Freak In(2003) The Infinite is the 19th album by American jazz trumpeter Dave Douglas. It was released on the RCA label in 2002 and has performances by Douglas, Chris Potter, Uri Caine, James Genus and Clarence Penn.[1] Reception Professio...

Hiperparatiroidismo secundario Tiroides y paratiroidesEspecialidad EndocrinologíaSinónimos SHPT[editar datos en Wikidata]El hiperparatiroidismo secundario es una afección médica de secreción excesiva de hormona paratiroidea (PTH) por las glándulas paratiroideas en respuesta a hipocalcemia (niveles de calcio en sangre bajos), que da lugar a una hiperplasia de estas glándulas. Este trastorno se ve principalmente en pacientes con insuficiencia renal crónica. A veces se ab...

Chinese government policy initiative China's waste import ban, instated at the end of 2017, prevented foreign inflows of waste products. Starting in early 2018, the government of China, under Operation National Sword, banned the import of several types of waste, including plastics. The ban has greatly affected recycling industries worldwide,[1] as China had been the world's largest importer of waste plastics and processed hard-to-recycle plastics for other countries, especially in the...
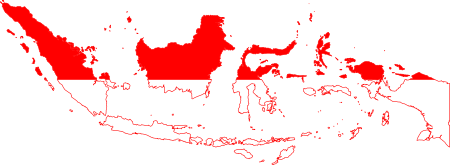
Kawasan Konservasi Perairan Daerah Kabupaten Klungkung (KKPD Kabupaten Klungkung) adalah salah satu kawasan konservasi perairan daerah yang ada di Bali, Indonesia. Dalam pembagian administratif Indonesia, KKPD Kabupaten Klungkung berada di wilayah administratif Kabupaten Klungkung. Nama lainnya adalah Kawasan Konservasi Perairan Nusa Penida Kabupaten Klungkung. Dasar hukum penetapannya adalah Surat Keputusan Menteri Kelautan dan Perikanan Nomor 24 Tahun 2014. Sebelumnya, status KKPD Kabupaten...

Cet article est une ébauche concernant la politique française. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. La soirée du Fouquet's du 6 mai 2007 est une réception organisée au Fouquet's, à Paris, pour célébrer l'élection de Nicolas Sarkozy à la présidence de la République le soir de l'élection présidentielle française de 2007. Cette soirée privée, érigée par ses détracteurs en symbole de l'...

Robert Hay Información personalNacimiento 6 de enero de 1799 Castillo de Duns (Reino Unido) Fallecimiento 4 de noviembre de 1863 (64 años)East Lothian (Reino Unido) Nacionalidad BritánicaFamiliaPadres Robert Hay Janet Erskine Cónyuge Kalitza Psaraki (desde 1828, hasta valor desconocido) Información profesionalOcupación Egiptólogo y arqueólogo [editar datos en Wikidata] Acuarela de Robert Hay (después de 1825) sobre La vaca celestial en la tumba de Seti I. Robert Hay (D...

American aircraft manufacturer between 1906 and 1923 Logo of the company NBL-1 The Wittemann-Lewis Aircraft Company was an American aircraft manufacturer between 1906 and 1923. It was notable for building two large aircraft, the Sundstedt-Hannevig transatlantic seaplane with a 100-foot wingspan and the NBL-1 Barling Bomber a six-engined triplane with a 120-foot wingspan.[1] Early years The company had been formed at Staten Island, New York in 1906 by Charles and Adolph Wittemann as Wi...

Marvel Adventures, formerly Marvel Age, was an imprint of Marvel Comics intended for younger audiences, including small children. Unlike the standard comics published by Marvel, which often take place in story arcs spanning several issues, each Marvel Adventures comic tells a standalone story. In April 2012 it was replaced by all new All Ages line tied to the Marvel Universe block on Disney XD. History The Marvel Age's superheroes, based on the Marvel Comics' main canon's characters. Cover ar...

Questa voce sull'argomento centri abitati dello stato di New York è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Segui i suggerimenti del progetto di riferimento. New Hyde Parkvillage(EN) New Hyde Park, New York New Hyde Park – Veduta LocalizzazioneStato Stati Uniti Stato federato New York ConteaNassau TerritorioCoordinate40°43′55.92″N 73°41′04.92″W / 40.7322°N 73.6847°W40.7322; -73.6847 (New Hyde Park)Coordin...

У этого термина существуют и другие значения, см. Сен-Жерменский мир. Сент-Жерменский мирный договор Дата подписания 10 сентября 1919 Место подписания Сент-Жермен, Франция Вступление в силу 10 сентября 1919 • условия Ратификация Австрией и странами от неё образовавши�...

فاضلة إبراهيم سلطان فاضلة إبراهيم سلطان مع أمها خانزاده سلطان معلومات شخصية الميلاد 8 أغسطس 1941 (83 سنة) باريس، ونويي-سور-سين مواطنة تركيا الزوج سعاد خيري أرغوبلو [لغات أخرى] (1965–1980)جون ألفونس برنارد [لغات أخرى] (1983–2015) الأب محمد ع...





![{\displaystyle x_{k}=\omega _{k}{\sqrt[{7}]{y_{1}}}+\omega _{k}^{6}{\sqrt[{7}]{y_{2}}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/bebaee11b85461c981511ca41b3bbb0df4c1aead)





