Dirichlet's unit theorem
|
Read other articles:

Фестиваль в Будапеште Второй международный фестиваль молодёжи и студентов — фестиваль студенчества и молодёжи планеты проходивший в 1949 году в Будапеште, столице социалистической Венгрии. Делегация Индонезии на II Всемирном фестивале молодёжи и студентов Открывшись...

دالة الشغل في الكيمياء والفيزياء (بالإنجليزية: work function) هي أقل طاقة يحتاجها الألكترون لكي يُنتزع من سطح مادة صلبة . ويعني هنا مجرد انتزاع إلكترون ليصبح بعيدا عن باقي الذرات ولكنه يبقى على مقربة من السطح . تشكل دالة الطاقة هذه إحدى الخواص النوعية للمادة .[1] تتسم المادة ا�...

هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (أبريل 2019) دان ستيوارت معلومات شخصية الميلاد 5 مارس 1961 (62 سنة) لوس أنجلوس مواطنة الولايات المتحدة الحياة العملية المهنة مغني، ومغن مؤلف، وعازف قيثار...

Sampul Have a Nice Day adalah album Roxette, diterbitkan pada Maret 1999. Daftar lagu Crush On You (Per Gessle) Wish I Could Fly (Gessle) 1st single You Can't Put Your Arms Around What's Already Gone (Gessle) Waiting For The Rain (Marie Fredriksson) Anyone (Gessle) 2nd single It Will Take A Long Long Time (Gessle) 7Twenty7 (Gessle) I Was So Lucky (Gessle) Stars (Gessle) 3rd single Salvation (Gessle) 4 single Pay The Price (Gessle) 2nd single in japan Cooper (Gessle) Staring At The Ground (Ges...

Artikel ini perlu diwikifikasi agar memenuhi standar kualitas Wikipedia. Anda dapat memberikan bantuan berupa penambahan pranala dalam, atau dengan merapikan tata letak dari artikel ini. Untuk keterangan lebih lanjut, klik [tampil] di bagian kanan. Mengganti markah HTML dengan markah wiki bila dimungkinkan. Tambahkan pranala wiki. Bila dirasa perlu, buatlah pautan ke artikel wiki lainnya dengan cara menambahkan [[ dan ]] pada kata yang bersangkutan (lihat WP:LINK untuk keterangan lebih lanjut...

和歌山県立串本高等学校 北緯33度27分44秒 東経135度46分45秒 / 北緯33.46225度 東経135.77928度 / 33.46225; 135.77928座標: 北緯33度27分44秒 東経135度46分45秒 / 北緯33.46225度 東経135.77928度 / 33.46225; 135.77928過去の名称 串本町立串本実業学校串本町立串本商業学校和歌山県立串本商業学校国公私立の別 公立学校設置者 和歌山県学区 全県一学区設立年

1520 rebellion in Spain For other revolts by this name, see Comunero (disambiguation). Revolt of the ComunerosExecution of the Comuneros of Castile, by Antonio Gisbert (1860)DateApril 16, 1520 – October 25, 15211LocationCrown of CastileResult Royalist victoryBelligerents Comuneros rebels Royalist CastiliansCommanders and leaders Juan López de Padilla Juan Bravo Francisco Maldonado María PachecoAntonio de Acuña Pedro Girón Charles V, Holy Roman EmperorAdrian of ...
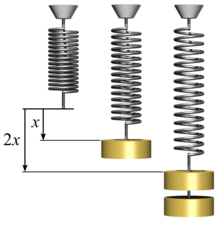
Physical law: force needed to deform a spring scales linearly with distance Hooke's law: the force is proportional to the extension Bourdon tubes are based on Hooke's law. The force created by gas pressure inside the coiled metal tube above unwinds it by an amount proportional to the pressure. The balance wheel at the core of many mechanical clocks and watches depends on Hooke's law. Since the torque generated by the coiled spring is proportional to the angle turned by the wheel, its oscillat...

This article includes a list of general references, but it lacks sufficient corresponding inline citations. Please help to improve this article by introducing more precise citations. (February 2012) (Learn how and when to remove this template message) No. 400 Tactical Helicopter SquadronNo. 400 Squadron RCAF badgeActive5 October 1932 – 7 August 194515 April 1946 – PresentCountry CanadaBranch Royal Canadian Air ForceRoleCH-146 2nd maintenance lineMotto(s)Latin: Percussuri Vigiles(On t...

Sporting event delegationChina at the2022 Asian GamesIOC codeCHNNOCChinese Olympic Committeeexternal link (in Chinese and English)in Hangzhou, China23 September 2023 (2023-09-23) – 8 October 2023 (2023-10-08)Competitors886 (449 men and 437 women)Flag bearers (opening)Yang Liwei (basketball) Qin Haiyang (Swimming)Flag bearer (closing)Xie ZhenyeMedalsRanked 1st Gold 201 Silver 111 Bronze 71 Total 383 Asian Games appearances (overview)1974...

Óxido de sódioAlerta sobre risco à saúde Nome IUPAC Óxido de sódio Identificadores Número CAS 1313-59-3 Propriedades Fórmula molecular Na2O Massa molar 61.979 Aparência sólido de cor branca Densidade 2.27 g/cm3 Ponto de fusão 1132 °C Ponto de ebulição decompõem-se a 1950 °C Solubilidade em água reage para formar hidróxido de sódio Solubilidade em outros solventes insolubilidade Estrutura Estrutura cristalina cúbico Geometria decoordenação 8 Riscos associados Classificaç...

Indonesian military officer and politician (1938–2023) T. B. SilalahiSilalahi in 1993Minister of Public ServantsIn office17 March 1993 – 14 March 1998Preceded bySarwono Kusumaatmadja [id]Succeeded byHartarto Sastrosoenarto [id] Personal detailsBornTiopan Bernhard Silalahi(1938-04-17)17 April 1938Pematangsiantar, Dutch East IndiesDied13 November 2023(2023-11-13) (aged 85)Jakarta, IndonesiaNationalityIndonesianPolitical partyDemocraticEducationPadjadj...

Rudolf Alberth RodjaDeputi Bidang Koordinasi Keamanan dan Ketertiban Masyarakat Kemenko PolhukamPetahanaMulai menjabat 2 Maret 2023Kepala Kepolisian Daerah PapuaMasa jabatan26 April 2019 – 27 September 2019PendahuluMartuani SorminPenggantiPaulus WaterpauwKepala Kepolisian Daerah Papua BaratMasa jabatan20 Juli 2017 – 26 April 2019PendahuluMartuani SorminPenggantiHerry Rudolf NahakKaroprovos Divpropam PolriMasa jabatan14 November 2016 – 20 Juli 2017Pendahulu...

SCIndeks - Serbian Citation IndexProducerCentre for Evaluation in Education and Science (Serbia)LanguagesSerbian, EnglishAccessCostFreeCoverageDisciplinesScience, social science, arts, humanitiesRecord depthCitation indexing, author, topic title, subject keywords, abstract, periodical title, author's address, publication year, full textFormat coverageAcademic journal articlesTemporal coverage1991 to presentGeospatial coverageSerbiaNo. of records80,000 + indexed articles and more than one mill...

Metropolitan area in PhilippinesMetro Baguio BLISTTMetropolitan areaSkyline of Baguio City at nightBaguio and neighboring towns which is regarded as part of Metro Baguio.CountryPhilippinesRegionCordillera Administrative Region(CAR)ProvinceBenguetEstablishedApril 11, 2004Metropolitan CenterBaguioCity/Municipality List BaguioLa TrinidadItogonSablanTubaTublay Government[1] • TypeCouncil • ChairmanBenjamin Magalong(mayor of Baguio) • Co-ChairmanArthur B...

This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Great Astrolabe Reef – news · newspapers · books · scholar · JSTOR (April 2010) (Learn how and when to remove this template message) Great Astrolabe Reef The Great Astrolabe Reef is in Fiji and surrounds the fourth largest island, Kadavu Island, which is approx...

601st Tank Destroyer BattalionActive15 December 1941 – 1945Disbanded1945. Reorganized as 332nd Heavy Tank BattalionCountry United StatesAllegiance United States ArmyPart ofIndependent unitEquipmentM6 FargoM3 Gun Motor CarriageM10 tank destroyerM36 tank destroyerEngagementsWorld War II Torch Avalanche Shingle Dragoon Tunisia Campaign DecorationsPresidential Unit Citation (2)Military unit The 601st Tank Destroyer Battalion was a battalion of the United States Army active during...

This article relies largely or entirely on a single source. Relevant discussion may be found on the talk page. Please help improve this article by introducing citations to additional sources.Find sources: Malawa, Niger – news · newspapers · books · scholar · JSTOR (September 2010) Commune and village in Zinder, NigerMalawa, NigerCommune and villageCountry NigerRegionZinderDepartmentDungassTime zoneUTC+1 (WAT) Malawa, Niger is a village and rural c...

See also: Timeline of the Napoleonic era and First French Empire This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Napoleonic era – news · newspapers · books · scholar · JSTOR (March 2023) (Learn how and when to remove this template message) European history in the 1800s Napoleonic era1799–1815The Emperor Na...

Agujeta asiática En Dayuan, condado de Taoyuan, TaiwánEstado de conservaciónCasi amenazado (UICN 3.1)[1]TaxonomíaReino: AnimaliaFilo: ChordataClase: AvesOrden: CharadriiformesFamilia: ScolopacidaeGénero: LimnodromusEspecie: L. semipalmatus(Blyth, 1848)[editar datos en Wikidata] La agujeta o aguja asiática (Limnodromus semipalmatus)[2][3] es una especie de ave caradriforme de la familia Scolopacidae. Descripción Los adultos tienen patas oscuras...
![{\displaystyle n=[K:\mathbb {Q} ]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/830ec6a7ff9b48ef79d3e0f301ce7c3c881fc061)


















![{\displaystyle \left[1\times \log \left|{\frac {{\sqrt {5}}+1}{2}}\right|,\quad 1\times \log \left|{\frac {-{\sqrt {5}}+1}{2}}\right|\ \right].}](https://wikimedia.org/api/rest_v1/media/math/render/svg/83b9645fc51510239dce3e5e0fc0a960715efb79)


